The SOR method for infinite systems of linear equations (III)

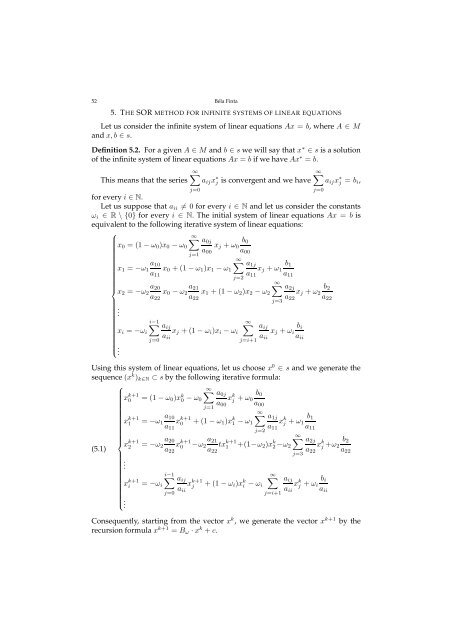
52 Béla Finta5. THE SOR METHOD FOR INFINITE SYSTEMS OF LINEAR EQUATIONSLet us consider the infinite system of linear equations Ax = b, where A ∈ Mand x, b ∈ s.Definition 5.2. For a given A ∈ M and b ∈ s we will say that x ∗ ∈ s is a solutionof the infinite system of linear equations Ax = b if we have Ax ∗ = b.∞∑∞∑This means that the series a ij x ∗ j is convergent and we have a ij x ∗ j = b i ,j=0for every i ∈ N.Let us suppose that a ii ≠0for every i ∈ N and let us consider the constantsω i ∈ R \{0} for every i ∈ N. The initial system of linear equations Ax = b isequivalent to the following iterative system of linear equations:⎧∑∞ a 0j b 0x 0 =(1− ω 0 )x 0 − ω 0 x j + ω 0a 00⎪⎨⎪⎩.j=1x 1 = −ω 1a 10a 11x 0 +(1− ω 1 )x 1 − ω 1a 00∑∞j=2a 1ja 11x j + ω 1b 1x 2 = −ω 2a 20a 22x 0 − ω 2a 21a 22x 1 +(1− ω 2 )x 2 − ω 2.∑i−1a ijx i = −ω i x j +(1− ω i )x i − ω ia iij=0∞ ∑j=i+1a 11∑∞j=3a ija iix j + ω ib ia iij=0a 2ja 22x j + ω 2b 2a 22Using this system of linear equations, let us choose x 0 ∈ s and we generate thesequence (x k ) k∈N ⊂ s by the following iterative formula:⎧∞∑x k+10 =(1− ω 0 )x k 0 − ω a 0j0 x k ja + ω b 0000(5.1)⎪⎨⎪⎩.j=1a 00x k+1 a 10∑∞1 = −ω 1 x k+10 +(1− ω 1 )x k a 1j1 − ω 1 x k b 1j + ω 1a 11 a 11 a 11x k+12 = −ω 2a 20a 22x k+10 −ω 2a 21a 22tx k+1.x k+1i∑i−1a ij= −ω i x k+1ja iij=0j=21 +(1−ω 2 )x k 2 −ω 2 ∞ ∑+(1− ω i )x k i − ω i∞∑j=i+1a ijj=3a iix k j + ω ia 2ja 22x k j +ω 2b 2a 22Consequently, starting from the vector x k , we generate the vector x k+1 by therecursion formula x k+1 = B ω · x k + c.b ia ii