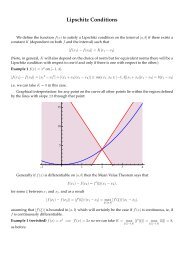
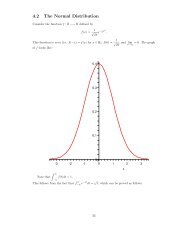
Tanja Lange

D. J. Bernstein & T. Lange http://hyperelliptic.org/tanja/newelliptic – p. 1

Elliptic strikes backD. J. Bernstein & T. Lange http://hyperelliptic.org/tanja/newelliptic – p. 2

To face the challenge, to take thecompetition to a completely new level. . .D. J. Bernstein & T. Lange http://hyperelliptic.org/tanja/newelliptic – p. 3

. . . elliptic has to reconsider its form . . .D. J. Bernstein & T. Lange http://hyperelliptic.org/tanja/newelliptic – p. 4

. . . has to abstract from its Weierstrass formD. J. Bernstein & T. Lange http://hyperelliptic.org/tanja/newelliptic – p. 5

. . . has to undergo severe isomorphictransformations . . .D. J. Bernstein & T. Lange http://hyperelliptic.org/tanja/newelliptic – p. 6

. . . until it finds . . .D. J. Bernstein & T. Lange http://hyperelliptic.org/tanja/newelliptic – p. 7

. . . its true . . .D. J. Bernstein & T. Lange http://hyperelliptic.org/tanja/newelliptic – p. 8

. . . normal form!D. J. Bernstein & T. Lange http://hyperelliptic.org/tanja/newelliptic – p. 9

Long, long ago . . .D. J. Bernstein & T. Lange http://hyperelliptic.org/tanja/newelliptic – p. 10

Euler 1761“ Observationes de Comparatione Arcuum CurvarumIrrectificabilium”1y 2 = 1 − nx21 − x 2 ⇔ x2 + y 2 = 1 + nx 2 y 2 .D. J. Bernstein & T. Lange http://hyperelliptic.org/tanja/newelliptic – p. 11

Euler 1761Euler gives doubling and (special) addition for (a, A) ona 2 + A 2 = 1 − a 2 A 2 .D. J. Bernstein & T. Lange http://hyperelliptic.org/tanja/newelliptic – p. 12

Gauss, posthumouslyGauss gives general addition for arbitrary points on1 = s 2 + c 2 + s 2 c 2 .D. J. Bernstein & T. Lange http://hyperelliptic.org/tanja/newelliptic – p. 13

Ex uno pluraHarold M. Edwards, Bulletinof the AMS, 44, 393–422, 2007x 2 + y 2 = a 2 (1 + x 2 y 2 ), a 5 ≠ adescribes an elliptic curve overfield k of odd characteristic.Every elliptic curve can bewritten in this form – oversome extension field.Ur-elliptic curvex 2 + y 2 = 1 − x 2 y 2needs √ −1 ∈ k transform.Edwards gives addition law for this generalized form,shows equivalence with Weierstrass form, provesaddition law, gives theta parameterization . . .D. J. Bernstein & T. Lange http://hyperelliptic.org/tanja/newelliptic – p. 14

Elliptic geared for cryptoIntroduce further parameter d to cover more curves over kx 2 (+ y 2 = c 2 (1 + dx 2 y 2 ), c, d ≠ 0, dc 4 ≠ 1.)xP y Q + y P x QP + Q =c(1 + dx P x Q y P y Q ) , y P y Q − x P x Q.c(1 − dx P x Q y P y Q )Neutral element is (0, c), this is an affine point!−(x P , y P ) = (−x P , y P ).D. J. Bernstein & T. Lange http://hyperelliptic.org/tanja/newelliptic – p. 15

Elliptic geared for cryptoIntroduce further parameter d to cover more curves over kx 2 (+ y 2 = c 2 (1 + dx 2 y 2 ), c, d ≠ 0, dc 4 ≠ 1.)xP y Q + y P x QP + Q =c(1 + dx P x Q y P y Q ) , y P y Q − x P x Q.c(1 − dx P x Q y P y Q )Neutral element is (0, c), this is an affine point!−(x P , y P ) = (−x P , y P ).[2]P =(xP y P + y P x Pc(1 + dx P x P y P y P ) , y P y P − x P x Pc(1 − dx P x P y P y P )).D. J. Bernstein & T. Lange http://hyperelliptic.org/tanja/newelliptic – p. 15

Elliptic geared for cryptoIntroduce further parameter d to cover more curves over kx 2 (+ y 2 = c 2 (1 + dx 2 y 2 ), c, d ≠ 0, dc 4 ≠ 1.)xP y Q + y P x QP + Q =c(1 + dx P x Q y P y Q ) , y P y Q − x P x Q.c(1 − dx P x Q y P y Q )Neutral element is (0, c), this is an affine point!−(x P , y P ) = (−x P , y P ).[2]P =(xP y P + y P x Pc(1 + dx P x P y P y P ) , y P y P − x P x Pc(1 − dx P x P y P y P )Unified group operations!).D. J. Bernstein & T. Lange http://hyperelliptic.org/tanja/newelliptic – p. 15

Elliptic geared for cryptoIntroduce further parameter d to cover more curves over kx 2 (+ y 2 = c 2 (1 + dx 2 y 2 ), c, d ≠ 0, dc 4 ≠ 1.)xP y Q + y P x QP + Q =c(1 + dx P x Q y P y Q ) , y P y Q − x P x Q.c(1 − dx P x Q y P y Q )A = Z P · Z Q ; B = A 2 ; C = X P · X Q ; D = Y P · Y Q ;E = d · C · D; F = B − E; G = B + E;X P +Q = A · F · ((X P + Y P ) · (X Q + Y Q ) − C − D);Y P +Q = A · G · (D − C); Z P +Q = c · F · G.D. J. Bernstein & T. Lange http://hyperelliptic.org/tanja/newelliptic – p. 15

Elliptic geared for cryptoIntroduce further parameter d to cover more curves over kx 2 (+ y 2 = c 2 (1 + dx 2 y 2 ), c, d ≠ 0, dc 4 ≠ 1.)xP y Q + y P x QP + Q =c(1 + dx P x Q y P y Q ) , y P y Q − x P x Q.c(1 − dx P x Q y P y Q )A = Z P · Z Q ; B = A 2 ; C = X P · X Q ; D = Y P · Y Q ;E = d · C · D; F = B − E; G = B + E;X P +Q = A · F · ((X P + Y P ) · (X Q + Y Q ) − C − D);Y P +Q = A · G · (D − C); Z P +Q = c · F · G.Needs 10M + 1S + 1C + 1D + 7A.At least one of c, d small: x 2 + y 2 = c 2 (1 + dx 2 y 2 ) andx 2 + y 2 = ¯c 2 (1 + ¯dx 2 y 2 ) isomorphic if c 4 d = ¯c 4 ¯d.¯c 4 ¯d = (c 4 d) −1 gives quadratic twist.D. J. Bernstein & T. Lange http://hyperelliptic.org/tanja/newelliptic – p. 15

Unified? Unified!No exceptional cases? What if a denominator is zero?If d is not a square then Edwards addition law iscomplete: For x 2 1 + y2 1 = 1 + dx2 1 y2 1 andx 2 2 + y2 2 = 1 + dx2 2 y2 2 always dx 1x 2 y 1 y 2 ≠ ±1.Outline of proof:If (dx 1 x 2 y 1 y 2 ) 2 = 1 then (x 1 + dx 1 x 2 y 1 y 2 y 1 ) 2 =dx 2 1 y2 1 (x 2 + y 2 ) 2 . Conclude that d is a square. But d is nota square!If d is not a square then there is exactly one point oforder 2 and two of order 4. Otherwise the full 2-torsiongroup is k-rational.Plane curve has 2 singular points at infinity; theirblow-ups are defined over k( √ d) and have order 2.D. J. Bernstein & T. Lange http://hyperelliptic.org/tanja/newelliptic – p. 16

Fastest unified addition-or-doubling formulSystemCost of unified addition-or-doublingProjective 11M+6S+1D; see Brier/Joye ’03Projective if a 4 = −1 13M+3S; see Brier/Joye ’02Jacobi intersection 13M+2S+1D; see Liardet/Smart ’01Jacobi quartic 10M+3S+1D; see Billet/Joye ’01Hessian 12M; see Joye/Quisquater ’01Edwards (c = 1) 10M+1S+1DExactly the same formulae for doubling (nore-arrangement like in Hessian where2(X 1 : Y 1 : Z 1 ) = (Z 1 : X 1 : Y 1 ) + (Y 1 : Z 1 : X 1 );no if-else)No exceptional cases if d is not a square. Formulaecorrect for all affine inputs (incl. (0, c), P + (−P )).D. J. Bernstein & T. Lange http://hyperelliptic.org/tanja/newelliptic – p. 17

Spotlight on the transformationCurve x 2 + y 2 = c 2 (1 + dx 2 y 2 ) in Edwards form is birationallyequivalent to curveE : (1/e)v 2 = u 3 + (4/e − 2)u 2 + uin Montgomery form, where e = 1 − dc 4 .Let (x 1 , y 1 ) + (x 2 , y 2 ) = (x 3 , y 3 ) on Edwards curve. PutP i = ∞ if (x i , y i ) = (0, c);P i = (0, 0) if (x i , y i ) = (0, −c);P i = (u i , v i ) if x i ≠ 0, where u i = (c + y i )/(c − y i ) andv i = 2c(c + y i )/(c − y i )x i .Then P i ∈ E(k) and P 1 + P 2 = P 3 .D. J. Bernstein & T. Lange http://hyperelliptic.org/tanja/newelliptic – p. 18

D. J. Bernstein & T. Lange http://hyperelliptic.org/tanja/newelliptic – p. 19

D. J. Bernstein & T. Lange http://hyperelliptic.org/tanja/newelliptic – p. 20