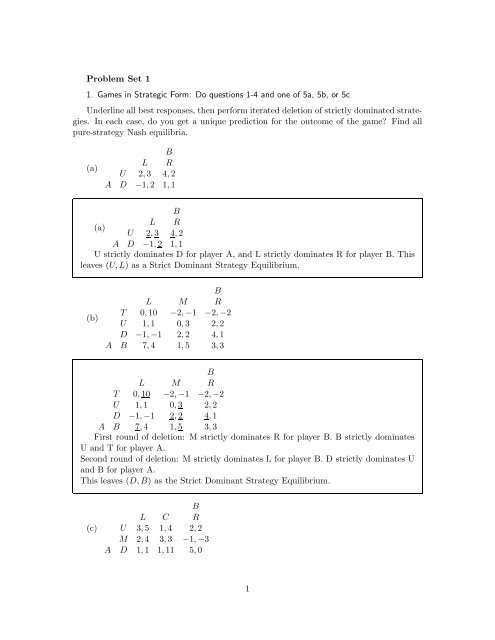
Problem Set 1 1. Games in Strategic Form: Do questions 1-4 and ...

Problem Set 11. Games in Strategic Form: Do questions 1-4 and one of 5a, 5b, or 5cUnderline all best responses, then perform iterated deletion of strictly dominated strategies.In each case, do you get a unique prediction for the outcome of the game? Find allpure-strategy Nash equilibria.(a)BL RU 2,3 4,2A D −1,2 1,1BL R(a)U 2,3 4,2A D −1,2 1,1U strictly dominates D for player A, and L strictly dominates R for player B. Thisleaves (U,L) as a Strict Dominant Strategy Equilibrium.(b)BL M RT 0,10 −2,−1 −2,−2U 1,1 0,3 2,2D −1,−1 2,2 4,1A B 7,4 1,5 3,3BL M RT 0,10 −2,−1 −2,−2U 1,1 0,3 2,2D −1,−1 2,2 4,1A B 7,4 1,5 3,3First round of deletion: M strictly dominates R for player B. B strictly dominatesU and T for player A.Second round of deletion: M strictly dominates L for player B. D strictly dominates Uand B for player A.This leaves (D,B) as the Strict Dominant Strategy Equilibrium.(c)BL C RU 3,5 1,4 2,2M 2,4 3,3 −1,−3A D 1,1 1,11 5,01

BL C RU 3,5 1,4 2,2M 2,4 3,3 −1,−3A D 1,1 1,11 5,0First round: C strictly dominates R for player B. M strictly dominates D for playerA.Second round: L strictly dominates C for player B. U strictly dominates M for playerA.This leaves (U,L) as the Strict Dominant Strategy Equilibrium.(d)BL C O RU 2,2 1,3 6,2 1,1T 4,3 2,2 5,1 2,−3A M 1,9 1,5 −3,2 8,8D 5,8 −1,7 2,2 1,10BL C O RU 2,2 1,3 6,2 1,1T 4,3 2,2 5,1 2,−3A M 1,9 1,5 −3,2 8,8D 5,8 −1,7 2,2 1,10Then C strictly dominates O for the column player. Once O is gone, T strictlydominates U for the row player. Then L strictly dominates C for the column player.Nothing else can be deleted.You can see that no strategy profile is a mutual best response (two underlines), sothere is no pure-strategy Nash equilibrium. This means there isn’t a weak dominantstrategy or strict dominant strategy equilibrium, either.2. Write the following games in strategic form. Find all pure-strategy Nash equilibria, ifthey exist.(a). Two firms that make alcohol spend money trying to outdo one another in advertising.If they both advertise, they share industry profits of 10,000 equally, but if one firmadvertises and the other doesn’t, the advertising firm gets 7,000 and the non-advertiser gets3,000. If they fail to advertise, there are fewer sales but both save on the cost of the adcampaigns, and each makes 6,000.FirmBAdvertise Don ′ tAdvertise 5,5 7,3FirmA Don ′ t 3,7 6,62

The Nash equilibrium is (A,A), since it is a mutual best response: If either playerchanges her behavior, she gets 3 instead of 5, making her worse off. Therefore, no onehas an incentive to change.(b). Twofirmsworkclosely together andhavetodecidewhethertobuyMacorWindowscomputers. If they both buy the same platform, they coordinate well together, and earnprofits of 3 each. If they buy different platforms, they have trouble coordinating, and get apayoff of 1 each.FirmBWindows MacWindows 3,3 1,1FirmA Mac 1,1 3,3Therearetwo pure-strategy Nashequilibria: (W,W) and (M,M). Check: No playercan change her behavior and get a higher pay-off.(c). You and an opponent both have a penny. Secretly, you choose Heads or Tails, thensimultaneously reveal the strategies you picked. If the coins match (both heads or bothtails), you get both pennies. If they are different (one head and one tail), your opponentgets both.ThemH TH 1,−1 −1,1You T −1,1 1,−1There is NO pure-strategy Nash equilibrium. However, you should now see there’sa mixed-strategy Nash equilibrium: Play H and T each with probability 1 2 .(d). Two firms are deciding whether or not to research a new technology. If neitherfirm researches, they both make zero. If both firms research, they get 5 each. If one firmresearches and the other doesn’t, the firm that does the research gets 10 and the firm thatdoesn’t research gets 0.2R DR 5,5 10,01 D 0,10 0,0Both firms have a dominant strategy to research, so the Nash equilibrium is (R,R).3. Two firms can choose any whole dollar price from 0 to 4 to charge for their product,for which they have noproduction costs. Theoverall demand is for thegood is D(p) = 4−p;3

however, since there are two firms, their prices determine what share of the market eachfirm gets. If the firms choose the same price, they split the market half-half. If one firmcharges a strictly higher price, it gets nothing, while the firm that charged less serves thewhole market. More formally, if p i is firm i’s price and p j is its opponent’s price, thenprofits for firm i are ⎧⎨ (4−p i )p i ,p i < p jπ i (p i ,p j ) = (4−p⎩ i )p i /2 ,p i = p j0 ,p i > p jWrite out the strategic form and solve by iterated elimination of weakly dominated strategies.Find all Nash equilibria. Were any Nash equilibria removed by elimination of weaklydominated strategies? Is there an economic argument to focus on any of the eliminatedstrategies that you can think of?If we fill out the strategic form, we get0 1 2 3 40 0,0 0,0 0,0 0,0 0,01 0,0 3/2,3/2 3,0 3,0 3,02 0,0 0,3 1,1 4,0 4,03 0,0 0,3 0,4 1/2,1/2 1,04 0,0 0,3 0,4 0,1 0,0If we do IDWDS, the following happens: 0, 3 and 4 are weakly dominated for bothplayers, by 1 and 2. That leaves us with a strategy set of {1,2} for both players. Butthen 1 weakly dominates 2 for both players, so the outcome of IDWDS is (1,1) — eachplayer uses a price of 1 in equilibrium.Note there are two Nash equilibria: (0,0) and (1,1). You might say the (0,0)equilibrium is silly, but remember that marginal costs here are 0: The model is reallysaying that charging marginal cost or marginal cost plus the smallest unit of currencyarethe equilibriaof the game. Thisis pretty surprising, sinceit says that two competingfirms will behave very similarly to a perfectly competitive market.The interpretation of the previous paragraph is one way of thinking about the game:As long as the players are pricing above marginal cost, the other player has an incentiveto undercut. IDWDS leads to the (1,1) equilibrium, making it a good candidate solution,because it has the appealing feature that the players can reason there separatelyby using strategy dominance arguments. However, like the previous paragraph said, it’sfair to think of the (0,0) equilibrium as one in which the firms are pricing at marginalcost. (Like most things in game theory, there are different arguments that supportdifferent predictions.)4. (a). Consider the following three player simultaneous-move game: Player A choosesthe strategic from {1,2}, player B chooses a row, and C chooses a column. A gets the firstnumber as payoff, B gets the second, C gets the third.4

(1) CL RU 2,2,3 1,1,2B D 1,3,4 1,4,3(2) CL RU 1,1,3 2,1,2B D −2,5,2 2,2,1Justto recap, Player A chooses either the left or the right strategic form above, not knowingwhat players B and C are doing; Player B chooses from U or D, not knowing what playersA or C are doing; and Player C chooses from L or R, not knowing what Players A or B aredoing. Find the dominant strategy equilibrium of the three-player game. (Explain all yourreasoning).It’s a simultaneous game, so the row and column players act as usual, but you alsohave a third player deciding which of (1) and (2) is used. If A picks (1), the row andcolumn players face the left strategic form. For instance, the strategy profile (1,U,L)leads to payoffs (2,2,3), but (2,U,L) leads to payoffs (1,1,3).Despite this, you can solve by eliminating strictly dominated strategies. D strictlydominates U for the Row player (B) in both strategic forms (1) and (2), so you cancross it out on both strategic forms. Then L strictly dominates R for the column playerin both strategic forms. Finally, player A faces (1,3,4) from choosing (1) and (−2,5,2)from choosing (2), so (1) strictly dominates (2) for player A.So the strict dominant strategy equilibrium of the game is (1,D,L).(b). What are the pure-strategy Nash equilibria of the following three-player game:(1) CL RU 2,1,1 0,5,4B D 1,2,4 1,2,3(2) CL RU 0,1,2 2,3,3B D 4,0,5 2,2,3Underlining best responses gives(1) CL RU 2,1,1 0,5,4B D 1,2,4 1,2,3(2) CL RU 0,1,2 2,3,3B D 4,0,5 2,2,3So the Nash equilibrium is (2,U,R).5a. Our assumptions that players are intelligent, rational and have perfect informationis pretty strong. It’s easy to imagine players failing to complete a long string of iterateddeletions of dominated strategies, or not being thoughtful enough to start this process in thefirst place. One suggestion to model learning in games is called “best-response dynamics”,and it works like this: The game is repeated a large number of rounds, and players choosetheir strategy each new round to play a best-response to their opponent’s last move.5

So consider the game below. If the row player used b this round, the column player’sbest-response would have been z for a payoff of 3. If the column player’s strategies aredecided by best-response dynamics, then, we’d predict he should play z in the next round.x y za 3,3 1,2 1,0b 0,1 2,2 3,3c 1,2 -1,-1 4,4(a). Find all Nash equilibria.(b). Show that if you start at (b,y), play eventually reaches (c,z) and stays there permanently.Is this a Nash equilibrium?(c). Show that if you start at (a,z), the learning process never reaches a Nash equilibrium.(d). Is there any starting point that converges to the Nash equilibrium at (a,x)? Why isthis a “fragile” Nash equilibrium under best-response dynamics, especially if players sometimesmake mistakes?(e). Show that if each player uses the same strategy twice, then their strategies form apure-strategy Nash equilibrium, and they will play those strategies in all future periods.(f). Is this a good model of learning or not? Explain your answer (particularly taking youranswer to part (v) into account).i. Find all Nash equilibria. Show that if you start at (b,y), play converges to (c,z).Is this a Nash equilibrium?The Nash equilibria are underlined.Here is a map of how the game moves:(a,x) → (a,x)(a,y) → (b,x)(a,z) → (c,x)(b,x) → (a,z)(b,y) → (b,z)(b,z) → (c,z)(c,x) → (a,z)(c,y) → (b,z)(c,z) → (c,z)Thebestthingtodoisdrawarrowsonthestrategicformshowingtheaboveinformation;this lets you visualize the dynamics.iii. Show that if you start at (a,z), the game never converges to an equilibrium.From the “map” above, (a,z) → (c,x) and (c,x) → (a,z), so the players bounce backand forth between those two profiles forever.iv. Is there any starting point that converges to the Nash equilibrium at (a,x)?Why is this a “fragile” Nash equilibrium under best-response dynamics?Only (a,x). If you look at the map, no profile ends at (a,x) except (a,x) itself. Thismeans that unless the game starts there, the players will never find it, and if eitherplayer makes a mistake and does something else, the game will never return to thisoutcome. Imagine that the dynamics are like a ball rolling on a bowl. If the bowl is6

ight-side up and you drop the ball into the bowl, it will roll to the bottom and staythere. If you tweak the ball, it will return to the bottom. If you flip the bowl upsidedown, you can balance the ball exactly and it will stay there, but if you tweak it, it willroll away. That’s the difference between the Nash equilibrium in the upper-right cornerand the lower-left corner.v. Show that if either player knew the other was using best-response dynamics,that player could “guide” the other player to the Nash equilibrium at (c,z).If the column player uses z twice, the row player will use c on the second roundand they can stay at the “good” Nash equilibrium forever. If the row player uses ctwice, the column player will use z for sure on the second round and they can stay atthe “good” Nash equilibrium forever. This shows that if your opponent is using bestresponsedynamics, you probably don’t have an incentive to use best-responsedynamicsyourself.vi. Is this a good model of learning or not? Explain your answer (particularlytaking your answer to part (v) into account).Yes: It incorporates feedback that players receive through their payoffs to makedecisions about future play. Normal game theory assumes that players “know” whatequilibrium they are using or can reason their way to a unique prediction, which isn’talways a good assumption. Here, the players update over time, and as long as we don’tend up in the cycle they converge fairly quickly to a Nash equilibrium anyway.No: In part iii, iv, and v, we see that players can end up in unrealistic situationsas a result of best-response dynamics, where real players would probably abandon thatapproachandtrysomethingelse. Insteadofusingaforward-looking, deductiveapproachitreliesonabackward-looking, inductiveapproachthatisnotalwaysgoingtoaccuratelypredict future play. Ideally, we’d like something in the middle, but this seems toosimplistic.5b. The purpose of this question is to show that while the tools we have adopted (bestresponses,strategy dominance, iterated deletion of dominated strategies) are useful, thereare other ways to approach the material.Rather than focus on dominance, let’s try another idea, called “rationalizability”. Astrategy is rationalizable if it is a best-response to some profile of strategies your opponentscould use.(a). Write a mathematical definition for a rationalizable strategy similar to the definitionof a dominant strategy, using the u i (s i ,s −i ) notation. It’s OK if you find this hard, or don’tthink your answer is correct; this is just an exercise in thinking in alternative ways aboutplayers, games and behavior.(b). Write out a process similar to iterated deletion of strictly dominated strategies called‘iterated deletion of unrationalizable strategies’. Use it to solve the following game:7

L C RU 3,2 1,1 3,3M 5,3 6,6 2,3D 2,2 -1,5 5,4Is the solution a Nash equilibrium? Try solving the same game using IDDS; do you get adifferent prediction?(c). For prisoners’ dilemma, what are the rationalizable strategies? For battle of thesexes and matching pennies, show that all strategies for all players are rationalizable.(d). Show that no strictly dominated strategy is rationalizable, using your definition from i.Are weakly dominated strategies rationalizable? Show that dominant strategies are alwaysrationalizable. Are rationalizable strategies always dominant?(e). Show that in a pure-strategy Nash equilibrium, both players are using rationalizablestrategies.(a). A strategy s ∗ i is rationalizable if there exists a profile of opponent strategies s −ifor which u i (s ∗ i ,s −i) ≥ u i (s ′ i ,s −i) for any other s ′ i that i could pick.(b). Iterated deletion of unrationalizable strategies: (0) Pick a player. If that playerhas strategies that are not strictly/weakly rationalizable, remove it from the game.Do this for all players. (1) If any strategies were removed in step 0, repeat step 0.Otherwise, stop.The strategy U is not rationalizable for the row player, since it is not a best responseto anything. The strategy L is not rationalizable for the column player, since it is nota best response to anything.With U and L removed, R is no longer a best response to anything the row playermight use, so it is unrationalizable.With R removed, D is not rationalizable for the row player.This leaves (M,C) as the unique profile that survived iterated deletion of unrationalizablestrategies.IDDS fails to remove anything and does not provide a unique prediction.(c). The rationalizable strategy in prisoners’ dilemma is C, since it is a best responseto anything an opponent might play. In battle of the sexes, both strategies arerationalizable since F is a best response to F and B is a best response to B, so eachstrategy is a best response to something an opponent might play. In matching pennies,H is a best response for the row player to H and T is a best response to T, so bothof the row player’s strategies are rationalizable. For the column player, H is a bestresponse to T and T is a best response to H, so both of his strategies are rationalizable.(d). A strictly dominated strategy s 1 is one for which there exists another strategy,s 2 , satisfies u i (s 2 ,s −i ) > u i (s 1 ,s −i ) for all s −i . Consequently, s 2 provides a higherpayoff than s 1 for anything that i’s opponents might do, so s 1 is never a best-responseto anything, so it is not rationalizable.Weakly dominated strategies can be rationalizable, since it might be a best responseto some strategy an opponent might play, for example, inL Ru 2,∗ 5,∗d 2,∗ 1,∗8

the strategy d is a best response to L, so it is rationalizable, even though it is weaklydominated by u.Dominant strategies are always rationalizable. Strategy s ∗ i is a dominant strategyfor player i if u i (s ∗ i ,s −i) ≥ u i (s ′ i ,s −i) for every s −i and any s ′ i that player i could choose.But this implies that s ∗ i is a best responseto any strategy profile, so it is a rationalizablestrategy.Rationalizable strategies are not always dominant. The example above shows this,since u weakly dominates d.(e). InaNashequilibriums ∗ , foreveryplayer i, u i (s ∗ i ,s∗ −i ) ≥ u i(s ′ i ,s∗ −i )foranyothers ′ i that player i could choose. Then s∗ i is a best response to s∗ −i , so it is a rationalizablestrategy.5c. This question involves agents who care about how their payoff compares to that oftheir opponent.We start from a particular strategy profile (a i ,a j ) in a strategic form, where the playershave material payoffs u i (a i ,a j ), that capture the direct payoff to player i of a given strategyprofile (a i ,a j ). The kindness of player j to player i at (a i ,a j ) is given byk ji (a i ,a j ) = u i (a i ,a j )− u i(a i ,a j )+u j (a i ,a j )2This compares the material payoff of player i to the average of the two player’s materialpayoffs. If the average material payoff is larger than player i’s material payoff, k ji will benegative and player j is unkind to player i. If the average payoff is smaller than player i’smaterial payoff, k ji is positive and player j is being kind to player i. Note that if k ji > 0then k ij < 0, so that if one player is being kind, the other player must be unkind.Define the player’s kindness payoffs asU i (a i ,a j ) = u i (a i ,a j )+ck ji (a i ,a j )so that player i cares about his payoff per se, but also how kind his opponent is to him. Thevalue ccan bepositive or negative, and measures how strongly the “kindness”motive affectsthe players’ payoffs. A strategy profile a ∗ = (a ∗ i ,a∗ j ) is a pure-strategy Nash equilibrium ifis a Nash equilibrium in the strategic form game with payoffs (U i (a i ,a j ),U j (a i ,a j )).(a). For c = 3, rewrite the strategic form for Battle of the Sexes with payoffs of U i (a i ,a j )rather than u i (a i ,a j ), where the material payoffs are given byColumnL RRow U 2,1 0,0D 0,0 1,2Do the equilibriumpredictions change? Explain the role that cplays in changing theagents’motives. Now, for c = −1, rewrite the strategic form for Prisoners’ Dilemma with payoffs9

of U i (a i ,a j ) rather than u i (a i ,a j ), where the material payoffs are given byColumnL RRow U 1,1 −1,2D 2,−1 0,0Do the equilibriumpredictions change? Explain the role that cplays in changing theagents’motives.(b). For what values of c are (F,B) or (B,F) pure-strategy Nash equilibria in the Battleof the Sexes game, and for what values of c are (F,F) and (B,B) pure-strategy Nash equilibriain the Battle of the Sexes game? Explain why different values of c lead to differentequilibrium outcomes.(c). Behavioral economists have noted that, “the same people who are altruistic to otheraltruistic people are also motivated to hurt those who hurt them” (Advances in BehavioralEconomics, by Camerer, Loewenstein and Rabin, p. 296). We’ll call this idea reciprocalaltruism. Is the above model a good model of reciprocal altruism? Explain why or why not.(d). Explain whether the following statement is true or false: “The contribution of behavioraleconomics is to explain why the payoffs take the values they do, because once we fixthe players’ payoffs we can then use standard game theory to analyze the strategic form.Therefore, it is without loss of generality to focus on self-regarding players.”(a). The strategic form for battle of the sexes becomesL RU 7/2,−1/2 0,0D 0,0 −1/2,7/2The profiles (U,L) and (D,R) are no longer Nash equilibria. In fact, this becomeslike a matching pennies game, where there is no pure strategy Nash equilibrium. Byincreasing c, the players have become “vindictive”, and prefer to be alone rather thango to the other agent’s prefered event. So players prefer to be unkind.The strategic form for prisoners’ dilemma becomesL RU 1,1 1/2,1/2D 1/2,1/2 0,0Now there is a unique equilibrium at (U,L) rather than (D,R). By decreasing c, playersworry about “hurting” their opponent, so neither player wants to move from Silent toConfess and impose a burden on the other player. So player dislike being unkind.(b). The strategic form isL RU 2+c/2,1−c/2 0,0D 0,0 1−c/2,2+c/210

The usual profiles (U,L) and (D,R) are PSNE ifor 2 ≥ c, and1−c/2 ≥ 02+c/2 ≥ 0so that c > −4. So if 2 ≥ c ≥ −4, then (U,L) and (D,R) are PSNE.The other profiles are PSNE ifor 2 ≤ c, and1−c/2 ≤ 02+c/2 ≤ 0or c ≤ −4. So if c ≤ −4, (U,R) and (D,L) are PSNE.What happens when c > 2? Try 10: The game becomes like matching pennies, withno pure strategy equilibria.As c becomes more positive, the players respond more to the unkindness of theiropponent, and want to hurtpeoplewhohurt them. As c becomes more negative, playersdon’t want to be unkind themselves, and go out of their way to give their opponenttheir preferred outcome.(c). This model can capture one half of reciprocal altruism; it is like a “one-sided”altruism model. Typically, altruism assumes that I just want to make other peoplebetter off per se, even when their actions harm me. In this one-sided altruism model,I either want to hurt players who are unkind to me (c > 0)), or I don’t want to hurtplayers who are kind to me (c < 0). So it captures some of the observations behavioraleconomists have made, but doesn’t incorporate both motivations into the same model.(d). In this version of behavioral economics, we used a hypothesis about humanbehavior to transform the payoffs, but then we used standard game theory to analyzethe transformed payoffs. In this sense, game theory and behavioral economics arecomplementary, and there is no reason you can’t freely mix both together. This exerciseshows that you can build in any behavioral aspects you want simply by transformingthe payoffs the right way. This suggests you can study game theory and behavioraleconomics separately without loss of generality, but then bring them together wheneverit’s appropriate.11