Shape Understanding Using the Laplace Beltrami ... - Visionday
Shape Understanding Using the Laplace Beltrami ... - Visionday
Shape Understanding Using the Laplace Beltrami ... - Visionday
Create successful ePaper yourself
Turn your PDF publications into a flip-book with our unique Google optimized e-Paper software.
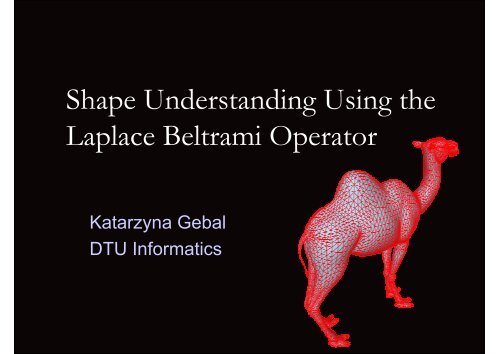
<strong>Shape</strong> <strong>Understanding</strong> <strong>Using</strong> <strong>the</strong><strong>Laplace</strong> <strong>Beltrami</strong> OperatorKatarzyna GebalDTU Informatics
Vectors Find <strong>the</strong> basis: b 1 , b 2 ,…,b nf if ,e if i f i e i Orthonormal To find <strong>the</strong> coordinates project onto <strong>the</strong> basisvectors Meaningful We can extract/remove some information
More dimensions Discreterepresentation Scalar productg ,hi 1 g x i h x iarea i
Functions Continuous functions Scalar productorthogonalitybg x h x dxg ,ha
Fourier analysishttp://mathworld.wolfram.com/FourierSeries.html Fourier seriesb nxe inx2f nf ,b nf xnf n b nx
The 1D case – Fourier & eigenvaluesd 2dx 2 einxn 2 e inx e inx – an eigenvalue of <strong>the</strong> second derivative Linear operators on functions Map functions to functions Matrix representation in discrete case with n×nentries
The 2D case – Fourier & eigenvalues In 2 dimensions second derivative becomes:d 2d 2dx 2 dy 2 Eigenvalues can be found analytically An example – rectangles, zero at <strong>the</strong> boundarya = 1b = 1a = 2b = 1
2sin(x π) sin(1/2 y π)2sin(x π) sin(y π)2sin(x π) sin(3/2 y π)2sin(2 x π) sin(1/2 y π)2sin(x π) sin(2 y π)1.51.51.51.51.5y1y1y1y1y10.50.50.50.50.500 0.5 1x00 0.5 1x00 0.5 1x00 0.5 1x00 0.5 1x2sin(2 x π) sin(y π)2sin(x π) sin(5/2 y π)2sin(2 x π) sin(3/2 y π)2sin(2 x π) sin(2 y π)2sin(3 x π) sin(1/2 y π)1.51.51.51.51.5y1y1y1y1y10.50.50.50.50.500 0.5 1x00 0.5 1x00 0.5 1x00 0.5 1x00 0.5 1x
The <strong>Laplace</strong>-<strong>Beltrami</strong> operator The generalization of <strong>the</strong> second derivative The divergence of <strong>the</strong> gradient In many physical phenomena <strong>the</strong> heat equation <strong>the</strong> wave equationffhttp://en.wikipedia.org/wiki/File:Spherical_wave2.gif http://en.wikipedia.org/wiki/File:Heat_eqn.gif
Eigensolutions of <strong>the</strong> LBO Provide orthonormal basis The generalization of Fourier series Provide not only <strong>the</strong> basis for <strong>the</strong> function butalso a good shape descriptors that dependson <strong>the</strong> shape – eigenvalues and eigenvectorsMinimization of <strong>the</strong> gradient
LBO for a discrete meshjα in×n matrix with entries:Cotan weightsiβ icot i cot jii j i2a iif j j ijcot i cot j2a ia io<strong>the</strong>rwise ij 0Nonzero elements only in a one ringSparse matrices if we need only a small numbers ofeigenvalues
The result
<strong>Shape</strong> filtering (Bruno Levy) Our functions x, y, zpositions of <strong>the</strong> points in <strong>the</strong> space Projecto onto eigenvalues Scale or cut some coefficients Reconstruct back <strong>the</strong> shapef if ,e if i f i e ix y z