Almost-all results on the p^\lambda problem
Almost-all results on the p^\lambda problem
Almost-all results on the p^\lambda problem
Create successful ePaper yourself
Turn your PDF publications into a flip-book with our unique Google optimized e-Paper software.
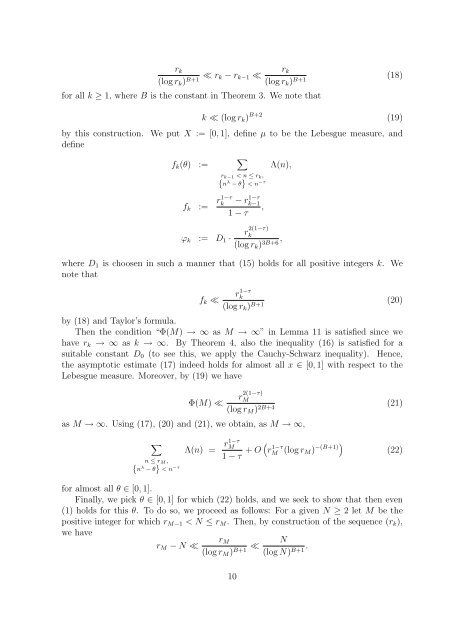
k<br />
(log rk) B+1 ≪ rk − rk−1 ≪<br />
rk<br />
(log rk) B+1<br />
for <str<strong>on</strong>g>all</str<strong>on</strong>g> k ≥ 1, where B is <strong>the</strong> c<strong>on</strong>stant in Theorem 3. We note that<br />
k ≪ (log rk) B+2<br />
by this c<strong>on</strong>structi<strong>on</strong>. We put X := [0, 1], define µ to be <strong>the</strong> Lebesgue measure, and<br />
define<br />
fk(θ) :=<br />
fk := r1−τ k<br />
�<br />
rk−1 < n ≤ rk,<br />
� n λ − θ � < n −τ<br />
− r1−τ k−1<br />
1 − τ<br />
,<br />
Λ(n),<br />
r<br />
ϕk := D1 ·<br />
2(1−τ)<br />
k<br />
,<br />
(log rk) 3B+6<br />
where D1 is choosen in such a manner that (15) holds for <str<strong>on</strong>g>all</str<strong>on</strong>g> positive integers k. We<br />
note that<br />
fk ≪<br />
r1−τ<br />
k<br />
(log rk) B+1<br />
by (18) and Taylor’s formula.<br />
Then <strong>the</strong> c<strong>on</strong>diti<strong>on</strong> “Φ(M) → ∞ as M → ∞” in Lemma 11 is satisfied since we<br />
have rk → ∞ as k → ∞. By Theorem 4, also <strong>the</strong> inequality (16) is satisfied for a<br />
suitable c<strong>on</strong>stant D0 (to see this, we apply <strong>the</strong> Cauchy-Schwarz inequality). Hence,<br />
<strong>the</strong> asymptotic estimate (17) indeed holds for almost <str<strong>on</strong>g>all</str<strong>on</strong>g> x ∈ [0, 1] with respect to <strong>the</strong><br />
Lebesgue measure. Moreover, by (19) we have<br />
Φ(M) ≪<br />
r2(1−τ)<br />
M<br />
(log rM) 2B+4<br />
as M → ∞. Using (17), (20) and (21), we obtain, as M → ∞,<br />
�<br />
n ≤ rM ,<br />
� n λ − θ � < n −τ<br />
Λ(n) = r1−τ M<br />
1 − τ<br />
+ O �<br />
r 1−τ<br />
M (log rM) −(B+1)�<br />
for almost <str<strong>on</strong>g>all</str<strong>on</strong>g> θ ∈ [0, 1].<br />
Fin<str<strong>on</strong>g>all</str<strong>on</strong>g>y, we pick θ ∈ [0, 1] for which (22) holds, and we seek to show that <strong>the</strong>n even<br />
(1) holds for this θ. To do so, we proceed as follows: For a given N ≥ 2 let M be <strong>the</strong><br />
positive integer for which rM−1 < N ≤ rM. Then, by c<strong>on</strong>structi<strong>on</strong> of <strong>the</strong> sequence (rk),<br />
we have<br />
rM − N ≪<br />
rM<br />
≪<br />
(log rM) B+1<br />
10<br />
N<br />
.<br />
(log N) B+1<br />
(18)<br />
(19)<br />
(20)<br />
(21)<br />
(22)