t b a b a

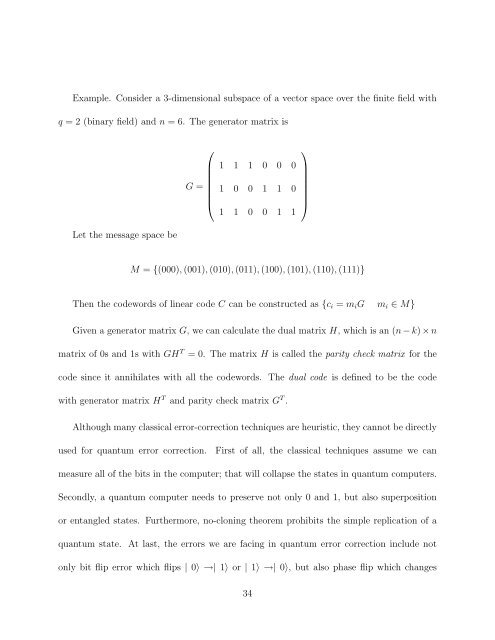
Example. Consider a 3-dimensional subspace of a vector space over the finite field with
q = 2 (binary field) and n = 6. The generator matrix is
Let the message space be
⎛
⎞
⎜
G = ⎜
⎝
1
1
1
0
1
0
0
1
0
1
0 ⎟
0
⎟
⎠
1 1 0 0 1 1
M = {(000), (001), (010), (011), (100), (101), (110), (111)}
Then the codewords of linear code C can be constructed as {ci = miG mi ∈ M}
Given a generator matrix G, we can calculate the dual matrix H, which is an (n − k) × n
matrix of 0s and 1s with GH T = 0. The matrix H is called the parity check matrix for the
code since it annihilates with all the codewords. The dual code is defined to be the code
with generator matrix H T and parity check matrix G T .
Although many classical error-correction techniques are heuristic, they cannot be directly
used for quantum error correction. First of all, the classical techniques assume we can
measure all of the bits in the computer; that will collapse the states in quantum computers.
Secondly, a quantum computer needs to preserve not only 0 and 1, but also superposition
or entangled states. Furthermore, no-cloning theorem prohibits the simple replication of a
quantum state. At last, the errors we are facing in quantum error correction include not
only bit flip error which flips | 0〉 →| 1〉 or | 1〉 →| 0〉, but also phase flip which changes
34