Homework 8 EE235, Spring 2012 Solutions 1. Find the (unilateral ...

Homework 8
EE235, Spring 2012
Solutions
1. Find the (unilateral) Laplace transform of the following signals:
(a) x(t) = e −t u(t)
(b) x(t) = e −t (t − 2)u(t − 2)
(c) x(t) = t 2 e −2t u(t)
X(s) = 1
s + 1 .
X(s) = e−2(s+1)
.
(s + 1) 2
X(s) =
2
.
(s + 2) 3
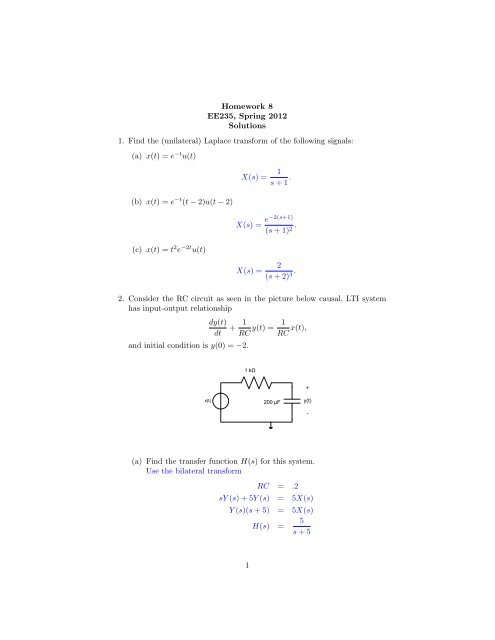
2. Consider the RC circuit as seen in the picture below causal, LTI system
has input-output relationship
dy(t)
dt
and initial condition is y(0) = −2.
x(t)
1 1
+ y(t) =
RC RC x(t),
1 kΩ
200 µF
(a) Find the transfer function H(s) for this system.
Use the bilateral transform
RC = .2
+
y(t)
sY (s) + 5Y (s) = 5X(s)
Y (s)(s + 5) = 5X(s)
H(s) =
5
s + 5
1
-

(b) What are the poles and zeros of this H(s)? Plot the corresponding
pole-zero plot.
There are no zeros for this function and a pole at s = −5.
Imaginary Axis
6
4
2
0
−2
−4
−6
Pole−Zero Map
−6 −4 −2 0
Real Axis
2 4 6
(c) With the given initial conditions, find the system response y(t) to an
input x(t) = 3
5 e−2t u(t).
Use the unilateral transform since we are given the initial condition
RC = .2
sY (s) − y(0 − ) + 5Y (s) = 5X(s)
Y (s)(s + 5) = 5X(s) + y(0 − )
Y (s) =
1
s + 5 (5X(s) + y(0− ))
X(s) = 3 1
5 s + 2
Y (s) =
3
2
−
(s + 5)(s + 2) s + 5
Y (s) =
1 1 2
− −
s + 2 s + 5 s + 5
Y (s) =
1 3
−
s + 2 s + 5
y(t) = (e −2t − 3e −5t )u(t)
(d) Use the initial value theorem to find the initial value of y(t), i.e.,
y(0 + ). Confirm your answer with your result in part (c).
2

The initial value theorem states
lims→∞sY (s) = y(0 + )
Y (s) =
1 3
−
s + 2 s + 5
lims→∞sY (s) = lims→∞s( 1
= lims→∞
= 1 − 3
= −2
1 + 2
s
− 3
s + 5 )
s + 2
1
− 3
1 + 5
s
This is what we expect since the initial condition is -2 and the voltage
across the capacitor cannot change instantaneously to match the
forcing function.
(e) Use the final value theorem to find the final value of y(t), i.e., the
value of y(t) when t → +∞. Confirm your answer with your result
in part (c).
The initial value theorem states
lims→0sY (s) = limt→∞y(t)
Y (s) =
1 3
−
s + 2 s + 5
lims→0sY (s) = lims→0s( 1 3
−
s + 2 s + 5 )
= 0 1
2
= 0
− 03
5
This is what we expect because at t → ∞ we expect the voltage
across the capacitor to match the voltage of the forcing function. At
time t → ∞, x(t) = 0.
3. For an LTI system with transfer function H(s) = 3s(s−1)
(s−2)(s+3) , give its natural
modes and state if the system is stable/unstable/marginally stable.
Justify your answer.
The natural modes are e2t and e−3t . The poles to the system are at
s = 2, −3. The system is unstable due to the pole at s = 2.
4. Consider a causal LTI system is described by
d2y(t) − y(t) = 4x(t)
dt2 with all initial conditions zero. An input x(t) = cos(5t)u(t) is applied.
3

(a) Use (unilateral) Laplace transforms to find the output y(t).
d2y(t) − y(t)
dt2 =
↓
4x(t)
s 2 Y (s) − d
dt x(t)|t=0 − sx(t)|t=0 − Y (s) = 4X(s)
Y (s)(s 2 − 1) = 4X(s)
Y (s) =
4
s2 s
− 1 s2 + 25
Y (s) = 2 s
13 s2 2 s
−
− 1 13 s2 + 25
Y (s) = 2 1 1 2 s
( + ) −
26 s − 1 s + 1 13 s2 ↓
+ 25
y(t) = 2
26 (et + e −t )u(t) − 2
13 cos(5t)u(t)
(b) State if the system is stable/unstable/marginally stable. Justify your
answer.
The system is unstable because there is a pole at s = 1.
5. Consider the system with the impulse response
h(t) = δ(t) + e −3t u(t) + 2e −t u(t)
(a) Find the transfer function of the inverse system.
Want to find G(s) such that G(s) = 1
H(s) .
H(s) = 1 + 1 2
+
s + 3 s + 1
H(s) =
(s + 3)(s + 1)
(s + 3)(s + 1) +
(s + 1)
(s + 3)(s + 1) +
2(s + 3)
(s + 3)(s + 1)
H(s) = s2 + 4s + 3 + 2s + 6 + s + 1
(s + 3)(s + 1)
H(s) = s2 + 7s + 10
(s + 3)(s + 1)
G(s) =
(s + 3)(s + 1)
s2 + 7s + 10
G(s) =
(s + 3)(s + 1)
(s + 2)(s + 5)
4

(b) Does a stable and causal inverse system exist?
Yes a causal, stable inverse exists. The inverse system has poles at
s = −2, −5.
6. Consider a system with a causal impulse response h(t). Its system transfer
function H(s) has the following pole-zero plot,
(a) Given that this impulse response h(t) is a causal signal, what is the
region of convergence?
causal ⇒ right-sided ⇒ ROC: Re{s} > 1
(b) Find h(t) (you can keep any constant multiplier in your solution).
If we keep constant multiplier A, we have
where
H(s) =
=
A
(s + 3)(s + 2 − j)(s + 2 + j)(s − 1)
A1
s + 3 +
A2
s + 2 − j +
A3 A4
+
s + 2 + j s − 1 ,
A1 = − 1
8 A, A2
1 + 2j
=
20 A, A3
1 − 2j
=
20 A, A4 = 1
40 A.
So the corresponding causal impulse response is
h(t) = A1e −3t u(t) + A2e −(2−j)t u(t) + A3e −(2+j)t u(t) + A4e t u(t)
= − 1
8 Ae−3t u(t) + 1
10 Ae−2t (cos t − 2 sin t)u(t) + 1
40 Aet u(t)
(c) Is the system with this impulse response h(t) a BIBO stable system?
No, this system has a pole s = 1 on the right half-plane, so it is not
BIBO stable.
5

(d) We form another causal LTI system, whose system transfer function
is H1(s) = (s + 2 + j)H(s). Let h1(t) be the impulse response of this
new system. Is h1(t) a real signal? Justify your answer.
Since
H1(s) = (s + 2 + j)H(s) =
A
(s + 3)(s + 2 − j)(s − 1) ,
which has three poles, h1(t) cannot be real, because for real signals,
if they have complex poles or zeros, they must be in conjugate pairs.
Here for H1(s), it has a complex pole at s = −2 + j, but it does not
have a pole at s = −2 − j, so h1(t) cannot be real.
6