Fonctions trigonométriques et fonctions hyperboliques

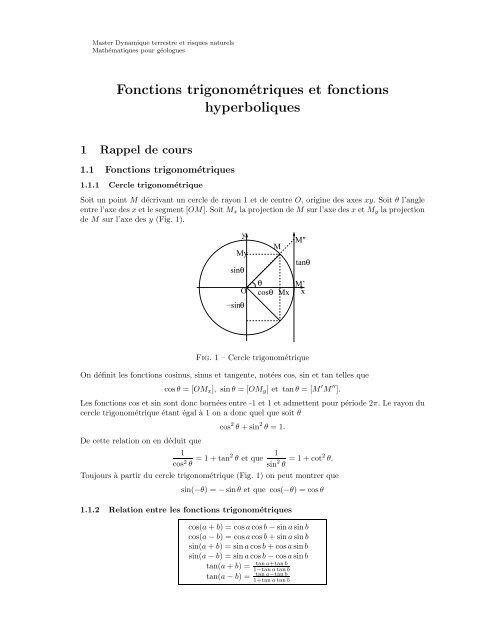
Master Dynamique terrestre et risques naturelsMathématiques pour géologuesFonctions trigonométriques et fonctionshyperboliques1 Rappel de cours1.1 Fonctions trigonométriques1.1.1 Cercle trigonométriqueSoit un point M décrivant un cercle de rayon 1 et de centre O, origine des axes xy. Soit θ l’angleentre l’axe des x et le segment [OM]. Soit M x la projection de M sur l’axe des x et M y la projectionde M sur l’axe des y (Fig. 1).yMysinθO−sinθθcosθMMxM"tanθM’xFig. 1 – Cercle trigonométriqueOn définit les fonctions cosinus, sinus et tangente, notées cos, sin et tan telles quecosθ = [OM x ], sin θ = [OM y ] et tan θ = [M ′ M ′′ ].Les fonctions cos et sin sont donc bornées entre -1 et 1 et admettent pour période 2π. Le rayon ducercle trigonométrique étant égal à 1 on a donc quel que soit θDe cette relation on en déduit quecos 2 θ + sin 2 θ = 1.1cos 2 θ = 1 + 1tan2 θ et quesin 2 θ = 1 + cot2 θ.Toujours à partir du cercle trigonométrique (Fig. 1) on peut montrer quesin(−θ) = − sinθ et que cos(−θ) = cosθ1.1.2 Relation entre les fonctions trigonométriquescos(a + b) = cosacosb − sin a sin bcos(a − b) = cosacosb + sin a sin bsin(a + b) = sin a cosb + cosasinbsin(a − b) = sin a cosb − cosasinbtan a+tan btan(a + b) =tan(a − b) =1−tan atan btan a−tan b1+tan atan b

En faisant b = a on obtient les formules de multiplication par 2sin(2a) = 2 sinacosacos(2a) = cos 2 a − sin 2 a = 2 cos 2 a − 1 = 1 − 2 sin 2 asin(2a) = 2 tan a1+tan 2 acos(2a) = 1−tan2 a1+tan 2 atan(2a) = 2tan a1−tan 2 a .En posant a + b = p et a − b = q les formules précèdentes peuvent s’exprimer comme suit1.1.3 Formules d’EulerOn définit e iy paret en changeant y par −ycos(p) + cos(q) = 2 cos p+q2cos(p) − cos(q) = −2 sin p+q2sin(p) + sin(q) = 2 sin p+qsin(p) − sin(q) = 2 cos p+q2e iy = cosy + i sinye −iy = cosy − i siny.p−qcos2p−qsin22cos p−q2sinp−q2En ajoutant et en retrancheant membre à membre ces deux égalités, on obtient les formules d’Euler1.1.4 Formules de Moivrescosy = eiy +e −iy2etsin y = eiy −e −iy2iÀ partir des formules d’Euler on peut déduire facilement les formules de Moivre(cos x + i sinx) n = cosnx + i sinnx et (cos x − i sin x) n = cosnx − i sinnx1.2 Fonctions hyperboliques1.2.1 DéfinitionsPar définition on appelle cosinus hyperbolique de x, qu’on note coshx la quantitéde même le sinus hyperbolique de x estcoshx = ex + e −x,2sinhx = ex − e −x.2Par analogie avec les fonctions trigonométriques on définit la tangente hyperbolique de x partanhx = sinhxcoshx = ex − e −xe x + e −x,et la cotangente hyperbolique parcothx = 1tanhx .

1.3 Égalités utilesPar définition on aet par conséquentDe cette relation on en déduit quecoshx + sinhx = e x et coshx − sinhx = e −x ,cosh 2 x − sinh 2 x = 1.1cosh 2 = 1 − tanh2 x et que1sinh 2 = coth2 x − 1.1.4 Relation entre les fonctions hyperboliquescosh(a + b) = coshacoshb + sinhasinhbcosh(a − b) = coshacoshb − sinhasinhbsinh(a + b) = sinhacoshb + cosh a sinhbsinh(a − b) = sinhacoshb − cosh a sinhb2 Exercicescosh(p) + cosh(q) = 2 cosh p+q p−q2cosh2cosh(p) − cosh(q) = 2 sinh p+q p−q2sinh2sinh(p) + sinh(q) = 2 sinh p+q2cosh p−q2sinh(p) − sinh(q) = 2 cosh p+q p−q2sinh21. Démontrer les formules de Moivres, puis les utiliser pour calculer cos(3θ) et sin(3θ) en fonctionde cosθ et sin θ.2. Déduire à partir des formules d’Euler l’expression de sin(2x) et cos(2x) en fonction de sinxet cosx.3. θ étant compris entre −πque2et π 2, exprimer sinhx, coshx et tanhx en fonction de θ, sachant[ θx = ln tan2 + π ].44. Résoudre le système pour a, α ∈ R.{ coshx + coshy = a coshαsinhx + sinhy = a sinhα5. Déterminer l’expression de (coshx − sinhx) n et de (cosh x + sinhx) n en fonction de coshnxet sinh nx.