ANALYSIS QUALIFYING EXAM PROBLEMS BRIAN LEARY ...
ANALYSIS QUALIFYING EXAM PROBLEMS BRIAN LEARY ...
ANALYSIS QUALIFYING EXAM PROBLEMS BRIAN LEARY ...
You also want an ePaper? Increase the reach of your titles
YUMPU automatically turns print PDFs into web optimized ePapers that Google loves.
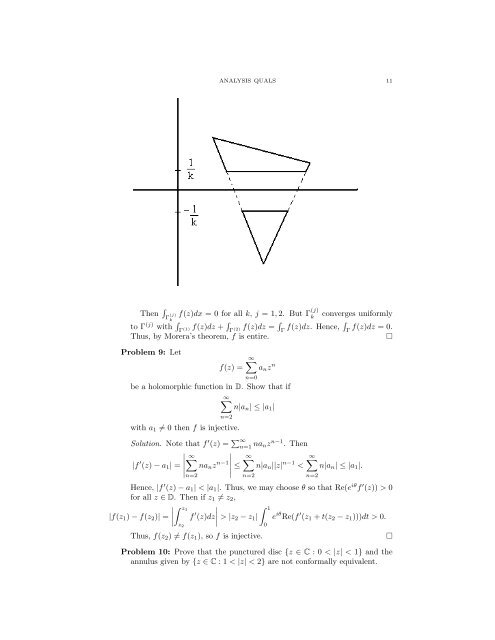
Then <br />
Γ (j)<br />
k<br />
<strong>ANALYSIS</strong> QUALS 11<br />
f(z)dx = 0 for all k, j = 1, 2. But Γ (j)<br />
k converges uniformly<br />
to Γ (j) with <br />
Γ (1) f(z)dz + <br />
Γ (2) f(z)dz = <br />
<br />
f(z)dz. Hence, f(z)dz = 0.<br />
Γ Γ<br />
Thus, by Morera’s theorem, f is entire. <br />
Problem 9: Let<br />
f(z) =<br />
∞<br />
anz n<br />
n=0<br />
be a holomorphic function in D. Show that if<br />
∞<br />
n|an| ≤ |a1|<br />
n=2<br />
with a1 = 0 then f is injective.<br />
Solution. Note that f ′ (z) = ∞ n=1 nanzn−1 . Then<br />
|f ′ <br />
∞ <br />
(z) − a1| = nanz<br />
<br />
n−1<br />
<br />
<br />
<br />
<br />
≤<br />
∞<br />
n|an||z| n−1 ∞<br />
< n|an| ≤ |a1|.<br />
n=2<br />
n=2<br />
Hence, |f ′ (z) − a1| < |a1|. Thus, we may choose θ so that Re(e iθ f ′ (z)) > 0<br />
for all z ∈ D. Then if z1 = z2,<br />
<br />
z1 <br />
|f(z1) − f(z2)| = <br />
f ′ <br />
<br />
(z)dz<br />
> |z2 − z1|<br />
z2<br />
1<br />
0<br />
n=2<br />
e iθ Re(f ′ (z1 + t(z2 − z1)))dt > 0.<br />
Thus, f(z2) = f(z1), so f is injective. <br />
Problem 10: Prove that the punctured disc {z ∈ C : 0 < |z| < 1} and the<br />
annulus given by {z ∈ C : 1 < |z| < 2} are not conformally equivalent.