Chapter 7 Infinite product spaces

7.2. PROOF OF BOREL ISOMORPHISM THEOREM 3
7.2 Proof of Borel isomorphism theorem
The proof invokes a lot of nice arguments that have their core in set theory. We
begin by developing a tool for proving equivalence of measure spaces:
Theorem 7.3 [Cantor-Schröder-Bernstein] Let A ⇢ X and B ⇢ Y be sets such that
there exist bijections f : X ! B and g : Y ! A. Then there exists a bijection h : X ! Y.
Moreover, if F is a s-algebra on X and G is a s-algebra on Y, and if f , g 1 are F /G -
measurable and f 1 , g are G /F -measurable, then h can be taken F /G -measurable.
Proof. Let C =(g f )(X) ⇢ A and note that the sets
{(g f ) n (X \ A)}n 0, {(g f ) n (A \ C)}n 0, \
(g f ) n (X) (7.5)
form a disjoint partition of X. We define
n 0
8
>< f (x), if x 2
h(x) =
>:
S
n 0(g f ) n (X \ A),
g 1 (x), if x 2 S
n 0(g f ) n (A \ C),
g 1 (x), if x 2 T
n 0(g f ) n (X).
(7.6)
(Note that we could have also defined h(x) to be f (x) in the last case because g
f maps the intersection onto itself in one-to-one fashion.) Since g : Y ! A is a
bijection, we need to show that g h : X ! A is a bijection. In the latter two cases g
X
A
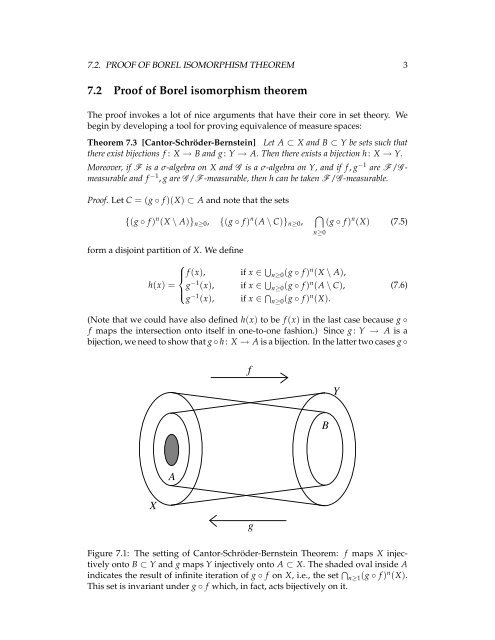
Figure 7.1: The setting of Cantor-Schröder-Bernstein Theorem: f maps X injectively
onto B ⇢ Y and g maps Y injectively onto A ⇢ X. The shaded oval inside A
indicates the result of infinite iteration of g f on X, i.e., the set T
n 1(g f ) n (X).
This set is invariant under g f which, in fact, acts bijectively on it.
f
g
B
Y