Eisenstein series and approximations to pi - Mathematics
Eisenstein series and approximations to pi - Mathematics
Eisenstein series and approximations to pi - Mathematics
Create successful ePaper yourself
Turn your PDF publications into a flip-book with our unique Google optimized e-Paper software.
12 BRUCE C. BERNDT AND HENG HUAT CHAN<br />
where<br />
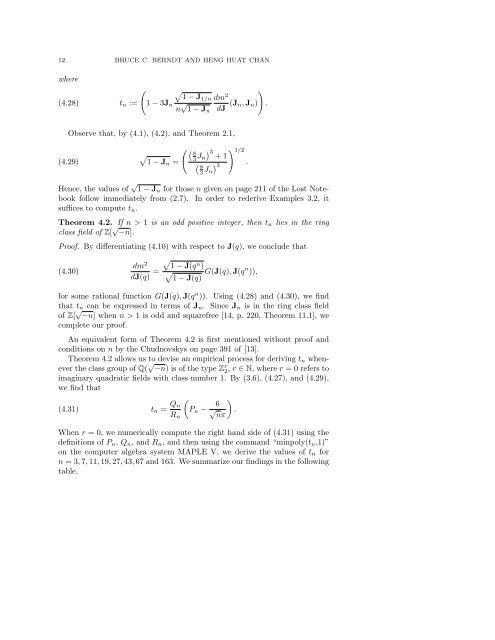
<br />
1 − J1/n<br />
(4.28) tn := 1 − 3Jn<br />
n √ 1 − Jn<br />
Observe that, by (4.1), (4.2), <strong>and</strong> Theorem 2.1,<br />
(4.29)<br />
1 − Jn =<br />
<br />
8<br />
3Jn <br />
8<br />
3Jn 3 dm2 dJ (Jn,<br />
<br />
Jn) .<br />
3 + 1<br />
1/2<br />
Hence, the values of √ 1 − Jn for those n given on page 211 of the Lost Notebook<br />
follow immediately from (2.7). In order <strong>to</strong> rederive Examples 3.2, it<br />
suffices <strong>to</strong> compute tn.<br />
Theorem 4.2. If n > 1 is an odd positive integer, then tn lies in the ring<br />
class field of Z[ √ −n].<br />
Proof. By differentiating (4.10) with respect <strong>to</strong> J(q), we conclude that<br />
(4.30)<br />
dm2 dJ(q) =<br />
<br />
1 − J(qn )<br />
G(J(q), J(q<br />
1 − J(q) n )),<br />
for some rational function G(J(q), J(q n )). Using (4.28) <strong>and</strong> (4.30), we find<br />
that tn can be expressed in terms of Jn. Since Jn is in the ring class field<br />
of Z[ √ −n] when n > 1 is odd <strong>and</strong> squarefree [14, p. 220, Theorem 11.1], we<br />
complete our proof.<br />
An equivalent form of Theorem 4.2 is first mentioned without proof <strong>and</strong><br />
conditions on n by the Chudnovskys on page 391 of [13].<br />
Theorem 4.2 allows us <strong>to</strong> devise an em<strong>pi</strong>rical process for deriving tn whenever<br />
the class group of Q( √ −n) is of the type Zr 2, r ∈ N, where r = 0 refers <strong>to</strong><br />
imaginary quadratic fields with class number 1. By (3.6), (4.27), <strong>and</strong> (4.29),<br />
we find that<br />
(4.31) tn = Qn<br />
<br />
Pn − 6<br />
<br />
√ .<br />
nπ<br />
Rn<br />
When r = 0, we numerically compute the right h<strong>and</strong> side of (4.31) using the<br />
definitions of Pn, Qn, <strong>and</strong> Rn, <strong>and</strong> then using the comm<strong>and</strong> “minpoly(tn,1)”<br />
on the computer algebra system MAPLE V, we derive the values of tn for<br />
n = 3, 7, 11, 19, 27, 43, 67 <strong>and</strong> 163. We summarize our findings in the following<br />
table.<br />
.