A refactored proof of conceptual completeness

Then I ∗ stabilizes every S ≤ IA so by 3.1(ii), I is full on subobjects.
Now suppose that f : IA → IA ′ ∈ F. We can represent f as a graph
Γf ↣ IA×IA ′ . Because I is full on subobjects, Γf has a preimage R ↣ A×A ′ .
If I ∗ is also essentially surjective then I will be conservative. From conservativity
and the fact that Γf was a provably function relation one can easily show that
R must be provably functional. Then I sends the composite R ↣ A × A ′ → A ′
to f, and I is full.
Now assume that I ∗ is additionally faithful, so that it is an equivalence of
categories. By 3.1(iii), this means that I is finitely covering. For any B ∈ F
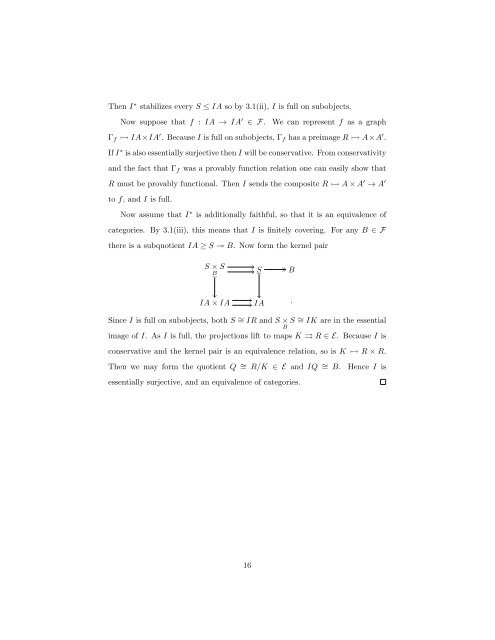
there is a subquotient IA ≥ S ↠ B. Now form the kernel pair
S × S
B
IA × IA
S
IA
Since I is full on subobjects, both S ∼ = IR and S × S
B
∼ = IK are in the essential
image of I. As I is full, the projections lift to maps K ⇒ R ∈ E. Because I is
conservative and the kernel pair is an equivalence relation, so is K ↣ R × R.
Then we may form the quotient Q ∼ = R/K ∈ E and IQ ∼ = B. Hence I is
B
essentially surjective, and an equivalence of categories.
16
.