Benoit Palmieri and David Ronis, Diffusion in ... - McGill University
Benoit Palmieri and David Ronis, Diffusion in ... - McGill University
Benoit Palmieri and David Ronis, Diffusion in ... - McGill University
Create successful ePaper yourself
Turn your PDF publications into a flip-book with our unique Google optimized e-Paper software.
<strong>Diffusion</strong> <strong>in</strong> Channeled Structures J. Phys. Chem. B, Vol. 109, No. 45, 2005 21335<br />
-dependent Onsager diffusion coefficient given by<br />
q<br />
where Jz is the z component of the irreversible or dissipative<br />
part of the current <strong>and</strong> A is the area of the crystal. Also note<br />
that the <strong>in</strong>tegration variable, z, is def<strong>in</strong>ed to be normal to the<br />
<strong>in</strong>terface (i.e., parallel to the net flux) <strong>and</strong> is perpendicular to<br />
r|.<br />
The time correlation function that appears <strong>in</strong> eq 2.3 is not<br />
q<br />
directly amenable to simulation s<strong>in</strong>ce the dynamics <strong>in</strong> Jz(r, t)<br />
<strong>in</strong>clude a projection operator that projects out the slow parts of<br />
the motion (<strong>in</strong> practice, the parts not <strong>in</strong>cluded explicitly <strong>in</strong> the<br />
macroscopic equations). 12 By manipulat<strong>in</strong>g the well-known<br />
<strong>in</strong>tegral equations relat<strong>in</strong>g correlation <strong>and</strong> memory functions,<br />
Vertenste<strong>in</strong> <strong>and</strong> <strong>Ronis</strong>2,12 obta<strong>in</strong>ed an approximate expression<br />
for D(z) by assum<strong>in</strong>g <strong>in</strong>f<strong>in</strong>ite dilution <strong>in</strong>side the material <strong>and</strong><br />
that D(z) eW(z) is approximately constant near the W(z) barrier<br />
tops; the result is that<br />
where n∞ is the number density of the guest <strong>in</strong> the bulk, Vz is<br />
the z component of the guest velocity, 〈...〉r denotes a conditional<br />
equilibrium average under the constra<strong>in</strong>t that the <strong>in</strong>itial position<br />
of the guest is r, <strong>and</strong> W(z) <strong>and</strong> F(z) are the plane averaged<br />
potential of mean force <strong>and</strong> mean force of the guest <strong>in</strong> the plane<br />
z, respectively (we dist<strong>in</strong>guish the plane average quantities <strong>and</strong><br />
their 3D counterparts by the argument to the function, z <strong>in</strong> the<br />
former <strong>and</strong> r <strong>in</strong> the latter); <strong>in</strong> particular,<br />
Note that the denom<strong>in</strong>ator <strong>in</strong> eq 2.4 approximately reproduces<br />
the effect of the projection operator that is implicit <strong>in</strong> J q , the<br />
irreversible part of the current, compare to eq 2.3.<br />
It follows from eqs 2.3 or 2.4 that D(z) will be small when<br />
z is a barrier-top plane, <strong>and</strong> it is just these planes that give the<br />
ma<strong>in</strong> contributions to the permeability, compare to eq 2.2.<br />
Hence, provided that a practical way is found for calculat<strong>in</strong>g<br />
the potential of mean force near the transition states (e.g.,<br />
analytically or us<strong>in</strong>g umbrella sampl<strong>in</strong>g), our theory becomes<br />
a transition state sampl<strong>in</strong>g method, only requir<strong>in</strong>g a knowledge<br />
of dynamical correlations for <strong>in</strong>itial states near the transition<br />
state.<br />
Clearly, it is necessary to simulate the guest dynamics <strong>in</strong>side<br />
the crystal <strong>in</strong> order to calculate the time correlation functions<br />
appear<strong>in</strong>g <strong>in</strong> eq 2.4. As described <strong>in</strong> I, we assume that the guest<br />
<strong>in</strong>teracts with a limited number of crystal atoms (referred to as<br />
the “target atoms”, or T for short). The effect of the rest of the<br />
crystal (the bath) will be described us<strong>in</strong>g a projection operator<br />
approach, 13 where the projection operator is an average over<br />
the bath degrees of freedom <strong>and</strong> gives rise to the follow<strong>in</strong>g<br />
generalized Langev<strong>in</strong> equation:<br />
<strong>and</strong><br />
D(z) ) 1<br />
A ∫ ∞ q q<br />
dt<br />
0 ∫ dr |∫ dr′〈Jz(r, t) Jz(r′)〉<br />
(2.3)<br />
n∞ ∫0 D(z) )<br />
A + ∫0 ∞ dt1∫ dr | 〈V z (t 1 )V z 〉 r e -W(r)<br />
∞ dt1∫ dr | 〈F[z(t 1 )]V z 〉 r e -[W(r)-W(z)]<br />
e -W(z) ≡ A -1 ∫ dr | e -W(r)<br />
dp(t)<br />
dt )-dU[r(t),R T (t)]<br />
dr<br />
(2.4)<br />
(2.5)<br />
dPT (t)<br />
dt )-KeffRT (t) - dU[r(t),RT (t)]<br />
+<br />
where U is the potential <strong>in</strong>teraction between the guest <strong>and</strong> the<br />
target atoms, RT <strong>and</strong> PT are, respectively, the target position<br />
<strong>and</strong> momentum column vectors, Keff is an effective force<br />
q<br />
constant matrix, FT is a colored Gaussian noise column vector<br />
q q<br />
with zero mean <strong>and</strong> variance matrix 〈FT(t)(FT) T 〉, <strong>and</strong> the T<br />
superscript <strong>in</strong>dicates a transpose.<br />
The projection operator drops out of the time dependence of<br />
the memory function, which is obta<strong>in</strong>ed by consider<strong>in</strong>g the<br />
harmonic motion of the bath <strong>in</strong> the presence of frozen target<br />
<strong>and</strong> guest. Note that these equations are exact, provided that<br />
the <strong>in</strong>teraction between the crystal atoms is harmonic <strong>and</strong><br />
provided that the guest does not directly <strong>in</strong>teract with the bath<br />
(this last po<strong>in</strong>t can be relaxed somewhat to allow for direct<br />
harmonic <strong>in</strong>teractions between the guest <strong>and</strong> the bath). In I, we<br />
described, <strong>in</strong> detail, the procedures that allow us to calculate<br />
q q<br />
Keff <strong>and</strong> 〈FT(t)(FT) T 〉. When the Laplace transform of the<br />
memory function is approximately written as<br />
the equations of motions simplify to a set of coupled stochastic<br />
differential equations with additive white noise, which, by<br />
properly choos<strong>in</strong>g the matrices A, B, <strong>and</strong> C, reproduces the basic<br />
features of the <strong>in</strong>f<strong>in</strong>ite crystal vibrational density of states (cf.<br />
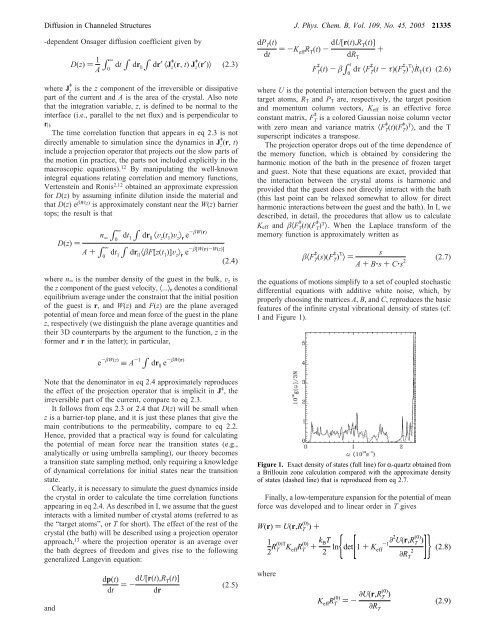
I <strong>and</strong> Figure 1).<br />
F<strong>in</strong>ally, a low-temperature expansion for the potential of mean<br />
force was developed <strong>and</strong> to l<strong>in</strong>ear order <strong>in</strong> T gives<br />
where<br />
dRT q t q q T<br />
FT(t) - ∫ dτ 〈FT(t<br />
- τ)(FT)<br />
〉R˙<br />
0<br />
T (τ) (2.6)<br />
q q T<br />
〈FT(s)(FT) 〉 )<br />
s<br />
A + B‚s + C‚s 2<br />
(2.7)<br />
Figure 1. Exact density of states (full l<strong>in</strong>e) for R-quartz obta<strong>in</strong>ed from<br />
a Brillou<strong>in</strong> zone calculation compared with the approximate density<br />
of states (dashed l<strong>in</strong>e) that is reproduced from eq 2.7.<br />
(0)<br />
W(r) ) U(r,RT ) +<br />
1<br />
2 R k (0)T (0) BT T KeffRT +<br />
2 ln{ det[ 1 + Keff (0)<br />
∂U(r,R (0) T )<br />
KeffRT )-<br />
∂RT -1∂2 (0)<br />
U(r,RT )<br />
]} 2<br />
∂RT (2.8)<br />
(2.9)