On a forcing model for non-standard arithmetic

Sheaf semantics A non-principal ultrafilter A forcing model for non-standard arithmetic Open questions
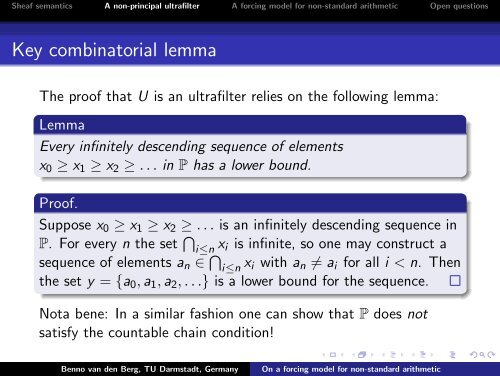
Key combinatorial lemma
The proof that U is an ultrafilter relies on the following lemma:
Lemma
Every infinitely descending sequence of elements
x0 ≥ x1 ≥ x2 ≥ . . . in P has a lower bound.
Proof.
Suppose x0 ≥ x1 ≥ x2 ≥ . . . is an infinitely descending sequence in
P. For every n the set
i≤n xi is infinite, so one may construct a
sequence of elements an ∈
i≤n xi with an = ai for all i < n. Then
the set y = {a0, a1, a2, . . .} is a lower bound for the sequence.
Nota bene: In a similar fashion one can show that P does not
satisfy the countable chain condition!
Benno van den Berg, TU Darmstadt, Germany On a forcing model for non-standard arithmetic