1.2 Directional Derivatives
1.2 Directional Derivatives
1.2 Directional Derivatives
Create successful ePaper yourself
Turn your PDF publications into a flip-book with our unique Google optimized e-Paper software.
Functions and their properties 9<br />
y ∂z<br />
∂y<br />
x ∂z ∂z<br />
+ y<br />
∂x ∂y<br />
=<br />
x 2<br />
x 2 + y 2<br />
1<br />
= y ·<br />
2 (<br />
1<br />
x2 ) · (2y)<br />
+ y2 =<br />
= 1<br />
y 2<br />
x 2 + y 2<br />
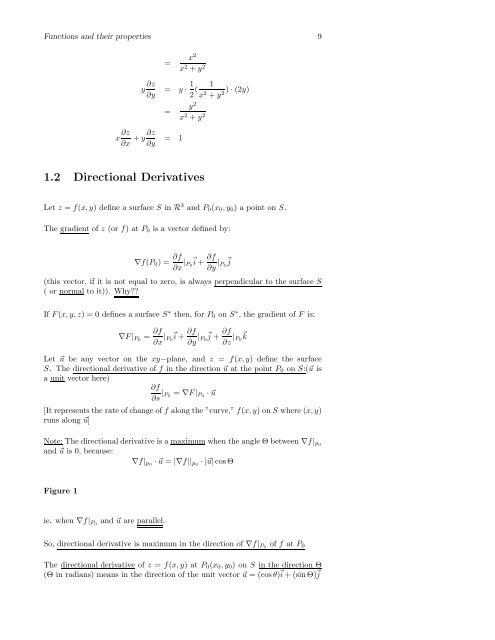
<strong>1.2</strong> <strong>Directional</strong> <strong>Derivatives</strong><br />
Let z = f(x, y) define a surface S in R 3 and P0(x0,y0) apointonS.<br />
The gradient of z (or f) atP0 is a vector defined by:<br />
∇f(P0) = ∂f<br />
∂x |P0 i + ∂f<br />
|P0<br />
j<br />
∂y<br />
(this vector, if it is not equal to zero, is always perpendicular to the surface S<br />
(ornormalto it)). Why??<br />
If F (x, y, z) = 0 defines a surface S ∗ then, for P0 on S ∗ , the gradient of F is:<br />
∇F |P0 = ∂f<br />
∂x |P0 i + ∂f<br />
∂y |P0 j + ∂f<br />
∂z |P0 k<br />
Let u be any vector on the xy−plane, and z = f(x, y) define the surface<br />
S. The directional derivative of f in the direction u at the point P0 on S:(u is<br />
a unit vector here)<br />
∂f<br />
∂s |P0 = ∇F |P0 · u<br />
[It represents the rate of change of f along the ”curve,” f(x, y) onS where (x, y)<br />
runs along u]<br />
Note: The directional derivative is a maximum when the angle Θ between ∇f|p0<br />
and u is 0, because:<br />
∇f|p0 · u = |∇f||p0 ·|u| cos Θ<br />
Figure 1<br />
ie. when ∇f|P0 and u are parallel.<br />
So, directional derivative is maximum in the direction of ∇f|P0 of f at P0<br />
The directional derivative of z = f(x, y) atP0(x0,y0) onS in the direction Θ<br />
(Θ in radians) means in the direction of the unit vector u =(cosθ)i +(sinΘ)j
10 The ABC’s of Calculus<br />
Example 9<br />
Find ∂f<br />
∂s |P0<br />
Here f(x, y) =x 2 + xy + y 2<br />
z = x 2 + xy + y 2 , P0 =(3, 1), Θ= π<br />
3<br />
∇f = ∂f<br />
∂x i + ∂f<br />
∂y j<br />
= (2x + y)i +(x +2y)j<br />
∇f|P0 = ∇f| (3,1)<br />
= (2· 3+1)i +(3+2· 1)j<br />
= 7i +5j<br />
Also u = cosΘi +sinΘj<br />
= cos π<br />
3 i +sin π<br />
3 j<br />
= 1<br />
2 i<br />
√<br />
3<br />
+<br />
2 j<br />
Therefore ∂f<br />
∂s |P0 = ∇f|P0 · u<br />
= (7i +5j) · ( 1<br />
2 i +<br />
= 7<br />
2 + 5√3 2<br />
Example 10 z =2x 2 +3xy − y 2 at (1, 1) toward (2, 1).<br />
√ 3<br />
2 j)<br />
Here f(x, y) =2x 2 +3xy − y 2 , ∇f =(4x +3y, 3x − 2y) or ∇f| (1,−1) =(4· 1+<br />
3(−1), 3(1) − 2(−1)) = (1, 5)<br />
To find u we need to find a vector from (1, 1) to (2, 1). This is given by v =<br />
√(2, 1) − (1, −1) √ = (1, 2). Tomakevinto a unit vector we divide v by its length:<br />
12 +22 = 5.<br />
Therefore u = v (1, 2)<br />
= √ =(<br />
|v| 5 1<br />
√ ,<br />
5 2<br />
√ )<br />
5<br />
∂f<br />
∂s | (1,−1) = ∇f| (1,−1) · u<br />
= (1, 5) · ( 1<br />
√ ,<br />
5 2 √ )<br />
5<br />
=<br />
1<br />
√ +2<br />
5 √ 5<br />
√<br />
5<br />
=<br />
5 +2√5 = 11√<br />
5<br />
5
Functions and their properties 11<br />
What is its maximum value? When:<br />
u = ∇f<br />
|∇f| |P0 : ∂f<br />
∂s | (1,−1) = 1 2 +5 2 = √ 26<br />
1.3 Maxima and Minima Subject to Constraints<br />
To look for maximum and minimun of functions of two variables, z = f(x, y)<br />
we set:<br />
∂f<br />
∂f<br />
=0 and<br />
∂x ∂y =0<br />
and look for those points P (x, y) atwhichbothof these hold. If, at such P ,<br />
( ∂2f ∂x∂y )2 − ( ∂2f ∂x2 )(∂2 f<br />
) < 0<br />
∂y2 and<br />
1) ∂2f ∂x2 + ∂2f ∂y2 > 0<br />
OR:<br />
at p ⇒ relative minimum at p<br />
2) ∂2f ∂x2 + ∂2f ∂y2 < 0 at p ⇒ relative maximum at p<br />
3) ∂2 f<br />
∂x 2 + ∂2 f<br />
∂y 2 =0 atp ⇒ Undetermined: need more info.<br />
NOTE : The boundary of the domain of f must always be checked separately for<br />
relative maximum or minimum values.<br />
Example 11 z =2x +4y − x 2 − y 2 − 3 (= f(x, y))<br />
(Domain of f is the whole plane R 2 ), (so boundary of domain is at ∞)<br />
So we need:<br />
(1, 2) is only possible candidate<br />
∂z 0= ∂x =2− 2x ⇒ x =1<br />
=4− 2y ⇒ y =2<br />
0= ∂z<br />
∂y<br />
so x =1, y =2. This means that (1, 2) is the only critical point inside dom(f).<br />
At P0:<br />
[( ∂2 f<br />
∂x∂y )2 +( ∂2 f<br />
∂x 2 ) · (∂2 f<br />
∂y 2 )](1, 2) = (0)2 − (−2)(−2) = −4 < 0 O.K.<br />
So go to the next step, At P0:<br />
[( ∂2f ∂x2 )+(∂2 f<br />
)](1, 2) = (−2) + (−2) = −4 < 0<br />
∂y2 By this second derivative test, therefore relative maximum at (1, 2).
12 The ABC’s of Calculus<br />
Example 12 Find positive numbers x, y, z such that x + y + z =12and xy 2 z 3<br />
is a maximum.<br />
So, here we want to maximize the function xy 2 z 3 subject to the constraint x +<br />
y + z =12.<br />
But then z =12−x−y and so we wnat to maximize the function of two variables<br />
f(x, y) =xy 2 (12 − x − y) 3 over those x>0,y >0.<br />
Now,<br />
0= ∂f<br />
∂x = y2 (12 − x − y) 3 + xy 2 · 3(12 − x − y) 2 (−1)<br />
= (12−x−y) 2 y 2 [(12 − x − y)+x(−3)]<br />
= (12−x−y) 2 y 2 next<br />
(12 − 4x − y)<br />
0= ∂f<br />
∂y = 2xy(12 − x − y)3 + xy 2 · 3(12 − x − y) 2 (−1)<br />
= xy(12 − x − y) 2 [2(12 − x − y) − 3y]<br />
= xy(12 − x − y) 2 (24 − 2x − 5y)<br />
so we need to find those x, y for which,<br />
and<br />
(12 − x − y) 2 y 2 (12 − 4x − y) =0<br />
(12 − x − y) 2 xy(24 − 2x − 5y) =0<br />
there are many possiblilities – Each one must be considered in turn.<br />
1) One possibility is that (12 − x − y) 2 =0⇒ x + y =12<br />
In this case, since x+y+z =12and x+y =12⇒ z =0and so xy 2 z 3 =0<br />
is a ”minimum” (as x, y, z are all assumed positive).<br />
2) Another possibility is tah y =0but again this gives a minimum.<br />
3) Yet another possibility is that 12 − 4x − y =0Now from the second equation<br />
there are two sub-cases (Why?):<br />
a) x =0<br />
b) 24 − 2x − 5y =0<br />
a) x =0As before this gives xy2z 3 =0, a minimum.<br />
b) 24 − 2x − 5y =0and 12 − 4x − y =0have the common solution<br />
(x, y) =(2, 4).<br />
So the only possible critical point which can give a maximum is: (x, y) =(2, 4)<br />
For (x, y) =(2, 4), z=12− x − y =12− 2 − 4=6.
Functions and their properties 13<br />
<br />
Now at (2, 4) =<br />
[( ∂2 f<br />
∂x∂y )2 +( ∂2 f<br />
∂x 2 ) · ( ∂2 f<br />
∂y 2 )] < 0<br />
And calculations show that the above is a maximum.<br />
Maximum value<br />
xy 2 z 3 = (2)(4) 2 (6) 3 =32· 6 3<br />
Remarks: In many of these problems we are faced with solving systems of<br />
equations of the form:-<br />
ABC =0<br />
Where A, B, C are functions of several variables.<br />
Then the cases are:<br />
A = 0 B = 0 C =0<br />
A = 0 B =0 C = 0<br />
A = 0 B =0 C =0<br />
A =0 B = 0 C =0<br />
A =0 B =0 C = 0<br />
A =0 B =0 C =0<br />
A =0 B = 0 C = 0<br />
and each one must be treated separately.<br />
1.4 Constrained Maxima and Minima: Method<br />
of Lagrange<br />
This techmique is used for finding the maximum and minimum of functions<br />
z = f(x, y) subject to some additional constraint, like:<br />
The idea is then:<br />
1) Form the function<br />
h(x, y) = f(x, y)<br />
<br />
Origional<br />
φ(x, y) =0<br />
+ λ<br />
<br />
Lagrange multiplierf(x,y)<br />
2) Solve ∂h ∂h<br />
∂x =0, ∂x = 0 for all possible x, y and λ.<br />
constraint<br />
<br />
φ(x, y)
14 The ABC’s of Calculus<br />
3) Continue as in previous section.<br />
Example 13 Find area of largest rectangle which can be inscribed in a semicircle<br />
of radius a with base on its diameter.<br />
Denote rectangle’s sides by: 2x, y<br />
Figure 2<br />
We want to maximize the area of the rectangle, i.e. A(x, y) =sxy subject to<br />
constraint that ”rectangle is inscribed in semicircle”. This means that x 2 + y 2 =<br />
a 2 (see diagram) or, φ(x, y) =x 2 + y 2 − a 2 =0.<br />
UseLagrangemultipliers:So let h(x, y) =2xy + λ(x 2 + y 2 − a 2 )<br />
Then:<br />
Also<br />
∂h<br />
=0⇒ 2y + λ(2x) =0 ie. λx + y =0<br />
∂x<br />
∂h<br />
=0⇒ 2x + λ(2y) =0 ie. λy + x =0<br />
∂y<br />
From the first of these two we get λ = −y<br />
x (if x = 0).<br />
(an assumption which is good, since if x =0⇒ no area)<br />
Substitute this value of λ = −y<br />
x<br />
−y 2<br />
into the second equation, to find:<br />
x + x =0 or x2 = y 2 ⇒ x = ±y<br />
Since we can assume x>0,y >0, thismeansx = y.<br />
Now:<br />
x 2 + y 2<br />
or 2x 2 =7a 2<br />
or x =<br />
= a 2<br />
⇒ x 2 + x 2 = a 2<br />
a<br />
√ 2<br />
Thus x = a √ 2 ,y = a √ 2 is an extreme point and:<br />
Area = 2xy<br />
= 2· a √ ·<br />
2 a √<br />
2<br />
= a 2
Functions and their properties 15<br />
Example 14 Find the shortest and largest distance from the origin to the curve<br />
x 2 + xy + y 2 =16and give a geometric iterpretation.<br />
HINT: find the maximum of x 2 + y 2 .<br />
CONSTRAINT: x 2 + xy + y 2 =16<br />
[h(x, y) =x 2 + y 2 + λ(x 2 + xy + y 2 − 16)]<br />
∂h<br />
∂x<br />
= 2x + λ(2x + y) =0 1<br />
∂h<br />
∂y<br />
= 2x + λ(x +2y) =0 2<br />
∂h<br />
∂λ = x2 + xy + y 2 − 16 = 0 3<br />
Now: if 2x + y =0,thenx =0too! but then y = −2x =0too! so x =0, y =0<br />
is only possibility here for 1 .<br />
NOTE: x =0, y =0satisfied 1 , 2 ,butnot 3 so 2x + y = 0can solve for λ<br />
λ = −( 2x<br />
2x+y ) from 1<br />
so we feed this value of λ into 2<br />
2y +(− 2x<br />
)(x +2y)<br />
2x + y<br />
= 0<br />
2y(2x + y) − 2x(x +2y)<br />
2x + y<br />
= 0<br />
so y(2x + y) − x(x +2y) = 0<br />
y 2 − x 2 = 0<br />
⇒ y = ±x<br />
finally set y = ±x into constraint equation x 2 + xy + y 2 =16.<br />
Which leaves two cases: y = x and y = −x<br />
case 1: y = x ⇒ x 2 + x 2 + x 2 =16or 3x 4 =16<br />
x = ± 4 √ 3 But y = x ⇒ y = ± 4 √ 3 too!
oy-<br />
16 The ABC’s of Calculus<br />
case2: y = −x ⇒ x 2 − x 2 + x 2 =16or x 2 =16.<br />
So we get four critical points:<br />
x 2 + y 2<br />
32<br />
3<br />
32<br />
3<br />
32<br />
32<br />
x 2 + y 2<br />
<br />
32<br />
3<br />
32<br />
3<br />
√<br />
√<br />
32<br />
32<br />
x = ±4 But y = −x ⇒ y = ±4 too!<br />
x = 4 √ 3<br />
check critical points for max/min.<br />
, y = 4 √ 3<br />
x = − 4 √<br />
3<br />
, y = − 4 √<br />
3<br />
x =4 , y = −4<br />
x = −4 , y =4<br />
Note that maximum is at (4, −4) and (−4, 4) and the value at this point is √ 32<br />
Note that minimum is at (± 4 √ 3 , ± 4 √ 3 ) and the value here is<br />
1.5 Double and Iterated Integrals<br />
32<br />
3<br />
Let domain f = R and write z = f(x, y) continuous over a finite region R of<br />
the x, y plane. We divide R into n subregions area ∆A1, ∆A2, ......, ∆An in any<br />
fashion what so ever.<br />
Figure 4<br />
Next we pick a point (x1,y1), (x2,y2), ......, (xn,yn) in each one of these subregions<br />
and form the sum:<br />
n<br />
f(xi,yi)∆Ai = f(x1,y1)∆A1 + ...... + f(xn,yn)∆An<br />
i=1<br />
Think about f(xi,yi)∆Ai : This is the signed volume of a parallelepipeds of<br />
base area ∆Ai and height f(xi,yi).