16 The Quadratic Reciprocity Law - Caltech Mathematics Department

16 The Quadratic Reciprocity Law
Fix an odd prime p. If q is another odd prime, a fundamental question, as
q
we saw in the previous section, is to know the sign , i.e., whether or
p
not q is a square mod p. This is a very hard thing to know in general. But
q
Gauss noticed something remarkable, namely that knowing is equivalent
p
p
to knowing ;they need not be equal however. He found the precise law
q
which governs this relationship, called the Quadratic Reciprocity Law. Gauss
was very proud of ths result and gave several proofs. We will give one of
his proofs, which incidentally introduces a very basic, ubiquitous sum in
Mathematics called the Gauss sum. We will also give an alternate proof,
which is in some sendse more clever than the first, due to Eisenstein.
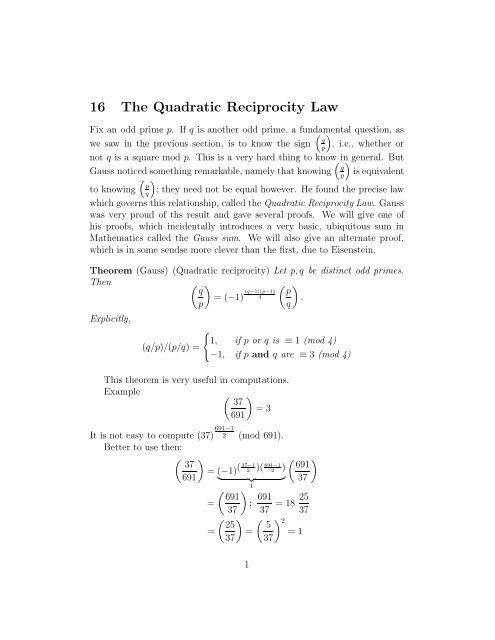
Theorem (Gauss) (Quadratic reciprocity) Let p, q be distinct odd primes.
Then
q
=(−1)
p
(q−1)(p−1)
Explicitly,
p
4 .
q
(q/p)/(p/q) =
1,
−1,
if p or q is ≡ 1 (mod 4)
if p and q are ≡ 3 (mod 4)
This theorem is very useful in computations.
Example
37
=3
691
It is not easy to compute (37) 691−1
2 (mod 691).
Better to use then:
37
691
37−1
=(−1) ( 2 )( 691−1
2 ) 691
37
1
691
= ;
37
691 25
=18
37 37
2 25 5
= = =1
37 37
1

Proof#1oftheorem: p, q odd primes, p = q. Put
ξ = e 2πi
q ∈ C
Then
ξ q =1, but ξ m =1ifm

This allows us to do “congruence arithmetic” mod p in R.
), Gauss introduced the following “Gauss Sum”:
To study ( q
p
Sq =
a mod q
a
ξ
q
a .
Clearly, Sq ∈ R.
Aside (Not part of proof of Quad. Recip., but interesting)
So if
Sq =
2
q−1
a=1
a
q
−1
=1,Sq =
q
ξ a +
2
q−1
a=1
−a
ξ
q
−a
( −1
q )( a
q ) ¯ ξa
a
(ξ
q
a + ¯ ξa ) ∈ R ∩ R
and if
−1
= −1, Sq∈ R ∩ iR
q
pure read or im.
Lemma 1:
Proof of Lemma 1:
S 2 q =
a mod q
c mod q
S 2 q =(−1) q−1
2 q
a
ξ
q
a
a mod q
b mod q
b
ξ
q
b
=
a b
ξ
q q
a mod q b mod q
a ξ b
=
ab
ξ
q
a+b
=
ξ c
a(c − a)
q
3

So
where a ′ a ≡ 1(modq).
where
S 2 q =
c mod q
=
But −a 2 (1 − a ′ c)
f(c) =?
c ≡ 0(modq):
q
c mod q
c
ξ
2 ac − a
a mod q
2 ′ −a (1 − a c)
c
ξ
a mod q
−1
=
q
(−1) q−1
2
⇒ S 2 q =(−1) q−1
2
f(c) =
a mod q
f(0) =
q
q
2 a
q
=1 as a≡0 modq
c mod q
′ 1 − a c
q
a mod q
a≡0 (modq)
⇒ f(0) = q − 1
c ≡ 0(modq): Note that, in this case, the set
ξ c f(c),
,
a ≡ 0modq
1
q
{1 − a ′ c|a mod q, a ≡ 0modq}
′ 1 − a c
runs over elements of Z/q −{1} exactly once. Indeed, given any b ∈ Z/q,
b ≡ 1(modq), we can solve (a ′ + b ≡ 1(modq), and the solution is unique.
Therefore,
f(c) =
b mod q
b≡1 (modq)
4
b
.
q
q

We proved earlier that
so
when c ≡ 0(modq).
Consequently
S 2 q =(−1) q−1
2
Claim:
c mod q ξc =0.
Proof of claim:
c mod q
ξ c =
⇒ (1 − ξ)
=0
Proof 2 of claim:
By claim,
c mod q
S 2 q
This proves Lemma 1.
Lemma 1: S 2 q
b mod q
f(c) =
⎡
(c−1) mod q
c mod q
=(−1) q−1
2 q
b
=0
q
1
= −1,
q
⎢
⎣(q − 1) + (−1)
ξ c =
c mod q
ξ c =0⇒
c mod q
ξ c =1+ξ + ···+ ξ q−1 =
=0sinceξ q =1.
c mod q
c≡0 modq
⎤
ξ c⎥
⎦
ξ c+1 = ξ
c mod q
ξ c
ξ c = 0 as claimed.
1 − ξq
1 − ξ
q−1
=(−1) 2 (q − 1) + (−1)(0 − 1)
+1
=(−1) q−1
2 q.
5

Lemma 2: S p−1
q
≡ ( p
q )(modp)
(This happens in R mod p)
Proof of Lemma 2:
S p
a
q =
ξ
q
a mod q
a
p =
p a
ξ
q
ap + pw, w ∈ R.
( a
p )q =( a
p
a mod q
a
) because ( )=±1 andpis odd
q
In other words,
S p q ≡
a
ξ
q
ap (mod p).
a mod q
Since p = q, p is invertible mod q, andthemapa ↦→ ap is a permutation of
Z/q, alsoap ≡ 0(modq) iffa ≡ 0(modq). so the sum over a mod q can be
replaced with the sume over ap mod q. Write b for ap mod q. Then
a ≡ bp ′ (mod q), where pp ′ ≡ 1modq).
⇒ S p q ≡
b mod q
bp ′
But
′
′
bp b p
=
.
q q q
Since p ′ p ≡ 1(modq),
′
′
p p 1
p
= =1⇒ =
q q q
q
So
′ bp b p
= .
q q q
So (∗) gives
S p
p
b
q ≡
ξ
q q
b mod q
b
(mod p)
Sq
6
q
ξ b (mod p) (*)
p
q

This is justified because
⇒ S p−1
q ≡
p
q
Sq ≡ 0(modp),
(mod p)
which follows from lemma 1.
ProofofTheorem: Compute Sp−1 in 2 different ways. On the one hand,
by lemma 1,
i.e.,
S p−1 =(S 2 ) p−1
2 =
≡
Euler
(−1) q−1
2 q
(−1) q−1
2 q
p−1
2
(mod p
p
⇒ S p−1 q−1
2 −1
q
≡
(mod p,
p 2
S p−1 p−1
≡ (−1) ( 2 )( q−1
2 )
On the other hand, by lemma 2,
S p−1 ≡
So, putting them together we get
p
q
p
q
q
p
(mod p).
p−1
=(−1) ( 2 )( q−1
2 )
(mod p).
q
.
p
Last time, gave a proof of Quadratic Reciprocity law. More precisely we
proved:
Theorem (Gauss) Let p, q be distinct, odd primes. Then
p
q
p−1
(−1) ( 2 )( q−1
2 )
7
q
p
.

Example:
Check if 29 is a square mod 43:
29 and 43 are distinct odd primes, so by
=1. byQRL,
definition 29 ≡ (mod 43) iff 29
43
29
=(−1)
43
28(42)
43 43
4 =
29 29
14 2 7
= =
29 29 29
2
= −1 as29≡5(mod8) 29
29 7
= − = −(−1)
43 29 QRL
6(28)
29
4
7
29 1
= − = − = −1
7 7
So 29 ≡ (mod 43).
Remark: QRL tells you a way to know
1. whether q is a square mod p or not. But when it is a square, it gives
no procedure to find the square root.
2. One can use QRL to check whether a number is a prime, similar to the
way one uses Fermat’s little theorem. For example, one can show that
m = 1729 is not a prime by looking at
y def ≡ 11 864 (mod 1729)
Note: 864 = 1729−1
.So,ifmis a prime, y ≡ ( 2
11
1729 )(modm).
Since 1729 ≡ 1(mod4),byQRL,
11 1729
= =
1729 11
2
= −1
11
as 11 ≡ 3 (mod 8). on the other hand, one can check using PARI, or
by successively squaring mod m = 1729, that
11 864 ≡ 1(modm).
8

(This is part of a homework problem.) Get a contradiction! So the only
possibility is that 1729 is not a prime (which is easy to verify directly
as 1729 = 13 · 133 = 13 · 7 · 17). But this method is helpful, when it
works, for larger numbers.
A histoical remark: G.H.Hardy went to see Ramanujan, when the latter
was dying of TB in England. Then Ramanujan asked Hardy if the number
of the taxicab Hardy came in was an interesting number. Hardy said “No,
not interesting, just 1729”. Ramanujan replied immediately, saying, “On the
contrary, the number is interesting because it is the first number which can
be written as a sum of 2 cubes in two different ways”. (Indeed we have
1729 = 10 3 +9 3 =12 3 +1 3 .
)
A second proof of quadratic recip. (Eisenstein) (Eisenstein’s trignometric
lemma)
Lemma: Letnbea positive, odd integer. Then
sin nx
sin x
n−1
=(−4) 2
(n−1)
2
j=1
sin 2 2 2πj
x − sin
n
Proof: Up to us. Hint: treat as a polynomial in sin x:
Example:
n =3
sin 3x sin(2x + x)
LHS = =
sin x sin x
= sin 2x cos x +cos2xsin x
sin x
= 2sinxcos2 x +(1−2sin 2 x)sinx
sin x
=2(1− sin 2 x)+(1− 2sin 2 x)=3− 4sin 2 x
⎛
RHS = −4(sin 2 ⎜
x − ⎜
2π ⎟
⎝
sin ⎟
3 ⎠
=3− 4sin 2 x.
√ 3/2
9
⎞
2
= −4 sin 2 x − 3
4

Sketch of proof of lemma: Use induction on n to show that
sin nx
sin x = fn(sin 2 x),
where fn is a polynomial in sin 2 x of degree n−1
2 .
(f0(t) =1,f3(t) =3− 4t,...)
On the other hand, the RHS of lemma is also of the form gn(sin 2 x), where
gn is the explicitly given polynomial in sin 2 x of degree n−1
2 .
So it suffices to show that fn and gn have the same roots and that the
leading coefficient of fn is (−4) n−1
2 . So when we use induction on n, check
that the leading coefficient is (−4) (n−1)
2 and that its roots are
2 2πj
sin
n − 1
n 1 ≤ j ≤ .
2
Alternatively, check the constant coefficient by checking at x → 0.
Recall Gauss’ lemma:
q
=
p
es(q)
s∈S
where S = {1, 2,..., p−1
2 } and ex(q) ∈{±1} defined by
Applying sin( 2π),
we get
p
qs = es(q)s ′ , with s ′ ∈ S.
2πqs 2πes(q)s
sin =sin
p
′
p
′ 2πs
= es(q)sin
p
since sin is an odd function. So
2πqs
sin p
es(q) =
2πs ′
sin
10
p

By Gauss’ lemma,
q
=
p
2πqs
sin p
2πs ′
s∈S sin p
s∈S
=
sin
2πqs
p
s∈S sin
.
2πs ′
Note the map S ↦→ S ′ is a permutation of S. So,
′ 2πs
sin =
p
2πs
sin
p
s∈S
⇒
(p−1)
q
2
=
p
i=1
s∈S
p
sin 2πiq
p
sin 2πi
p
Applying Eisenstein’s trig. lemma with n = q and sub. in (3), we get
q
p−1
=(−4) ( 2 )(
p
q−1
(p−1)
2
2 )
i−1
(q−1)
2
i−1
sin 2
2πi
− sin
p
2
2πj
p
Can get everything we need from this without computing the sines:
Reversing the roles of p and q, weget
p
=(−4)
q
q−1
2
p−1
2
2
q−1
i−1 i−1
Comparing (3) and (4), we see that
q
p
sin 2
=(−1) (p−1) (q−1)
2 2
11
2πj
− sin
p
2
2πi
p
p
q
(1)