- Page 1 and 2: NON-EQUILIBRIUM STATISTICAL PHYSICS
- Page 3 and 4: ISBN 978-0-7354-0887-6 ISSN 0094-24
- Page 5 and 6: To learn more about AIP Conference
- Page 7 and 8: Editors Pedro L. Garrido Joaquín M
- Page 9 and 10: On the approach to thermal equilibr
- Page 11 and 12: Analytical study of hysteresis in t
- Page 13 and 14: Fluctuations of the dissipated ener
- Page 15 and 16: EDITORS' PREFACE This volume origin
- Page 17 and 18: Nonequilibrium Statistical Physics
- Page 19 and 20: The third issue is really, if you w
- Page 21 and 22: is which domain you keep in your mi
- Page 23 and 24: Still, if I give you a number, pick
- Page 25 and 26: sions from kinetic theory, even at
- Page 27 and 28: the forces between them, then we wo
- Page 29 and 30: • JLL: I think in general. . . Wh
- Page 31 and 32: something some interactions that ap
- Page 33 and 34: equations of motion. Working just o
- Page 35 and 36: you have some other open problems w
- Page 37 and 38: Three lectures: NEMD, SPAM, and sho
- Page 39 and 40: E = 1/2 0.3 Fermi-Pasta-Ulam 0.2 0.
- Page 41 and 42: Consider the simplest interesting c
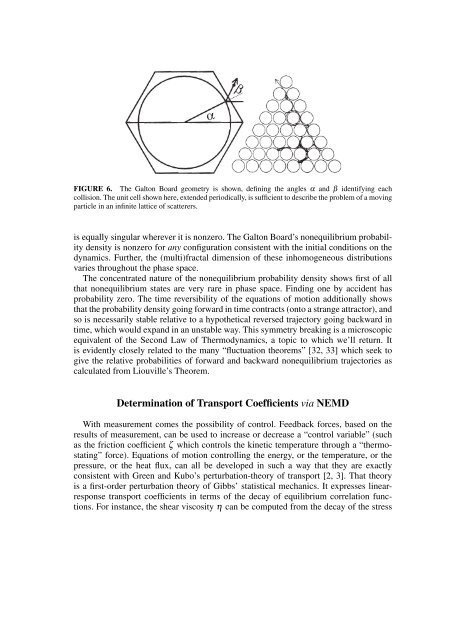
- Page 43 and 44: Nosé-Hoover mechanics opens up the
- Page 45: FIGURE 5. A series of 200,000 Galto
- Page 49 and 50: FIGURE 8. Rayleigh-Bénard problem,
- Page 51 and 52: SPAM Algorithms and the Continuity
- Page 53 and 54: FIGURE 10. Contours of average dens
- Page 55 and 56: FIGURE 12. Water Column collapse fo
- Page 57 and 58: FIGURE 14. Stationary shockwave in
- Page 59 and 60: FIGURE 16. Snapshots near the begin
- Page 61 and 62: FIGURE 17. Shock Thermal and Mechan
- Page 63 and 64: Solving the time-dependent continuu
- Page 65 and 66: time = 2 time = 4 time = 6 time = 1
- Page 67 and 68: time = 2 time = 4 time = 6 time = 1
- Page 69 and 70: Holland, Amsterdam, 1986, pp. 43-65
- Page 71 and 72: λ0 0000000 1111111 0000000 1111111
- Page 73 and 74: The Langevin equation for the overd
- Page 75 and 76: for the Langevin equation (4). The
- Page 77 and 78: The first term on the right hand si
- Page 79 and 80: As an important application, based
- Page 81 and 82: FIGURE 6. Sketch of protein unfoldi
- Page 83 and 84: Detailed fluctuation theorem In a N
- Page 85 and 86: 1 . 5 1 . 0 0 . 5 v io la t io n in
- Page 87 and 88: At first sight, one might not have
- Page 89 and 90: of internal states of the machine o
- Page 91 and 92: Hydrodynamics from dynamical non-eq
- Page 93 and 94: conclusions. THEORETICAL BACKGROUND
- Page 95 and 96: = exp[−β(H0(Γ) + k 2 ( ˆF(r)
- Page 97 and 98:
y the Blue Moon ensemble (see Refs.
- Page 99 and 100:
ent cells as , b three servoir n x
- Page 101 and 102:
(a) (b) FIGURE FIG. 5. 3. Setting L
- Page 103 and 104:
simulation box along the χ-th Cart
- Page 105 and 106:
!"!"!#$%&'# !"!"!(#$%&'# FIGURE 6.
- Page 107 and 108:
produce the phenomenon of pushing u
- Page 109 and 110:
11. R. Kubo, J. Phys. Soc. Jpn. 12,
- Page 111 and 112:
According to the equipartition law,
- Page 113 and 114:
FIGURE 1. Control parameter and con
- Page 115 and 116:
FIGURE 2. The bead in the optical t
- Page 117 and 118:
The unfolding of the hairpin is rev
- Page 119 and 120:
FIGURE 5. The Crooks fluctuation re
- Page 121 and 122:
FIGURE 6. FDC versus FEC. (a) Exper
- Page 123 and 124:
FIGURE 8. The generalized Crooks fl
- Page 125 and 126:
Universality in equilibrium and awa
- Page 127 and 128:
UNIVERSALITY IN NON-EQUILIBRIUM Let
- Page 129 and 130:
From this perspective, does it make
- Page 131 and 132:
(a) (b) (c) (d) σ=2.30 σ=2.38 σ=
- Page 133 and 134:
to the coarse-graining process. How
- Page 135 and 136:
ford, 1990; G. Parisi, Statistical
- Page 137 and 138:
Fourier law, phase transitions, and
- Page 139 and 140:
FIGURE 1. a β (m) is flat in [−m
- Page 141 and 142:
which shows that in the stationary
- Page 143 and 144:
FREE ENERGY FUNCTIONALS AND LYAPUNO
- Page 145 and 146:
If we use instead the Ginzburg-Land
- Page 147 and 148:
REFERENCES 1. A. De Masi, E. Presut
- Page 149 and 150:
scale. The theory, mesoscopic non-e
- Page 151 and 152:
where L(γ,P(γ)) is an Onsager coe
- Page 153 and 154:
To illustrate explicitly the influe
- Page 155 and 156:
which can also be expressed as J =
- Page 157 and 158:
CONCLUSIONS The classical way to st
- Page 159 and 160:
Noise-induced transitions vs. noise
- Page 161 and 162:
Alternatively, for fixed µ > 0 one
- Page 163 and 164:
expressed in terms of the variable
- Page 165 and 166:
the dynamics: for larger noise inte
- Page 167 and 168:
m(t) m(t) m(t) m(t) m(t) 4 2 0 -2 -
- Page 169 and 170:
On the approach to thermal equilibr
- Page 171 and 172:
We assume that there is one dominan
- Page 173 and 174:
we thus have that |ψt〉〈ψt| =
- Page 175 and 176:
To better appreciate the significan
- Page 177 and 178:
with a multitude of systems, all wi
- Page 179 and 180:
hypothesis [2], in which the thermo
- Page 181 and 182:
NON-EQUILIBRIUM THERMODYNAMIC POTEN
- Page 183 and 184:
and composition, the question about
- Page 185 and 186:
3. D. Y. Tzou, Macro-to-microscale
- Page 187 and 188:
Dynamical processes defined on netw
- Page 189 and 190:
For the marginal case s = 2, where
- Page 191 and 192:
may occur, which cause power-law dy
- Page 193 and 194:
Stationary points approach to therm
- Page 195 and 196:
is an insignificant restriction, si
- Page 197 and 198:
FIGURE 2. Sketch of stationary poin
- Page 199 and 200:
dimensions and height comparable to
- Page 201 and 202:
FIGURE 2. Sequence of top view conf
- Page 203 and 204:
ACKNOWLEDGMENTS We would like to th
- Page 205 and 206:
Local properties at equilibrium can
- Page 207 and 208:
✻ β −1 µ0 µ1 µ2 µ3 J compl
- Page 209 and 210:
On the role of Galilean invariance
- Page 211 and 212:
with δ ± λ j ≡ 2νa (h j±1
- Page 213 and 214:
ACKNOWLEDGMENTS HSW acknowledges fi
- Page 215 and 216:
where 〈...〉eq is an average ove
- Page 217 and 218:
the Heisenberg chain is incorporate
- Page 219 and 220:
Computing LDFs from scratch, starti
- Page 221 and 222:
which expresses the locally-Gaussia
- Page 223 and 224:
allowed because of (i). Hence for t
- Page 225 and 226:
G(J x ,J y ) 0 -1 -2 -3 -4 -5 -6 -4
- Page 227 and 228:
dependent optimal profiles (probabl
- Page 229 and 230:
FIGURE 1. a) SNR vs γ, for εc −
- Page 231 and 232:
can set up a reference frame S ′
- Page 233 and 234:
A Φ(x) =ξ− B Φ(x) =ξ+ Φ(x) =
- Page 235 and 236:
Dynamical behavior of heat conducti
- Page 237 and 238:
Fast transients in mesoscopic syste
- Page 239 and 240:
ACKNOWLEDGMENTS This research was p
- Page 241 and 242:
FIGURE 1. (a) Three types of decay
- Page 243 and 244:
To study the heat flow, we write th
- Page 245 and 246:
the time-reversal invariance of the
- Page 247 and 248:
FIGURE 1. Averaged stochastic traje
- Page 249 and 250:
Strong ratchet effects for heteroge
- Page 251 and 252:
Stochastic protein production and t
- Page 253 and 254:
Creating the conditions of anomalou
- Page 255 and 256:
Cluster size distribution in Gaussi
- Page 257 and 258:
A non-equilibrium potential functio
- Page 259 and 260:
Quasi-stationary states and a class
- Page 261 and 262:
Fluctuation relations and fluctuati
- Page 263 and 264:
Why are so many networks disassorta
- Page 265 and 266:
Analytical study of hysteresis in t
- Page 267 and 268:
Queues on narrow roads and in airpl
- Page 269 and 270:
Fluctuations out of equilibrium V.V
- Page 271 and 272:
Long-range interacting systems and
- Page 273 and 274:
Irreversibility of the renormalizat
- Page 275 and 276:
Classical systems: moments, continu
- Page 277:
ABSTRACTS OF SELECTED CONTRIBUTIONS
- Page 280 and 281:
Nonlinear Boltzmann equation for th
- Page 282 and 283:
The Liouville equation and BBGKY hi
- Page 284 and 285:
Experimental densities of binary mi
- Page 286 and 287:
New insights in a 2-D hard disk sys
- Page 288 and 289:
Velocity-velocity correlation funct
- Page 290 and 291:
Analysis of ship maneuvering data f
- Page 292 and 293:
Thermally activated escape far from
- Page 294 and 295:
Space-time phase transitions in the
- Page 296 and 297:
Fluctuations of the dissipated ener
- Page 298 and 299:
Breeding gravitational lenses J. Li
- Page 300 and 301:
A formula on the pressure for a set
- Page 302 and 303:
Noninteracting classical spins in a
- Page 304 and 305:
Current fluctuations in a two dimen
- Page 306 and 307:
Violation of fluctuation-dissipatio
- Page 308 and 309:
Nonequilibrium thermodynamics of si
- Page 310 and 311:
The non-equilibrium and energetic c
- Page 312 and 313:
Dynamical systems approach to the s
- Page 315 and 316:
Akiyama, Ryo Japan Álvarez-Estrada
- Page 317 and 318:
A Akiyama, Ryo 261, 295 Albano, Eze
- Page 319:
P Pérez-Espigares, C. 202, 268, 28