Laue indexing manual.pdf

Introduction
This program is designed for indexing Laue patterns, finding of the
orientation of monocrystal. Unlike the other software, which solve this task by
calculation the angles between the planes, this program uses the classical principle
of Stereographic projection calculation, finding the points of projection, which
align with arcs (meredians), analytical rotation of crystal to coincide the
Stereographic projection with Standard projection.
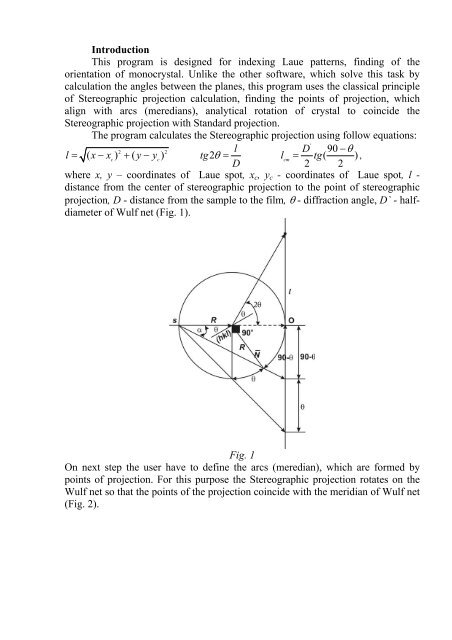
The program calculates the Stereographic projection using follow equations:
'
2 2
l
D 90
l ( xx ) ( y y ) tg2
l tg(
) ,
c c
D
ст
2 2
where x, y – coordinates of Laue spot, xc, yc - coordinates of Laue spot, l -
distance from the center of stereographic projection to the point of stereographic
projection, D - distance from the sample to the film, - diffraction angle, D` - halfdiameter
of Wulf net (Fig. 1).
Fig. 1
On next step the user have to define the arcs (meredian), which are formed by
points of projection. For this purpose the Stereographic projection rotates on the
Wulf net so that the points of the projection coincide with the meridian of Wulf net
(Fig. 2).

Fig. 2
The rotation of the projection is defined by the equations:

x x
y y
i c
i c
x x i c
y y
i c
yy arctg
xx c
c
' 180
x x2sin l cos
2 2
' 180
y y2sin l sin
2 2
' y y ст c
arctg
x x ст c
' 180
'
x x 2l sin cos
ст ст ст
2 2
' 180
'
y y 2l sin sin
ст ст ст
2 2
yy arctg
xx '
x x l
'
y y l
'
c
c
2sin sin
2 2
2sin cos
2 2
y y ст c
arctg
x x
ст c
' '
x x 2l sin sin
ст ст ст
2 2
' '
y y 2l sin cos
ст ст ст
2 2

x x
y y
i c
i c
x x i c
y y
i c
yy arctg
xx c
c
' 180
x x2sin l cos
2 2
' 180
y y2sin l sin
2 2
' y y ст c
arctg
x x ст c
' 180
'
x x 2l sin cos
ст ст ст
2 2
' 180
'
y y 2l sin sin
ст ст ст
2 2
yy arctg
xx '
x x l
'
y y l
'
c
c
2sin sin
2 2
2sin cos
2 2
y y ст c
arctg
x x
ст c
' '
x x 2l sin sin
ст ст ст
2 2
' '
y y 2l sin cos
ст ст ст
2 2

x x
y y
i c
i c
x x i c
y y
i c
x x i c
y y
i c
' 180
x x2sin l sin
2 2
' 180
y y2sin l cos
2 2
'
180
x x 2l sin sin
ст ст ст
2 2
'
180
y y 2l sin cos
ст ст ст
2 2
' 180
x x2sin l sin
2 2
' 180
y y2sin l cos
2 2
'
180
x x 2l sin sin
ст ст ст
2 2
'
180
y y 2l sin cos
ст ст ст
2 2
' 180
x x2sin l cos
2 2
' 180
y y2sin l sin
2 2
'
180
x x 2l sin cos
ст ст ст
2 2
'
180
y y 2l sin sin
ст ст ст
2 2

' 180
x x2sin l cos
2 2
' 180
y y2sin l sin
x x
i c
2 2
,
y y i c '
180
x x 2l sin cos
ст ст ст
2 2
'
180
y y 2l sin sin
ст ст ст
2 2
where - angle of rotation. The Wulf net coordinates are define by:
cossin x2R 1cos cos
sin
y2R 1cos cos
where - longitude, - latitude, R- radius of Wulf net.
The program also calculates the pole of the pole of planes, which is distant
from the meridian by 90 o (Fig. 2). The user has to define 4 meridians (Fig. 3).
Fig. 3
One should pay attention to the points of the projection, which lies on the intercept
of two meridians (Fig. 3). They are very important because corresponds the planes
with small Miller indices such as (001), (110), (111). To find the orientation of
crystal it is necessary to transform the stereographic projection of the crystal into
the Standard projection. It can be done by rotation of the stereographic projection
,

elative to the Wulf Net and analytical rotation the crystal and the projection by
angle . The points of projection move in parallels by rotation of crystal (Fig. 4).
Fig. 4
For coincidence of the stereographic projection of the crystal with standard
projection it is necessary to choose appropriate Standard projection. The
coordinates of standard projection are defined by:
nhkl
h2 2 2
1cos cos cos 2coscos
cos
a
k h
b a
h k cos cos cos hcos
( cos ) ( c sin )
a b sin
a
2 2 2
( ) ( cos ) 1 cos cos cos 2cos cos cos
where n – normal vector in orthogonal system coordinate, a, b, c, , , - lattice
parameters, h, k, l- Miller indices of plane.
,

ZaZ , , a Z
X arccos( nhkl ( ), Z)
S0 Z, n( hkl) Z
cZZ , , a Z
,
where Z - pole of standard projection, a, c – direction perpendicular to Z, - angle
between vector of reference direction Z and normal to the plane n(hkl).
, Z Z, n( hkl)
0
x
cos
arccos( S0, X)
r Rtg
,
360 , Z Z, n( hkl)
0
y
sin
where R- radius Wulf net, -polar angle.
The best solution for the orientation of monocrystal correspond the situation when
the most important points of the projection (points of meridians intercept and
poles) coincide with Standard projection (Fig. 5).
Fig. 5

Laue indexing manual
1. Required software: ImageJ, Matcad 2001 or later. Before to start it is necessary
to scan X-ray photograph with resolution at least 300dpi.
2. Open ImageJ. Find a file with Laue Photograph File->Open (Fig. 1).
Fig. 1
3. Set a scale for the Lauegramm: Analyze->Set Scale (Fig. 2). Fill in the fild
Distance in Pixels the resolution of the Fhoto (300 dpi), in the field “Known
Distance” set the distance between two points on the Laue pattern in mm (in
our example 25.4 mm, because 1inch is equals 25.4 mm), in the field “Unit of
Length” set the used unit dimension (mm).
4. Press key Point Selection (Fig. 3) and holding pressed key Shift define the Laue
spots on the Photograph by clicking on them left key of Mouse (Fig. 4).

Fig. 2
Fig. 3

Fig. 4
5. After that choose the menu “Analyze->Measure”. It will appear the table with
results (Results) (Fig. 4). Save the results “File->Save as” by name Laue
Spots.dat (We actually need only two columns X,Y. For this purpose the data
could be exported in software Origin lab or others. After that we can export file
again with the same name). Now we have to define the center of Laue pattern,
that is the point in which the incident white X-ray beam intercepts the X-ray
Film. Press key “Elliptical or brash selection” (Fig. 5).
6. Holding left mouse key pressed draw the ellipse on the Laue pattern so that, it
fill the dark spot in the place of X-ray beam intercept (Fig. 6).
7. In order to calculate the coordinates of the center we need to use menu “Image-
>Show Info” (Fig. 7). The coordinates of X-ray pattern defined by following:
x=X+Width/2; x=Y+Height/2, (46.48+10.16/2=51.56; 41.40+10.16/2=46.48).
The obtained results should be saved.
8. Open MathCad and find the file Laue indexing.mcd.

9. Open file with Laue spots coordinates (right mouse click -> Choose file) (Fig.
8).
Fig. 5
Fig. 6

Fig. 7
Fig. 8

10. Define coordinates of the center of Laue Pattern: xc=51.56; yc=46.48
11. Now we have to make the coincidence of our Laue pattern with one of the
Meredian of Wulf net (Fig. 10). This could be done by rotation of
Lauegramm by angle (Fig. 9).
Fig. 9
We have to realize that the rotation by angle >0 rotates the stereographic
projection anti-clockwise, and the rotation by angle

12. Define angle 2=-6 о , which corresponds the rotation of the projection
Fig. 10
Fig. 11

in the opposite side to the rotation by angle (Fig. 12,13). By this the meridian
formed by stereographic projections of pole of planes will become the initial
position (before rotation).
Fig. 12
Fig. 13

13. Next step is to make the coincidence of our Laue pattern with the second of
the Meredian of Wulf net. This requires the rotation of Lauegramm by angle
=-5 (Fig. 14).
The results can be seen on Fig. 15.
Fig. 14
Fig. 15

To draw the second meridian we have to define angle 3=70 о , which is
distant from the Great Circle on the left side from the center of Wulf net by
angle 20 о . Right picture shows for comparison the initial projection before
rotation.
14. Define the angle 4=5 о , which corresponds the rotation of meridian in
opposite side to the rotation by angle (Fig. 16). By this procedure the
meridian will come to the initial position.
Fig. 16
To find the third meridian on the Laue Pattern the stereographic projection
of sample should be rotate by angle =86 о . Fig. 17 shows the result of this
rotation. To draw the meridian define the angle 5=68 о . This meridian is
distant from the Great Circle of Wulf net on the left side from the center of
Wulf net by angle 22 о . Right picture shows for comparison the initial
projection before rotation.
15. Define angle 6=-86 о , which corresponds the rotation of meridian in
opposite side to the rotation by angle (Fig. 18). By this procedure the
meridian will come to the initial position.

Fig. 17
Fig. 18
16. To combine the forth meridian of Wulf net with our stereographic
projection, the later is rotated by angle =92 о . The result of rotation can be
seen on Fig. 19.

Fig. 19
17. To draw the meridian one defines the angle 7=-64 о , which is distant from
the Great Circle of Wulf net on the left side from the center of Wulf net by
angle 26 о . On the right picture is shown the initial projection before rotation.
18. We also have to define angle 8=-92 о , corresponded the rotation of meridian
in opposite side relative to the rotation by angle (Fig. 20). By this
procedure the meridian will come to the initial position. Therefore we have
found 4 meridians, corresponded 4 poles of planes in our sample (Fig.20).
This quantity of poles is quite enough to determine the orientation of crystal.
19. If it is necessary, we can save the results at file meredians.dat (Fig. 21) and
coordinates of points of Wulf net Wulfnet.dat (Fig. 22) for next calculations.
20. On the next step we have to think about which meridian will be used for
analytical rotation of crystal. The best way to choose the meridian, which
contains maximal quantity of stereographic projections and rotate the crystal
analytically кристалл by angle . In this example we define meridian,
which corresponds rotation angle =6 о (2=-6 о ) in relation to horizontal axis
or equator of Wulf net (Fig. 10). To do this define angle (Fig. 23). This

meridian is distant from the Great Circle of Wulf net (on the left side) by
angle =24 о .
21. Then rotate crystal by angle =-24 о (Fig. 24). As a result all point of the
projection (red) must to shift by parallels on the left side (blue) by this angle
(Fig. 25).
Fig. 20
Fig. 21

Fig. 22
Fig. 23
22. Define the lattice parameters of our crystal (a1, b1, c1, , , γ) and pole of
standard projection (Fig. 26). In our example we draw the standard projection
(111) (Fig. 27).

Fig. 24
Fig. 25

Fig. 26
Fig. 27

23. We have two ways to find the appropriate coincidence of the stereographic
projection of the sample (blue) with the points chosen Standard projection
(black):
to rotate the stereographic projection of the sample relative to the standard
projection. The rotation angle is designed as 1 (Fig. 28).
to rotate the standard projection relative to the stereographic projection of
the sample. The rotation angle is designed as (Fig. 29).
Fig. 28
Fig. 29

Fig. 30
24. The results of rotation we can see on Fig. 30. You can see the Stereographic
projection before (red circles), and after (blue circles) crystal rotation by angle ,
poles of planes before (red rhombs), and after (blue rhombs), axes X (cyan) and
Y (brown) before crystal rotation and the result of their movement after the
crystal rotation (black circle). Actually, the best result is when the all points of
the stereographic projections (blue) coincide with all points of standard.
However, this happens very seldom due to experimental errors. The most
important points of standard projections are the points in which intercept several
meridians. They correspond planes in crystal with low Miller indices (hkl) such
as (001), (111), (110). Realizing that, we need to find these points on the
stereographic projection of the sample. Next picture illustrates this principle (Fig.
31). You can see the points of stereographic projection of the sample which
forms meridians (pink).

Fig. 31
25. The Miller indices (hkl) of points of Standard projection with their
coordinates X,Y on Wulf net reported in table A2 (Fig. 32). In order to find the
Miller indices of points of Standard projections it is necessary to click right
mouse key by black point on the graph and select menu “Traces”, then choose the
option “Track data points”, then again click left mouse key on the point you want
to determine Miller indices (Fig. 32). In the small window appear the X,Y
coordinates of these point. To find Miller indices of this point you only need to
find the row in table A2, which has the same coordinates (column 3 corresponds
coordinates X, and column 4 corresponds Y). The columns 0,1,2 correspond the
Miller indices of the point on the Standard Projection.

Fig. 32
26. The obtained results you can save in file “crystal rotation and projection.dat”
(Fig. 33) where the columns designate follow:
S0- х coordinates of Stereographic projection of the sample before the crystal
rotation and after the coinsidence of the projection of crystal with standard
projection
S1- y coordinates of Stereographic projection of the sample before the crystal
rotation and after the coinsidence of the projection of crystal with standard
projection
S2- х coordinates of Stereographic projection of the sample after the crystal
rotation and after the coinsidence of the projection of crystal with standard
projection
S3- y coordinates of Stereographic projection of the sample after the crystal
rotation and after the coinsidence of the projection of crystal with standard
projection
S4- х coordinates of the center of projection
S5- y coordinates of the center of projection
S6- х coordinates of Axis X after rotation of crystal after the coinsidence of the
projection of crystal with standard projection
S7- y coordinates of Axis X after rotation of crystal after the coinsidence of the
projection of crystal with standard projection

S8- х coordinates of Axis Y projection after rotation of crystal after the coinsidence
of the projection of crystal with standard projection
S9- y coordinates of Axis Y projection after rotation of crystal after the coinsidence
of the projection of crystal with standard projection
S10- х coordinates of pole of Axis X projection after rotation of crystal and after the
coinsidence of the projection of crystal with standard projection
S11- y coordinates of pole of Axis X projection after rotation of crystal and after the
coinsidence of the projection of crystal with standard projection
S12- х coordinates of pole of Axis Y projection after rotation of crystal and after the
coinsidence of the projection of crystal with standard projection
S13- y coordinates of pole of Axis Y projection after rotation of crystal and after the
coinsidence of the projection of crystal with standard projection
S14- х coordinates of pole of stereographic projections, which form meredian after
rotation of crystal and after the coinsidence of the projection of crystal with
standard projection
S15- y coordinates of pole of stereographic projections, which form meredian after
rotation of crystal and after the coinsidence of the projection of crystal with
standard projection
S16-х coordinates of meredians after rotation of crystal and after the coinsidence of
the projection of crystal with standard projection
S17- y coordinates of meredians after rotation of crystal and after the coinsidence of
the projection of crystal with standard projection
Fig. 33