- Page 1:
Paperfolding, Automata, and Rationa
- Page 5 and 6:
Paperfolding, Automata, and Rationa
- Page 7 and 8:
Paperfolding Take a rectangular she
- Page 9 and 10: Paperfolding Take a rectangular she
- Page 11 and 12: Paperfolding Take a rectangular she
- Page 13 and 14: Paperfolding Take a rectangular she
- Page 15 and 16: Paperfolding Take a rectangular she
- Page 17 and 18: Paperfolding Take a rectangular she
- Page 19 and 20: Paperfolding Take a rectangular she
- Page 21 and 22: Paperfolding Take a rectangular she
- Page 23 and 24: Paperfolding Take a rectangular she
- Page 25 and 26: Paperfolding Take a rectangular she
- Page 27 and 28: Paperfolding Take a rectangular she
- Page 29 and 30: Paperfolding Take a rectangular she
- Page 31 and 32: A Mahler Functional Equation Aside.
- Page 33 and 34: A Mahler Functional Equation Aside.
- Page 35 and 36: A Mahler Functional Equation Aside.
- Page 37 and 38: A Mahler Functional Equation Aside.
- Page 39 and 40: A Mahler Functional Equation Aside.
- Page 41 and 42: A Mahler Functional Equation Aside.
- Page 43 and 44: A Mahler Functional Equation Aside.
- Page 45 and 46: A Mahler Functional Equation Aside.
- Page 47 and 48: Next, if we pair the sequence Regul
- Page 49 and 50: Next, if we pair the sequence Regul
- Page 51 and 52: The uniform, or regular, 2-substitu
- Page 53 and 54: The uniform, or regular, 2-substitu
- Page 55 and 56: The uniform, or regular, 2-substitu
- Page 57 and 58: The uniform, or regular, 2-substitu
- Page 59: The uniform, or regular, 2-substitu
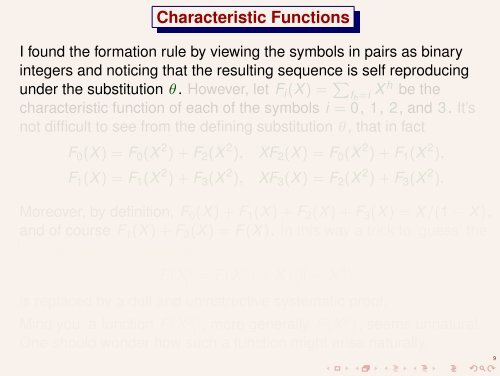
- Page 63 and 64: Characteristic Functions I found th
- Page 65 and 66: Characteristic Functions I found th
- Page 67 and 68: An Algebraic Equation in Characteri
- Page 69 and 70: An Algebraic Equation in Characteri
- Page 71 and 72: An Algebraic Equation in Characteri
- Page 73 and 74: An Algebraic Equation in Characteri
- Page 75 and 76: These remarks show that the paperfo
- Page 77 and 78: These remarks show that the paperfo
- Page 79 and 80: The Thue-Morse Sequence 0 1 10 11 1
- Page 81 and 82: The Thue-Morse Sequence 0 1 10 11 1
- Page 83 and 84: Euler’s Identity and a Functional
- Page 85 and 86: Euler’s Identity and a Functional
- Page 87 and 88: Euler’s Identity and a Functional
- Page 89 and 90: An Algebraic Equation The function
- Page 91 and 92: A Counter-example in Analysis I cla
- Page 93 and 94: A Counter-example in Analysis I cla
- Page 95 and 96: A Counter-example in Analysis I cla
- Page 97 and 98: The Shapiro Sequence Consider, the
- Page 99 and 100: The Shapiro Sequence Consider, the
- Page 101 and 102: The Shapiro Sequence Consider, the
- Page 103 and 104: The Shapiro Sequence Consider, the
- Page 105 and 106: The Shapiro Sequence Consider, the
- Page 107 and 108: A Remark on the Shapiro Function Se
- Page 109 and 110: A Remark on the Shapiro Function Se
- Page 111 and 112:
A Remark on the Shapiro Function Se
- Page 113:
A Remark on the Shapiro Function Se
- Page 116 and 117:
Transcendence of Automatic Numbers
- Page 118 and 119:
Transcendence of Automatic Numbers
- Page 120 and 121:
Transcendence of Automatic Numbers
- Page 122 and 123:
Complexity of a Sequence Given an i
- Page 124:
Complexity of a Sequence Given an i
- Page 127 and 128:
Algebraicity and Automaticity The p
- Page 129 and 130:
Algebraicity and Automaticity The p
- Page 131 and 132:
Algebraicity and Automaticity The p
- Page 133 and 134:
Algebraicity and Automaticity The p
- Page 135 and 136:
Comments on the Proof The best proo
- Page 137 and 138:
Comments on the Proof The best proo
- Page 139 and 140:
Comments on the Proof The best proo
- Page 141 and 142:
But for expansions over the complex
- Page 143 and 144:
But for expansions over the complex
- Page 145:
But for expansions over the complex
- Page 148 and 149:
Power Series in Several Variables R
- Page 150 and 151:
Power Series in Several Variables R
- Page 152 and 153:
Power Series in Several Variables R
- Page 154 and 155:
Power Series in Several Variables R
- Page 156 and 157:
Power Series in Several Variables R
- Page 158 and 159:
Diagonals and Hadamard Products The
- Page 160 and 161:
Diagonals and Hadamard Products The
- Page 162 and 163:
A Theorem of Furstenberg It follows
- Page 164 and 165:
A Theorem of Furstenberg It follows
- Page 166 and 167:
A Theorem of Furstenberg It follows
- Page 168 and 169:
As said, in characteristic zero, ne
- Page 170 and 171:
As said, in characteristic zero, ne
- Page 172 and 173:
As said, in characteristic zero, ne
- Page 174 and 175:
A Beautiful Transcendence Argument
- Page 176 and 177:
A Beautiful Transcendence Argument
- Page 178 and 179:
Breaking Up in Characteristic p The
- Page 180 and 181:
Breaking Up in Characteristic p The
- Page 182 and 183:
Breaking Up in Characteristic p The
- Page 184 and 185:
Algebraic power series in character
- Page 186 and 187:
Algebraic power series in character
- Page 188 and 189:
Algebraic power series in character
- Page 190 and 191:
Hence, since Fp is finite, there ar
- Page 192 and 193:
Hence, since Fp is finite, there ar
- Page 194 and 195:
Suppose the series P aνx ν is gen
- Page 196 and 197:
Suppose the series P aνx ν is gen
- Page 198 and 199:
Suppose the series P aνx ν is gen
- Page 200 and 201:
Suppose the series P aνx ν is gen
- Page 202 and 203:
So we have: Theorem. P aνx ν ∈
- Page 204 and 205:
So we have: Theorem. P aνx ν ∈
- Page 206 and 207:
So we have: Theorem. P aνx ν ∈
- Page 208 and 209:
So we have: Theorem. P aνx ν ∈
- Page 210 and 211:
The Lifting Theorem Looking careful
- Page 212 and 213:
The Lifting Theorem Looking careful
- Page 214 and 215:
The Lifting Theorem Looking careful
- Page 216 and 217:
Diagonals of Rational Functions Now
- Page 218 and 219:
Diagonals of Rational Functions Now
- Page 220 and 221:
Diagonals of Rational Functions Now
- Page 222 and 223:
Diagonals of Rational Functions Now
- Page 224 and 225:
Diagonals of Rational Functions Now
- Page 226 and 227:
Whilst (i) and (ii) are reasonably
- Page 228 and 229:
References MICHEL DEKKING, MICHEL M
- Page 230 and 231:
References MICHEL DEKKING, MICHEL M
- Page 246 and 247:
References MICHEL DEKKING, MICHEL M
- Page 248 and 249:
References MICHEL DEKKING, MICHEL M