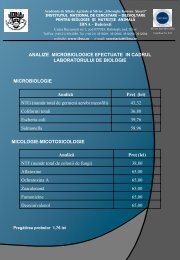
( ) ( ) [ ] ( ) ( ) ( ) ( ) [ ]n ( ) ( ) [ ]n
( ) ( ) [ ] ( ) ( ) ( ) ( ) [ ]n ( ) ( ) [ ]n
( ) ( ) [ ] ( ) ( ) ( ) ( ) [ ]n ( ) ( ) [ ]n
Create successful ePaper yourself
Turn your PDF publications into a flip-book with our unique Google optimized e-Paper software.
Optimal control of some metabolism processes<br />
R. Burlacu<br />
University of Agricultural Sciences and Veterinary Medicine, Bucharest<br />
During the recent years a collective from the Institute of Biology and Animal<br />
Nutrition, Baloteti developed a set of mathematical models of the metabolism<br />
processes for some categories of farm animals. These models describe the evolution of<br />
some synthetic indicators of the process of metabolism (body proteins and lipids)<br />
function of some characteristics of the given food (dietary protein and energy).<br />
Thus, if we note:<br />
Mn = the amount of dietary protein given at moment n;<br />
Vm = the amount of dietary energy given at moment n;<br />
Xn = the amount of body protein at moment n;<br />
Zn = the amount of body lipids at moment n.<br />
We may observe using the existing empirical formulas that these measures are linked<br />
by relations such as:<br />
~<br />
⎪⎧<br />
X<br />
n<br />
+ 1 = X<br />
n<br />
+ f ( xn<br />
, zn<br />
, un<br />
)<br />
⎨<br />
⎪⎩ Z<br />
n<br />
+ 1 = zn<br />
+ g~<br />
( xn<br />
, zn<br />
, unVn<br />
)<br />
where:<br />
Z ∈ [ ~ z , ~ z n 1 2<br />
]; ∈ ~ ~<br />
x<br />
n<br />
[ d1,<br />
( zn ),<br />
d<br />
2<br />
( z n<br />
)]<br />
V<br />
n<br />
∈[ u~<br />
( xn<br />
zn<br />
) u~<br />
1<br />
,<br />
3( xn<br />
, zn<br />
)]<br />
V<br />
n<br />
∈[ v~<br />
( xn<br />
zn<br />
un<br />
), v ~<br />
1<br />
, ,<br />
2<br />
( xn<br />
, zn<br />
)]<br />
~<br />
~ ~<br />
where the functions: f , g~ , u~ 1 , u~<br />
2, v ~ 1, v ~<br />
2,<br />
d1,<br />
d<br />
2<br />
have a rather complicated structure.<br />
A discrete command system was thus developed in which the command variables<br />
(inputs) were un, vn, and the state variables were xn, zn.<br />
The early studies were conducted in order to validate this model by selecting various<br />
strategies (commands (un, vn)) according to the practical experience and by calculating<br />
the outputs (xn, zn) of the model. The experimental data were compared to the<br />
calculated ones and a mean error of 3% was noticed (the standard mean error for this<br />
field is 5%), which showed that the model simulated quite well the actual processes.<br />
The following step was to formulate and solve problems of optimisation; to calculate<br />
commands which to optimise some target functions. Following are the most common<br />
targets among the many possibilities:<br />
1) Produce a given total weight (function of xn, zn) within a minimal period<br />
of time;<br />
2) Produce a maximal amount of protein within a given period of time;<br />
3) Minimize the cost of reaching the target weight at the set deadline.<br />
The complexity of the functions used by the model and of the restrictions (the<br />
commands must have values within the intervals depending on the previous outputs)
make it very difficult, if not impossible, to perform a full and rigorous study of these<br />
problems within the discrete interval mentioned earlier.<br />
On the other hand, some recent results on the dynamic programming within the<br />
theory of the optimal control as well as the existence of performing software for<br />
computer integration of the differential equations seem to offer great chances of<br />
solving them within a continuous model.<br />
The careful analysis of the real phenomena forming the background of the discrete<br />
model yields grounds for the development of a continuous model of the same pattern.<br />
Thus, if we note:<br />
x(t) = the amount of body protein at moment t<br />
z(t) = the amount of body lipids at moment t<br />
u(t) = the amount of dietary protein given at moment t<br />
v(t) = the amount of dietary energy given at moment t<br />
then, these functions are linked by differential equations:<br />
~<br />
⎧x'(<br />
t)<br />
= f ( x( t) , z( t) , u( t)<br />
)<br />
(2) ⎨<br />
⎩z'<br />
( t) = g~<br />
( x( t) , z( t) , u( t) , v( t)<br />
)<br />
with u (t) ∈[ u ~ ( x( t) z( t)<br />
) u~<br />
1<br />
, ,<br />
3( t) , z( t)<br />
]<br />
v(t) ∈[ v ~ ( x( t) , z( t) , u( t)<br />
), v ~ ( x( t) , z( t)<br />
)]<br />
1<br />
2<br />
The analysis of ~ f , g~ , u~ ~<br />
1 , u2, v ~ 1, v ~<br />
2<br />
functions shows that the system may be<br />
considerably simplified if variable z(t) is replaced by variable y(t) = total body weight<br />
at moment, given by:<br />
(3) y( t)<br />
x<br />
=<br />
( t) + z( t) + α + z( t)<br />
1−α<br />
1<br />
3<br />
α<br />
2<br />
where α<br />
1<br />
, α<br />
2,<br />
α<br />
3<br />
> 0 are given constants.<br />
With the new variables (x(.),y(.)), which are more convenient as terminal conditions<br />
and as criteria of optimisation too, the command system (2) becomes:<br />
x ( ( ))<br />
( ) [ ( ) ( )]<br />
( ) [ ( ( )),<br />
v ( y)<br />
]<br />
⎧ ′ = f y,<br />
u t , u t ∈ u1<br />
y , u3<br />
y<br />
(4) ⎨<br />
⎩y′<br />
= g( x,<br />
y,<br />
u( t) , v( t)<br />
),<br />
v t ∈ v1<br />
x,<br />
y,<br />
u t<br />
where functions ~ f , g~ , u~ ~<br />
1 , u2, v ~ 1, v ~<br />
2<br />
are as following:<br />
2<br />
f(y,u) =<br />
⎧b<br />
⎨<br />
⎩A;<br />
( y u)<br />
α<br />
= α u −α<br />
y ; u ∈ u ,( y) , u ( y)<br />
6<br />
,<br />
4 5<br />
u ∈<br />
[<br />
1 2<br />
]<br />
[ u ( y) , u ( y)<br />
]<br />
2<br />
2<br />
α<br />
A<br />
u<br />
1<br />
=<br />
2 1<br />
;<br />
3<br />
+<br />
α<br />
α<br />
5 α6<br />
( y) y ; u ( y) = u ( y) + u ( y) = ay b<br />
4<br />
4
(5) g ( x,<br />
y,<br />
u,<br />
v)<br />
= a( x) f ( y,<br />
u) + C( y,<br />
u,<br />
v)<br />
a<br />
( x)<br />
=<br />
x<br />
β<br />
β<br />
2<br />
4<br />
β β 1<br />
+ β<br />
3<br />
+<br />
x −<br />
5<br />
C (y,u,v) = δ1V1<br />
−δ<br />
2u<br />
−δ<br />
3<br />
y<br />
⎛ θ<br />
4<br />
V1(x,y,u) =<br />
1u 2<br />
y<br />
3<br />
f ( y,<br />
u)<br />
5<br />
x ⎟ ⎞<br />
θ + θ +<br />
⎜θ<br />
+<br />
⎝ θ − ⎠<br />
where A: α1... α<br />
6;<br />
β1...<br />
β<br />
5;<br />
δ1...<br />
δ<br />
4;<br />
θ1...<br />
θ<br />
5;a,<br />
b are given constants.<br />
The experimental data show that the state variables x, y must fall within preset limits.<br />
(6)<br />
y ∈<br />
x ∈<br />
[ y1,<br />
y2<br />
] = [ 30,110]<br />
[ d ( y) , d ( y)<br />
]<br />
1<br />
2<br />
Other limits can also be set but the constants used to define the functions from (5) may<br />
change.<br />
As it can be observed, the functions defining system (4) are generally quite<br />
complicated but the problem is now clearly formulated. It may also be noticed that (4)<br />
is a command system whose data are non-differentiable (but are Liepschtesian), which<br />
requires methods of the non-differentiable analysis that developed a lot recently.<br />
Furthermore, the functions from (5) that define system (4) have a structure of stratified<br />
functions and therefore the methods from (3) might apply.<br />
Each of the optimisation problems presented above consists in the minimisation of a<br />
cost functional defined as follows:<br />
The problem of the minimum period is formulated as follows:<br />
Given yF ∈ (y1, y2], for any<br />
(x0,y0) ∈X0 = {( x, y) / y ∈ [ y1 , y2<br />
); x ∈[ d1( y) , d<br />
2<br />
( y)<br />
] } determine ~ t > F<br />
0 and<br />
2<br />
( u~<br />
(.), v ~ (.) ):[ 0, t<br />
F<br />
] → IR +<br />
so that the system of differential equations from(4)<br />
admits a solution ( ~ x (.),<br />
~ y (.))<br />
defined on [0,tF] that verifies the restrictions from (4) and<br />
~ x t , ~ y t ∈ X ∀t<br />
∈ 0,<br />
~<br />
t , y<br />
~<br />
t = y<br />
(7) ( ( ) ( )) 0<br />
[<br />
F<br />
] (<br />
F<br />
)<br />
F<br />
and which minimises the functional; C (u(.), v(.)) defined by:<br />
t<br />
(8) C (u(.), v(.)) = tF =<br />
∫ F<br />
dt<br />
0<br />
in the class of all the admitted commands having these properties.<br />
In order to calculate the maximal amount of protein produced during a given period,<br />
T>0 one must minimise functional C (.,.) defined by:<br />
(9) C (u(.),v(.)) = -x(T) for each:
(t0,x0,y0) ∈ E0 = {( , x,<br />
y) / t ( 0, T ),<br />
y ∈( y , y ),<br />
x ∈[ d ( y) d ( y)<br />
]}<br />
t<br />
1 2<br />
1<br />
,<br />
2<br />
of all admitted commands (u(.), v(.)) : [0,T] → IR 2 +<br />
and the restrictions:<br />
∈ in class υ (t0,x0,y0)<br />
that verify the restrictions from (4)<br />
(9) (t,x(t), y(t)) ∈E0 ∀ t ∈(t0,T)<br />
In order to minimise the cost of producing the target weight within the set deadline,<br />
T>0 one must minimise functional C (.,.) defined by:<br />
(10) C(u(.), v(.)) = ( C u( t) C v( t)<br />
)<br />
t<br />
∫ 1<br />
+<br />
2<br />
dt.<br />
0<br />
where C1,C2 > 0 are the prices of the dietary protein and energy.<br />
The next step to be taken subsequently is to use the results and models from the<br />
literature to solve these problems of optimal control.<br />
References<br />
Burlacu Gh., R. Burlacu, I. Columbeanu, G. Alexandru <br />
proceselor de metabolism la monogastrice<br />
, 1987 -<br />
<br />
Român de Biometrie la Academia R.S.R. –<br />
1986 - Sufficient optimality condition for stratified optimal control<br />
problems, SIAM J Cont and Opt 24 (), 675-695.<br />
<br />
–<br />
Whittemore C.T. 1983 - Agricultural Systems, p 159-186.


![( ) ( ) [ ] ( ) ( ) ( ) ( ) [ ]n ( ) ( ) [ ]n](https://img.yumpu.com/22443310/1/500x640/-n-n.jpg)