Technical University Kaiserslautern Preprint - Fachbereich Physik ...

2
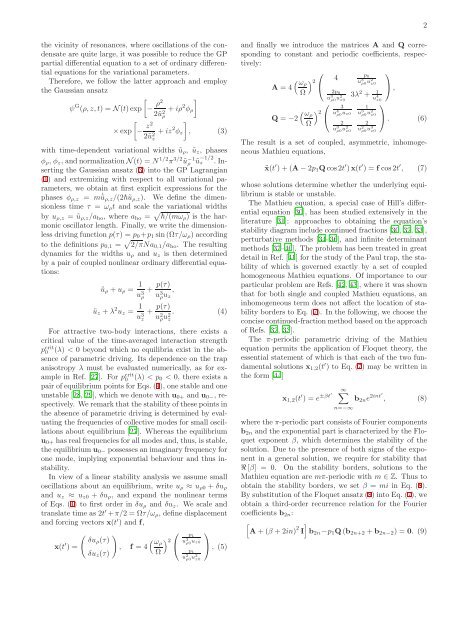
the vicinity of resonances, where oscillations of the condensate
are quite large, it was possible to reduce the GP
partial differential equation to a set of ordinary differential
equations for the variational parameters.
Therefore, we follow the latter approach and employ
the Gaussian ansatz
]
ψ G (ρ,z,t) = N(t)exp
[− ρ2
+iρ 2 φ ρ
2ũ 2 ρ
]
×exp
[− z2
2ũ 2 +iz 2 φ z , (3)
z
with time-dependent variational widths ũ ρ , ũ z , phases
φ ρ , φ z , andnormalizationN(t) = N 1/2 π 3/2 ũ −1
ρ ũ−1/2 z .Inserting
the Gaussian ansatz (3) into the GP Lagrangian
(1) and extremizing with respect to all variational parameters,
we obtain at first explicit expressions for the
phases φ ρ,z = m˙ũ ρ,z /(2ũ ρ,z ). We define the dimensionless
time τ = ω ρ t and scale the variational widths
by u ρ,z = ũ ρ,z /a ho , where a ho = √ /(mω ρ ) is the harmonic
oscillator length. Finally, we write the dimensionless
driving function p(τ) = p 0 +p 1 sin(Ωτ/ω ρ ) according
to the definitions p 0,1 = √ 2/πNa 0,1 /a ho . The resulting
dynamics for the widths u ρ and u z is then determined
by a pair of coupled nonlinear ordinary differential equations:
ü ρ +u ρ = 1 u 3 ρ
ü z +λ 2 u z = 1 u 3 z
+ p(τ)
u 3 ρu z
,
+ p(τ)
u 2 ρu 2 . (4)
z
For attractive two-body interactions, there exists a
critical value of the time-averaged interaction strength
p crit
0 (λ) < 0 beyond which no equilibria exist in the absence
of parametric driving. Its dependence on the trap
anisotropy λ must be evaluated numerically, as for example
in Ref. [27]. For p crit
0 (λ) < p 0 < 0, there exists a
pair ofequilibrium points for Eqs. (4), one stable and one
unstable [28, 29], which we denote with u 0+ and u 0− , respectively.
Weremarkthatthe stabilityofthesepointsin
the absence of parametric driving is determined by evaluating
the frequencies of collective modes for small oscillations
about equilibrium [27]. Whereas the equilibrium
u 0+ hasrealfrequenciesforallmodesand, thus, is stable,
the equilibrium u 0− possessesanimaginaryfrequencyfor
one mode, implying exponential behaviour and thus instability.
In view of a linear stability analysis we assume small
oscillations about an equilibrium, write u ρ ≈ u ρ0 + δu ρ
and u z ≈ u z0 + δu ρ , and expand the nonlinear terms
of Eqs. (4) to first order in δu ρ and δu z . We scale and
translate time as 2t ′ +π/2 = Ωτ/ω ρ , define displacement
and forcing vectors x(t ′ ) and f,
( ) ⎛
p
δuρ (τ) (
x(t ′ ωρ
)
1
⎞
2 u
) = , f = 4 ⎝
3 ρ0 uz0
⎠, (5)
δu z (τ) Ω
p 1
u 2 ρ0 u2 z0
and finally we introduce the matrices A and Q corresponding
to constant and periodic coefficients, respectively:
A = 4
Q = −2
⎛
( ωρ
) 2
⎝
Ω
⎛
( ωρ
) 2
⎝
Ω
4
2p 0
u 3 ρ0 u2 z0
p 0
u 3 ρ0 u2 z0
3λ 2 + 1
u 4 z0
3
u 4 ρ0 uz0 1
u 3 ρ0 u2 z0
2
u 3 ρ0 u2 z0
2
u 2 ρ0 u3 z0
⎞
⎞
⎠,
⎠. (6)
The result is a set of coupled, asymmetric, inhomogeneous
Mathieu equations,
ẍ(t ′ )+(A−2p 1 Qcos2t ′ )x(t ′ ) = f cos2t ′ , (7)
whose solutions determine whether the underlying equilibrium
is stable or unstable.
The Mathieu equation, a special case of Hill’s differential
equation [30], has been studied extensively in the
literature [31]: approaches to obtaining the equation’s
stability diagram include continued fractions [30, 32, 33],
perturbative methods [34–36], and infinite determinant
methods [37–40]. The problem has been treated in great
detail in Ref. [41] for the study of the Paul trap, the stability
of which is governed exactly by a set of coupled
homogeneous Mathieu equations. Of importance to our
particular problem are Refs. [42, 43], where it was shown
that for both single and coupled Mathieu equations, an
inhomogeneous term does not affect the location of stability
borders to Eq. (7). In the following, we choose the
concisecontinued-fractionmethod basedontheapproach
of Refs. [32, 33].
The π-periodic parametric driving of the Mathieu
equation permits the application of Floquet theory, the
essential statement of which is that each of the two fundamental
solutions x 1,2 (t ′ ) to Eq. (7) may be written in
the form [44]
x 1,2 (t ′ ) = e ±βt′
∞ ∑
n=−∞
b 2n e 2int′ , (8)
where the π-periodic part consists of Fourier components
b 2n and the exponential part is characterized by the Floquet
exponent β, which determines the stability of the
solution. Due to the presence of both signs of the exponent
in a general solution, we require for stability that
R[β] = 0. On the stability borders, solutions to the
Mathieu equation are mπ-periodic with m ∈ Z. Thus to
obtain the stability borders, we set β = mi in Eq. (8).
By substitution of the Floquet ansatz(8) into Eq. (7), we
obtain a third-order recurrence relation for the Fourier
coefficients b 2n :
[ ]
A+(β +2in) 2 I b 2n −p 1 Q(b 2n+2 +b 2n−2 ) = 0. (9)