Mathematical Optimization in Graphics and Vision - Luiz Velho - Impa

2.7. THE PROBLEM OF POSING PROBLEMS 31
3. The solution depends continuously on the initial conditions.
When at least one of the above conditions is not satisfied, we say that the
problem is ill-posed.
We have seen previously that in general inverse problems of the first and second
kind are ill-posed in the sense of Hadamard because of the non-uniqueness of the
solution,
The concept of ill-posed problem needs to be further investigated very carefully.
The continuity condition is important because it guarantees that small variations
of the initial conditions will cause only small perturbations of the solution.
Recall that, in practical problems, small variations in the initial conditions are
quite common (and sometimes, inevitable) due to measurement imprecision or
numerical errors. The non-uniqueness of solutions, on the other hand, should not
be necessarily an obstacle. An example will make this point clear.
Example 13. Consider the operator F : R 2 → R defined by F (x, y) = x 2 +y 2 −1
and the inverse problem of the first kind F (x, y) = 0. That is, we must solve the
quadratic equation x 2 + y 2 − 1 = 0. It is clear that it admits an infinite number of
solutions, therefore it is ill-posed in the sense of Hadamard. Geometrically, these
solutions constitute the unit circle S 1 of the plane. The non-uniqueness of the
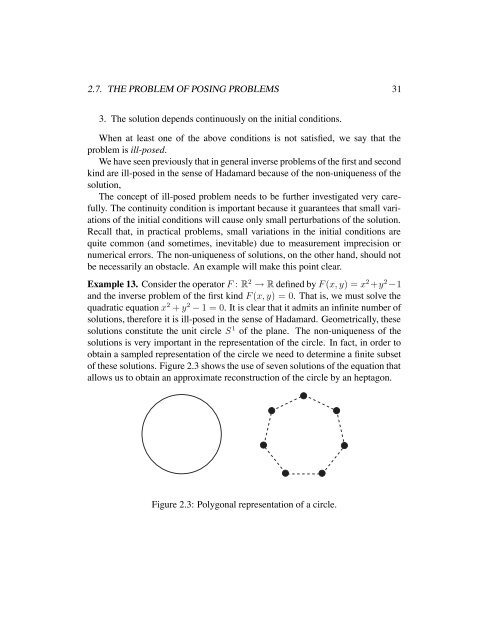
solutions is very important in the representation of the circle. In fact, in order to
obtain a sampled representation of the circle we need to determine a finite subset
of these solutions. Figure 2.3 shows the use of seven solutions of the equation that
allows us to obtain an approximate reconstruction of the circle by an heptagon.
Figure 2.3: Polygonal representation of a circle.