PHYSICS 455 Assignment 3 Due February 15, 2000 1. A simple ...

PHYSICS 455
Assignment 3
Due February 15, 2000
1. A simple model of rubber elasticity. Consider a polymer chain of N rigid links, but with
flexible joints between the junctions. Each link has a length a, and can point independently
along any of the x, −x, y, −y, z, −z axes (yes, it can point back on itself). The entire assembly
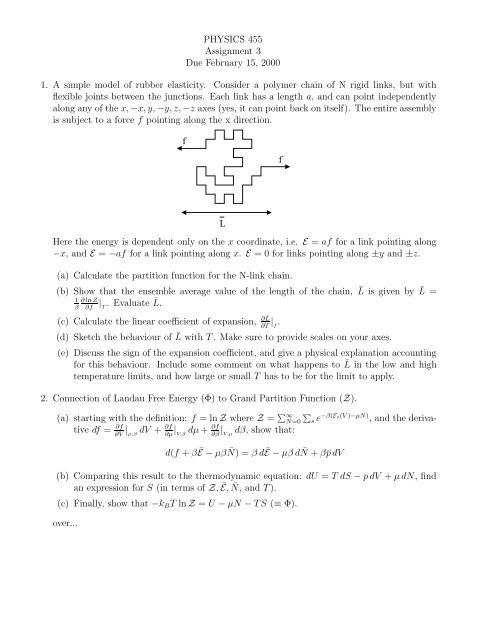
is subject to a force f pointing along the x direction.
f
f
L
Here the energy is dependent only on the x coordinate, i.e. E = af for a link pointing along
−x, and E = −af for a link pointing along x. E = 0 for links pointing along ±y and ±z.
(a) Calculate the partition function for the N-link chain.
(b) Show that the ensemble average value of the length of the chain, ¯L is given by ¯L =
. Evaluate ¯L.
1
β
∂ ln Z
∂f
| T
(c) Calculate the linear coefficient of expansion, ∂ ¯L
∂T | f .
(d) Sketch the behaviour of ¯L with T . Make sure to provide scales on your axes.
(e) Discuss the sign of the expansion coefficient, and give a physical explanation accounting
for this behaviour. Include some comment on what happens to ¯L in the low and high
temperature limits, and how large or small T has to be for the limit to apply.
2. Connection of Landau Free Energy (Φ) to Grand Partition Function (Z).
(a) starting with the definition: f = ln Z where Z = ∑ ∞
N=0
∑s e −β(Es(V )−µN) , and the derivative
df = ∂f | ∂f
dV + | ∂f
dµ + | dβ, show that:
∂V µ,β ∂µ V,β ∂β V,µ
d(f + βĒ − µβ ¯N) = β dĒ − µβ d ¯N + β ¯p dV
(b) Comparing this result to the thermodynamic equation: dU = T dS − p dV + µ dN, find
an expression for S (in terms of Z, Ē, ¯N, and T ).
(c) Finally, show that −k B T ln Z = U − µN − T S (≡ Φ).
over...

3. Consider a system consisting of a single impurity atom/ion in a semiconductor. Suppose that
the impurity atom has one “extra” electron compared to the neighboring atoms, as would a
phosphorus atom occupying a lattice site in a silicon crystal. The extra electron is then easily
removed, leaving behind a positively charged ion. The ionized electron is called a conduction
electron, because it is free to move through the material; the impurity atom is called a donor,
because it can “donate” a conduction electron.
(a) Derive a formula for the probability of a single donor atom being ionized. Do not neglect
the fact that the electron, if present, can have two independent spin states. Express your
formula in terms of the temperature, the ionization energy I, and the chemical potential
of the “gas” of conduction electrons.
(b) Assuming that the conduction electrons behave like an ordinary ideal gas (with two spin
states per particle), write their chemical potential in terms of the number of conduction
electrons per unit volume, N c /V .
(c) Now assume that every conduction electron comes from an ionized donor atom. In
this case the number of conduction electrons is equal to the number of donors that are
ionized. Use this condition to derive a quadratic equation for N c in terms of the number
of donor atoms (N d ), eliminating µ. Solve for N c using the quadratic formula. (It may
be helpful to introduce some abbreviations for dimensionless quantities. Try x = N c /N d ,
t = k B T/I, and so on.)
4. Hemoglobin is found as assemblies of four protein subunits, with each subunit having an
oxygen binding heme site. The tendency of oxygen to bind to a heme site increases as the
other sites become occupied. This system may have zero, one, two, three, or four oxygen
molecules bound. Taking the energy of the unbound molecule to be 0, assume that the energy
of the singly occupied states is -0.55 eV, the doubly occupied states as -1.3 eV, the triply
occupied states as -2.0 eV, and the quadruply occupied states as -3 eV, calculate and plot the
fraction of occupied sites as a function of the effective partial pressure of oxygen. Compare
to the graph we sketched in class for the independent site (Myoglobin) case.