Normal Modes Goals: Additional Resources: Problems:

More documents

Recommendations

Info

2. The displacements of two coupled oscillators q 1 and q 2 with mass m are described by the coupled equations m¨q 1 = k(q 2 − 2q 1 ) and m¨q 2 = k(q 1 − 2q 2 ), where k is a constant. The normal modes are given by Q 1 = (q 1 + q 2 )/ √ 2 and Q 2 = (q 1 − q 2 )/ √ 2 which evolve according to Q 1 = A cos ω 1 t + B sin ω 1 t and Q 2 = C cos ω 2 t + D sin ω 2 t, where ω 1 = √ k/m and ω 2 = √ 3k/m are the normal mode frequencies. (a) Find expressions for q 1 (t) and q 2 (t). (b) The forces appearing in the equations for q 1 and q 2 may be interpreted in terms of a potential energy function V (q 1 , q 2 ), as follows. We write the equations as Two equal masses m are connected as shown with two identical massless springs, of spring constant k. Considering m¨q 1 = − dV only motion , and m¨q 2 = − dV in the vertical direction, , obtain the differential equations dq for 1 the displacements dq 2 of the two masses from their equilibrium positions. Show that the angular frequencies of the normal modes are given by generalising “mẍ = dV/dx”. Show that V may be taken to be V = k(q 2 1 + q 2 2 − q 1 q 2 ). (c) Rewrite V in terms of the normal mode coordinates. Solution: V = mω 2 1Q 2 1/2 + mω 2 2Q 2 2/2. 2 ! = ± ( ) 3 5 k / 2m (d) Calculate the total kinetic energy K of the two masses. Solution: K = m( ˙Q 2 1 + ˙Q 2 2)/2. (e) Write Find down the the ratio total of energy the amplitudes V + K in terms of the oftwo coordinates masses Qin 1 each and Qseparate 2 and also mode. in terms of q 1 and q 2 . Discuss similarities and differences of these two expressions. Why does the acceleration due to gravity not appear in these answers? 3. Two masses m are fixed to springs at B and C as shown in Fig. 1. They are displaced by small distances x 1 5. and x 2 *AB, from their BC, equilibrium and CD are positions identical alongsprings the line with of thenegligible springs, andmass, execute and small stiffness oscillations. The springs have negligible masses. constant k: A B C D Figure 1: Masses connected by springs. (a) AB, BC, and CD are identical springs with stiffness constant k. Show that the angular frequencies of the normal modes are The masses m, fixed to the springs √ at B and C, are √ displaced by small distances k 3k x 1 and x 2 from their equilibrium ω 1 = positions along the line of the springs, and m and ω 3 = m execute small oscillations. Show that the angular frequencies of the normal modes are ! 1 = k / m and ! 2 = 3 k / m . Sketch how the two masses move in each mode. Find x 1 and x 2 at times t > 0 if at t = 0 the system is at rest with x 1 = a, x 2 = 0. Sketch how the two masses move in each mode. Find x 1 and x 2 at times t > 0 if at t = 0 the system is at rest with x 1 = a, x 2 = 0. (b) Now consider the case where the springs AB and CD have stiffness constant k 0 , while BC has stiffness constant k 1 . If C is clamped, B vibrates with frequency ν 0 = 1.81Hz. The frequency of the lower frequency normal mode is ν 1 = 1.14Hz. Calculate the frequency of the higher frequency normal mode, and the ratio k 1 /k 0 . 6. *The setup is as for question 5, except that in this case the springs AB and CD have stiffness constant k 0 , while BC has stiffness constant k 1 . If C is clamped, B vibrates with frequency ν 0 = 1.81 Hz. The frequency of the lower frequency L d2 I 1 normal mode dt 2 is + I 1 ν 1 C= − 1.14 M d2 I 2 dt Hz. 2 = Calculate 0, and I 2 the Ld2 dtfrequency 2 + I 2 C − M d2 I 1 of dt the 2 = higher 0, frequency normal mode, and the ratio k 1 /k 0 . (From French 5-7). 4. The currents I 1 and I 2 in two coupled LC circuits satisfy the equations where 0 < M < L. Find formulae for the two possible frequencies at which the coupled system may oscillate sinusoidally. 7. Two particles 1 and 2, each of mass m, are connected by a light spring of stiffness k, and are free to slide along a smooth horizontal track. What are the normal 2 d2 y frequencies of this system? Describe the motion in the mode of zero dx frequency. 2 − 3 dy dx + 2 dz dx + 3y + z = d 2 y e2x , and dx Why does a zero-frequency mode appear 2 − 3 dy dx + dz + 2y − z = 0, dx in this problem, but not in question 5, for example? 5. Solve the differential equations Is it possible to have a solution to these equations for which y = z = 0 when x = 0? Particle 1 is now subject to a harmonic driving force F cos!t . In the steady
V + K = mQ! 1 + m! 1Q1 + mQ! $ % $ 2 + m! 2Q2 % = E1 + E2 & 2 2 ' & 2 2 ' where E 1 is the total energy of ‘oscillator’ Q 1 with frequency ω 1 , and similarly for E 2 . What is the expression for the total energy when written in terms of q 1 , q 2 , q! 1 , and q! 2 ? Discuss the similarities and differences. 6. Two particles 1 and 2, each of mass m, are connected by a light spring of stiffness k, and are free to slide along a smooth horizontal track. What are the normal frequencies of this system? Describe the (d) Find the equations of motion for Q 1 and Q 2 from Newton’s law in the form motion in the mode of zero frequency. Why does a zero-frequency mode appear in this problem, but not V in problem 3, for example? mQ !! ! 1 = " Particle 1 is now subject to aQ , V mQ!! ! = " , ! 2 harmonic 1 driving force F ! cos Q2 ωt . In the steady state, the amplitudes of vibration and hence ofre-derive 1 and 2 are solution A and(2). B respectively. Find A and B, and discuss qualitatively the behaviour of the system as ω 2 is slowly increased from values near zero to values greater than 2k/m. 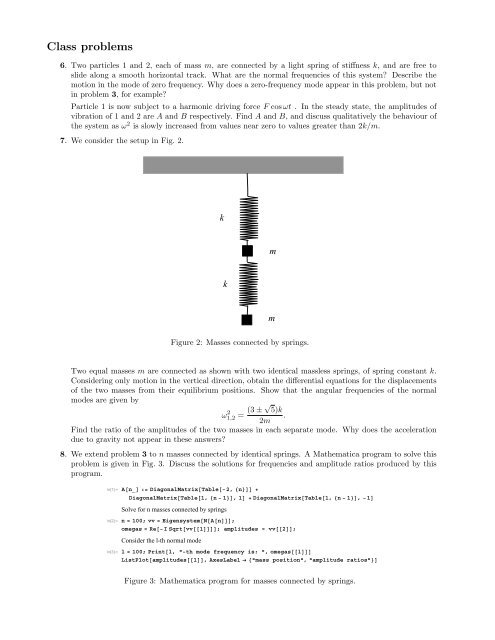7. 4.* We consider the setup in Fig. 2. Class problems k m k m Figure 2: Masses connected by springs. Two equal masses m are connected as shown with two identical massless springs, of spring constant k. Considering only motion in the vertical direction, obtain the differential equationsW2Q: for the 3 displacements of the two masses from their equilibrium positions. Show that the angular frequencies of the normal modes are given by ω1,2 2 = (3 ± √ 5)k 2m . Find the ratio of the amplitudes of the two masses in each separate mode. Why does the acceleration due to gravity not appear in these answers? 8. We extend problem 3 to n masses connected by identical springs. A Mathematica program to solve this problem is given in Fig. 3. Discuss the solutions for frequencies and amplitude ratios produced by this program. In[1]:= In[2]:= In[3]:= An_ :⩵ DiagonalMatrixTable2, n DiagonalMatrixTable1, n 1, 1 DiagonalMatrixTable1, n 1, 1 Solve for n masses connected by springs n ⩵ 100; vv ⩵ EigensystemNAn; omegas ⩵ ReI Sqrtvv1; amplitudes ⩵ vv2; Consider the l-th normal mode l ⩵ 100; Printl, "th mode frequency is: ", omegasl ListPlotamplitudesl, AxesLabel "mass position", "amplitude ratios" Figure 3: Mathematica program for masses connected by springs.
Page 1: Normal Modes D. Jaksch 1 Goals: •

Normal Modes Goals: Additional Resources: Problems:

You also want an ePaper? Increase the reach of your titles

Delete template?

Save as template?