Chapter 3. Duality in convex optimization

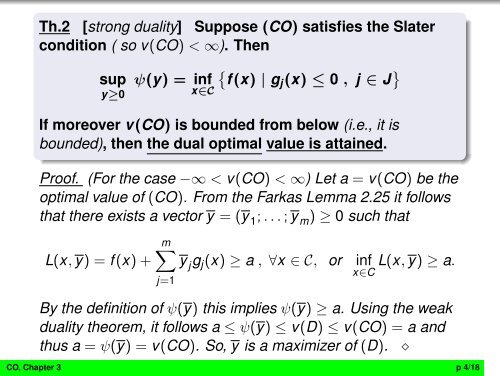
Th.2 [strong duality] Suppose (CO) satisfies the Slater
condition ( so v(CO) < ∞). Then
sup
y≥0
{
ψ(y) = inf f (x) | gj (x) ≤ 0 , j ∈ J }
x∈C
If moreover v(CO) is bounded from below (i.e., it is
bounded), then the dual optimal value is attained.
Proof. (For the case −∞ < v(CO) < ∞) Let a = v(CO) be the
optimal value of (CO). From the Farkas Lemma 2.25 it follows
that there exists a vector y = (y 1 ; . . . ; y m ) ≥ 0 such that
m∑
L(x, y) = f (x) + y j g j (x) ≥ a , ∀x ∈ C, or
j=1
inf L(x, y) ≥ a.
x∈C
By the definition of ψ(y) this implies ψ(y) ≥ a. Using the weak
duality theorem, it follows a ≤ ψ(y) ≤ v(D) ≤ v(CO) = a and
thus a = ψ(y) = v(CO). So, y is a maximizer of (D). ⋄
CO, Chapter 3 p 4/18