Denition approach of learning new topics

Recommendations

Info

26 4 DERIVATIVES seconds will travel 2t metres. So the function relating the time taken t to travel x distance is given by 5 x = 2t or x(t) = 2t So what is the rate of change of displacement with respect to time, between t = 1 and t = 2. We know that rate of change of displacement is ∆x ∆t = x 2 − x 1 = 4 − 2 t 2 − t 1 2 − 1 = 2. In physics, rate of change of diplacement is termed as velocity. Hence the average velocity is 2. What if we want to nd the instateneous velocity at t = 1. For that we need to get the secant at t = 1 which is a tangent. And we know that the tangent is the derivative. So the velocity at t = 1 is dx dt = d dt (2t) = 2 2. A particle starts moving from a starting point A such that velocity at every point is given by v = 2t 3 where t is the time taken. Then how is acceleration related to velocity and time taken. v = 2t 3 The rate of change of velocity is termed in physics as acceleration i.e. a = dv . Dierentiating we get, dt a = dv dt = d dt (2t3 ) = 2 d dt (t3 ) = 2 · 3t 2 = 6t 2 4.5 Instantaneous rate of change[3] Now we know that average rate of change in the function y = f(x) is represented as ∆y and to get the instantaneous rate we use the limit of average ∆x ∆y lim ∆x→0 ∆x 5 Since x can be expressed in terms of variable t. Second notation describes that. We have see if a function f is expresses in terms of x then we write it as y = f(x).
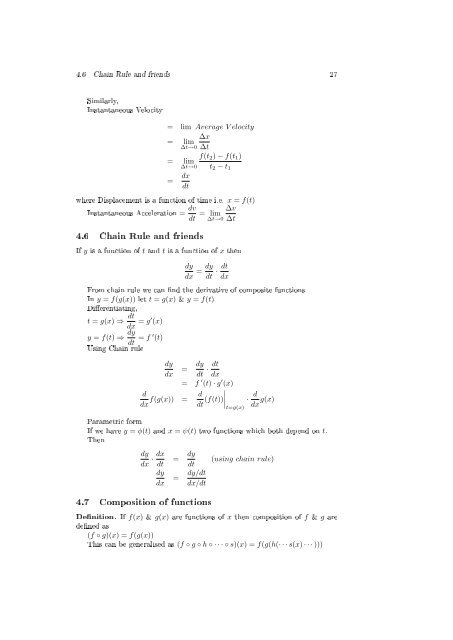
4.6 Chain Rule and friends 27 Similarly, Instantaneous Velocity = lim Average V elocity ∆x = lim ∆t→0 ∆t f(t 2 ) − f(t 1 ) = lim ∆t→0 t 2 − t 1 = dx dt where Displacement is a function of time i.e. x = f(t) Instantaneous Acceleration = dv dt = lim ∆v ∆t→0 ∆t 4.6 Chain Rule and friends If y is a function of t and t is a function of x then dy dx = dy dt · dt dx From chain rule we can nd the derivative of composite functions In y = f(g(x)) let t = g(x) & y = f(t) Dierentiating, t = g(x) ⇒ dt dx = g′ (x) y = f(t) ⇒ dy dt = f ′ (t) Using Chain rule dy dx = dy dt · dt dx = f ′ (t) · g ′ (x) d dx f(g(x)) = d ∣ ∣∣∣t=g(x) dt (f(t)) d · dx g(x) Parametric form If we have y = φ(t) and x = ψ(t) two functions which both depend on t. Then dy dx · dx dt = dy dt dy dx = dy/dt dx/dt 4.7 Composition of functions (using chain rule) Denition. If f(x) & g(x) are functions of x then composition of f & g are dened as (f ◦ g)(x) = f(g(x)) This can be generalised as (f ◦ g ◦ h ◦ · · · ◦ s)(x) = f(g(h(· · · s(x) · · · )))
Page 1 and 2: Foundation of Calculus July 15, 200
Page 3 and 4: CONTENTS 3 5.4 Methods of Solving I
Page 5 and 6: 1.2 Dene slope for two points 5 1.2
Page 7 and 8: 1.4 Slopes of line 7 1.4 Slopes of
Page 9 and 10: 2.4 Domain and range of a function
Page 11 and 12: 11 Part II Lecture 2 3 Examples of
Page 13 and 14: 3.3 Rotating a marble tied to a cor
Page 15 and 16: 3.5 Denition of Limit 15 3.5 Deniti
Page 17 and 18: 3.7 Worked out problems in limits 1
Page 19 and 20: 19 (b) lim x→1 + (c) lim x→1
Page 21 and 22: 21 dy dx = lim f(x + h) − f(x) h
Page 23 and 24: 4.1 Derivatives of other functions
Page 25: 4.3 Rate of change 25 Obviously, if
Page 29 and 30: 4.8 Derivative of a composite funct
Page 31 and 32: 5.3 Denite Integration 31 (a) d dx
Page 33 and 34: 5.3 Denite Integration 33 from the
Page 35 and 36: 5.4 Methods of Solving Integrals 35
Page 37: REFERENCES 37 References [1] Thomas

Denition approach of learning new topics

Create successful ePaper yourself

Delete template?

Save as template?