15) Given a 2D, non-âdivergent flow on an f-âplane with a velocity fiel
15) Given a 2D, non-âdivergent flow on an f-âplane with a velocity fiel
15) Given a 2D, non-âdivergent flow on an f-âplane with a velocity fiel
Create successful ePaper yourself
Turn your PDF publications into a flip-book with our unique Google optimized e-Paper software.
<str<strong>on</strong>g>15</str<strong>on</strong>g>) <str<strong>on</strong>g>Given</str<strong>on</strong>g> a <str<strong>on</strong>g>2D</str<strong>on</strong>g>, <str<strong>on</strong>g>n<strong>on</strong></str<strong>on</strong>g>-‐divergent <str<strong>on</strong>g>flow</str<strong>on</strong>g> <strong>on</strong> <strong>an</strong> f-‐pl<strong>an</strong>e <strong>with</strong> a <strong>velocity</strong> <strong>fiel</strong>d in which u = −y + y 3<br />
<strong>an</strong>d v = x − x 3 : <br />
€<br />
a. Verify that the <str<strong>on</strong>g>flow</str<strong>on</strong>g> is <str<strong>on</strong>g>n<strong>on</strong></str<strong>on</strong>g>-‐divergent. <br />
€ ∂u<br />
To show that the <str<strong>on</strong>g>flow</str<strong>on</strong>g> is <str<strong>on</strong>g>n<strong>on</strong></str<strong>on</strong>g>-‐divergent, we must show that <br />
∂x + ∂v<br />
∂y = 0. <br />
∂<br />
∂x (−y + y 3 ) + ∂ ∂y (x − x 3 ) = 0 + 0 = 0<br />
b. Determine the vorticity at t = t 0<br />
. <br />
€ We know ζ = ∂v<br />
∂x − ∂u . Solving for ζ, we get: <br />
∂y<br />
ζ = ∂v €<br />
∂x − ∂u<br />
∂y = ∂ ∂x (x − x 3 ) − ∂ ∂y (−y + y 3 ) =1− 3x 2 +1− 3y 2 = 2 − 3(x 2 + y 2 )<br />
€<br />
€<br />
c. Determine the local time derivative of vorticity at t = t 0<br />
. <br />
The <str<strong>on</strong>g>2D</str<strong>on</strong>g> Lagr<strong>an</strong>gi<strong>an</strong> vorticity equati<strong>on</strong> c<strong>an</strong> be written as: <br />
€ Dη<br />
Dt = ∂ζ<br />
∂t + ∂f<br />
∂t + u∂ζ ∂x + u∂f ∂x + v ∂ζ<br />
∂y + v df . However, <strong>with</strong> the assumpti<strong>on</strong> of <strong>an</strong> <br />
dy<br />
€<br />
Dζ<br />
f-‐pl<strong>an</strong>e, f = f 0<br />
the equati<strong>on</strong> reduces to <br />
Dt = ∂ζ<br />
∂t + u∂ζ ∂x + v ∂ζ <strong>an</strong>d given that <br />
∂y<br />
Dζ<br />
∂ζ ∂ζ<br />
€ the <str<strong>on</strong>g>flow</str<strong>on</strong>g> is <str<strong>on</strong>g>n<strong>on</strong></str<strong>on</strong>g>-‐divergent = 0, which leaves = −u<br />
Dt ∂t ∂x − v ∂ζ . Now we <br />
∂y<br />
€ ∂ζ<br />
solve for <br />
€<br />
∂t . <br />
∂ζ<br />
€<br />
€<br />
∂t = (y − y 3 ) ∂ ∂x [2 − 3(x 2 + y 2 )] − (x − x 3 ) ∂ ∂y [2 − 3(x 2 + y 2 )] = 6xy(y 2 − x 2 ) <br />
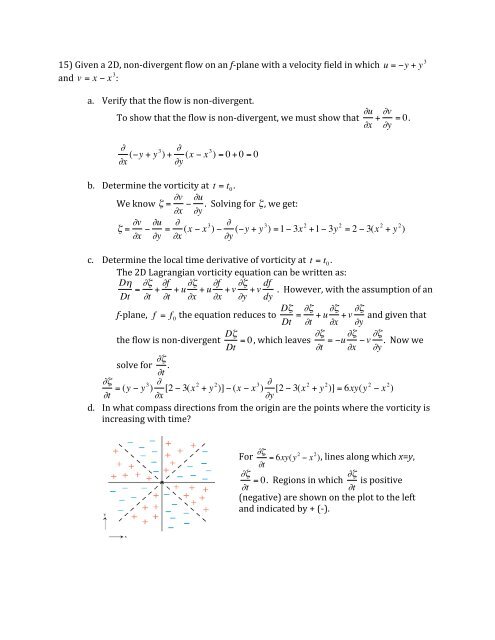
d. In what<br />
€<br />
compass directi<strong>on</strong>s from the origin are the points where the vorticity is <br />
increasing <strong>with</strong> time? <br />
€<br />
€<br />
€<br />
∂ζ<br />
For <br />
∂t = 6xy(y 2 − x 2 ), lines al<strong>on</strong>g which x=y, <br />
∂ζ<br />
∂ζ<br />
= 0. Regi<strong>on</strong>s in which is positive <br />
∂t ∂t<br />
(negative) are shown <strong>on</strong> the plot to the left <br />
<strong>an</strong>d indicated by + (-‐). <br />
€<br />
€
19) <str<strong>on</strong>g>Given</str<strong>on</strong>g> the ten inst<strong>an</strong>t<strong>an</strong>eous measurements below, solve for τ x<br />
= −ρ s<br />
u ʹ′ w ʹ′ , where <br />
ρ s<br />
=1.2kg m -‐3 . <br />
€<br />
u 8 6 11 14 13 9 8 9 <str<strong>on</strong>g>15</str<strong>on</strong>g> 12<br />
€<br />
w 0 1 0 -1 0 -1 -2 1 0 -2<br />
First calculate separately the average z<strong>on</strong>al <strong>an</strong>d vertical winds speeds to get <strong>an</strong>d u =10.5 <br />
ms -‐1 <strong>an</strong>d w = −0.4 ms -‐1 . Next calculate u ʹ′ , w ʹ′ , <strong>an</strong>d u ʹ′ w ʹ′ . <br />
€<br />
u' -2.5 -4.5 0.5 3.5 2.5 -1.5 -2.5 -1.5 4.5 1.5<br />
€<br />
w' 0.4 1.4 0.4 -0.6 0.4 -0.6 -1.6 1.4 0.4 -1.6<br />
u'w' -1 -6.3 0.2 -2.1<br />
€ €<br />
1<br />
€<br />
0.9 4 -2.1 1.8 -2.4<br />
Now we c<strong>an</strong> solve for u ʹ′ w ʹ′ , which u ʹ′ w ʹ′ = −0.6 . <br />
Finally, we have τ x<br />
= −ρ s<br />
u ʹ′ w ʹ′ = −1.2 kg m -3 (−0.6 m 2 s -2 ) = 0.72 (kg m s -2 )m -2 N m -‐2 . <br />
Note that we have solved for a stress – a t<strong>an</strong>gential force per unit area. <br />
€ €<br />
€