Cellular Systems Cellular Concepts The cellular concept was a ...
Cellular Systems Cellular Concepts The cellular concept was a ...
Cellular Systems Cellular Concepts The cellular concept was a ...
Create successful ePaper yourself
Turn your PDF publications into a flip-book with our unique Google optimized e-Paper software.
EE5401 <strong>Cellular</strong> Mobile Communications<br />
EE5401 <strong>Cellular</strong> Mobile Communications<br />
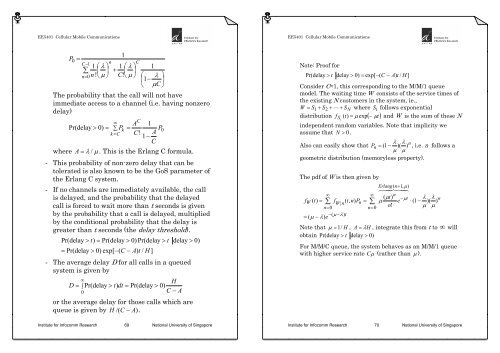
P<br />
0<br />
=<br />
C<br />
∑ − 1<br />
n=<br />
0<br />
1 ⎛ λ ⎞<br />
⎜ ⎟<br />
n!<br />
⎝ µ ⎠<br />
n<br />
1<br />
1 ⎛ λ ⎞<br />
+ ⎜ ⎟<br />
C!<br />
⎝ µ ⎠<br />
C<br />
1<br />
⎛ λ ⎞<br />
⎜1<br />
− ⎟<br />
⎝ µ C ⎠<br />
<strong>The</strong> probability that the call will not have<br />
immediate access to a channel (i.e. having nonzero<br />
delay)<br />
Pr( delay<br />
> 0) = ∑ ∞ P<br />
=<br />
k<br />
k C<br />
C<br />
A 1<br />
= P<br />
C!<br />
A<br />
1−<br />
C<br />
where A = λ / µ . This is the Erlang C formula.<br />
- This probability of non-zero delay that can be<br />
tolerated is also known to be the GoS parameter of<br />
the Erlang C system.<br />
- If no channels are immediately available, the call<br />
is delayed, and the probability that the delayed<br />
call is forced to wait more than t seconds is given<br />
by the probability that a call is delayed, multiplied<br />
by the conditional probability that the delay is<br />
greater than t seconds (the delay threshold).<br />
Pr(delay > t)<br />
= Pr(delay > 0) Pr(delay > t delay > 0)<br />
= Pr(delay > 0) exp[ −(<br />
C − A)<br />
t / H ]<br />
- <strong>The</strong> average delay D for all calls in a queued<br />
system is given by<br />
∞<br />
H<br />
D = ∫ Pr(delay > t)<br />
dt = Pr(delay > 0)<br />
0<br />
C − A<br />
or the average delay for those calls which are<br />
queue is given by H /( C − A)<br />
.<br />
0<br />
Note: Proof for<br />
Pr( delay > t delay > 0) = exp[ −(<br />
C − A)<br />
t / H ]<br />
Consider C=1, this corresponding to the M/M/1 queue<br />
model. <strong>The</strong> waiting time W consists of the service times of<br />
the existing N customers in the system, ie.,<br />
W = S1 + S2<br />
+ L+<br />
S N where S i follows exponential<br />
distribution fS ( t)<br />
= µ exp( − µ t)<br />
and W is the sum of these N<br />
i<br />
independent random variables. Note that implicity we<br />
assume that N > 0 .<br />
λ λ n<br />
Also can easily show that P n = (1 − )( ) , i.e. n follows a<br />
µ µ<br />
geometric distribution (memoryless property).<br />
<strong>The</strong> pdf of W is then given by<br />
Erlang(<br />
n+<br />
1, µ )<br />
64748 4<br />
∞<br />
∞ n<br />
( µ t)<br />
−µ<br />
t λ λ n<br />
fW<br />
( t)<br />
= ∑ f<br />
W | n<br />
( t,<br />
n)<br />
Pn<br />
= ∑ µ e ⋅ (1 − )( )<br />
n=<br />
0<br />
n=<br />
0 n!<br />
µ µ<br />
−(<br />
µ −λ)<br />
t<br />
= ( µ − λ)<br />
e<br />
Note that µ = 1/ H , A = λH<br />
, integrate this from t to ∞ will<br />
obtain Pr( delay > t delay > 0)<br />
For M/M/C queue, the system behaves as an M/M/1 queue<br />
with higher service rate C µ (rather than µ ).<br />
Institute for Infocomm Research 69 National University of Singapore<br />
Institute for Infocomm Research 70 National University of Singapore