COMPLEX GEOMETRY Course notes

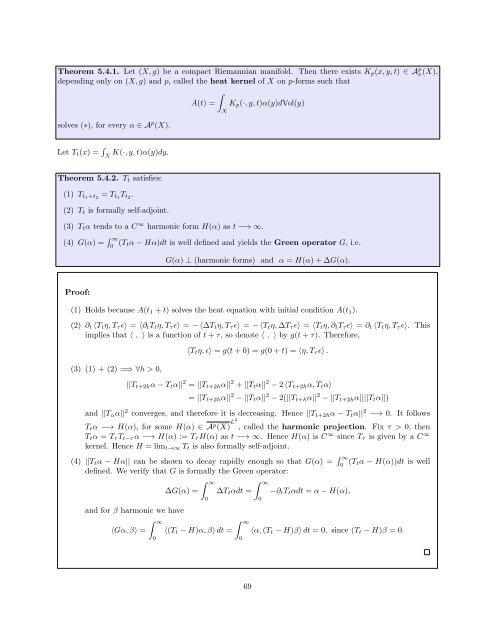
Theorem 5.4.1. Let (X, g) be a compact Riemannian manifold. Then there exists K p (x, y, t) ∈ A p x(X),
depending only on (X, g) and p, called the heat kernel of X on p-forms such that
∫
A(t) = K p (·, y, t)α(y)dVol(y)
solves (∗), for every α ∈ A p (X).
X
Let T t (x) = ∫ K(·, y, t)α(y)dy.
X
Theorem 5.4.2. T t satisfies:
(1) T t1+t 2
= T t1 T t2 .
(2) T t is formally self-adjoint.
(3) T t α tends to a C ∞ harmonic form H(α) as t −→ ∞.
(4) G(α) = ∫ ∞
0 (T tα − Hα)dt is well defined and yields the Green operator G, i.e.
G(α) ⊥ (harmonic forms) and α = H(α) + ∆G(α).
Proof:
(1) Holds because A(t 1 + t) solves the heat equation with initial condition A(t 1 ).
(2) ∂ t 〈T t η, T τ ɛ〉 = 〈∂ t T t η, T τ ɛ〉 = − 〈∆T t η, T τ ɛ〉 = − 〈T t η, ∆T τ ɛ〉 = 〈T t η, ∂ t T τ ɛ〉 = ∂ t 〈T t η, T τ ɛ〉. This
implies that 〈 , 〉 is a function of t + τ, so denote 〈 , 〉 by g(t + τ). Therefore,
(3) (1) + (2) =⇒ ∀h > 0,
〈T t η, ɛ〉 = g(t + 0) = g(0 + t) = 〈η, T τ ɛ〉 .
||T t+2h α − T t α|| 2 = ||T t+2h α|| 2 + ||T t α|| 2 − 2 〈T t+2h α, T t α〉
= ||T t+2h α|| 2 − ||T t α|| 2 − 2(||T t+h α|| 2 − ||T t+2h α||||T t α||)
and ||T α α|| 2 converges, and therefore it is decreasing. Hence ||T t+2h α − T t α|| 2 −→ 0. It follows
T t α −→ H(α), for some H(α) ∈ A p (X) L2 , called the harmonic projection. Fix τ > 0, then
T t α = T τ T t−τ α −→ H(α) := T τ H(α) as t −→ ∞. Hence H(α) is C ∞ since T τ is given by a C ∞
kernel. Hence H = lim t→∞ T t is also formally self-adjoint.
(4) ||T t α − Hα|| can be shown to decay rapidly enough so that G(α) = ∫ ∞
0 (T tα − H(α))dt is well
defined. We verify that G is formally the Green operator:
∆G(α) =
and for β harmonic we have
〈Gα, β〉 =
∫ ∞
0
∫ ∞
〈(T t − H)α, β〉 dt =
0
∆T t αdt =
∫ ∞
0
∫ ∞
0
−∂ t T t αdt = α − H(α),
〈α, (T t − H)β〉 dt = 0, since (T t − H)β = 0.
69