8-7 Similarity in Right Triangles 8-7 Similarity in Right Triangles

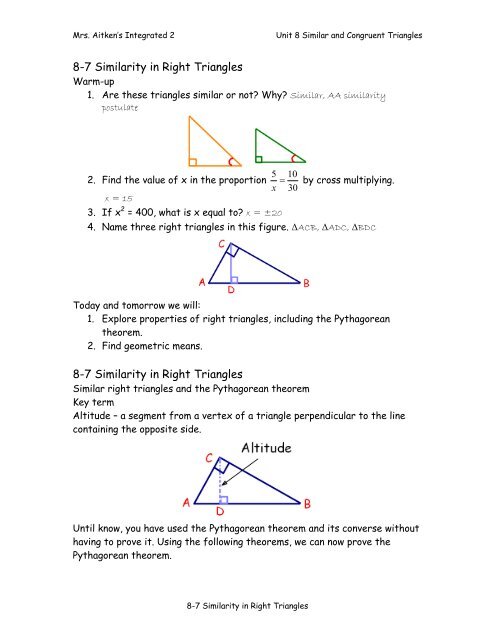
Mrs. Aitken’s Integrated 2
Unit 8 Similar and Congruent Triangles
8-7 Similarity in Right Triangles
Warm-up
1. Are these triangles similar or not? Why? Similar, AA similarity
postulate
2. Find the value of x in the proportion 5 = 10 by cross multiplying.
x 30
x = 15
3. If x 2 = 400, what is x equal to? x = ±20
4. Name three right triangles in this figure. ΔACB, ΔADC, ΔBDC
Today and tomorrow we will:
1. Explore properties of right triangles, including the Pythagorean
theorem.
2. Find geometric means.
8-7 Similarity in Right Triangles
Similar right triangles and the Pythagorean theorem
Key term
Altitude – a segment from a vertex of a triangle perpendicular to the line
containing the opposite side.
Until know, you have used the Pythagorean theorem and its converse without
having to prove it. Using the following theorems, we can now prove the
Pythagorean theorem.
8-7 Similarity in Right Triangles

Mrs. Aitken’s Integrated 2
Unit 8 Similar and Congruent Triangles
Similar Right Triangles theorem
If the altitude is drawn to the hypotenuse of a right triangle, then the two
triangles formed are similar to the original triangle and to each other.
C
So, ▲ABC ~ ▲ACD ~ ▲CBD
A
D
B
The Pythagorean Theorem
In any right triangle, the square of the length of the hypotenuse is equal to
the sum of the squares of the lengths of the legs.
c 2 = a 2 + b 2
Example 1
In ΔABC, BD is an altitude to hypotenuse AC. Identify the similar triangles.
Solution 1
ΔACB ~ ΔABD ~ΔBCD
8-7 Similarity in Right Triangles

Mrs. Aitken’s Integrated 2
Unit 8 Similar and Congruent Triangles
Example 2
Find the measure of each unknown side.
a. b.
Solution 2
Use the Pythagorean Theorem because these are right triangles!
a. a 2 + b 2 = c 2 b. a 2 + b 2 = c 2
5 2 +5 2 = c 2 x 2 +x 2 = 4 2
25 + 25 = c 2 2x 2 = 16
50 = c 2 x 2 =8
c = 7.1 x = 2.8
Example 3
In right ΔABC, CD is the altitude to the hypotenuse AB. The measure of

Mrs. Aitken’s Integrated 2
Unit 8 Similar and Congruent Triangles
Example 4
Write a two-column proof of the Pythagorean theorem
Given: ΔABC with right angle C,
Prove: c 2 = a 2 + b 2
Solution 4
Plan ahead
Draw the altitude of ΔABC from vertex C and label the point of intersection on AB as
point D. Use the similar right triangle theorem to identify similar triangles. Then use
the definition of similar triangles to write proportions involving the variables a, b, and
c. Use algebra to obtain c 2 = a 2 + b 2 .
As an aid, show the corresponding sides of the three similar right triangles.
Statement
1. ΔABC with right angle C 1. Given
2. Draw altitude from C to point
D on AB
ΔABC ~ ΔACD
ΔABC ~ ΔCBD
Justification
2. If the altitude is drawn to the hypotenuse
of a right triangle, then the two
triangles formed are similar to the
original triangle and to each other.
c b c a
3. Definition of similar triangles.
3. = ; =
b f a e
Corresponding sides are in proportion.
4. ce = a 2 ; cf = b 2 4. Multiplication property of equality
5. cf + ce = a 2 + b 2 5. Addition property of equality
6. c(f + e) = a 2 + b 2 6. Distributive property of equality
7. e + f = c 7. For any segment, the measure of the
whole is equal to the sum of the measures
of its non-overlapping parts
8. c(c) = a 2 + b 2 or c 2 = a 2 + b 2 8. Substitution property (Steps 6 & 7)
8-7 Similarity in Right Triangles

Mrs. Aitken’s Integrated 2
Unit 8 Similar and Congruent Triangles
Geometric Mean
The Geometric mean of two numbers, a and b, is the positive square root of
their product, √ab.
The geometric mean can be expressed as a proportion. The value of x
(geometric mean) in the proportion
a x
= , where a, b, and x are positive numbers. x is the geometric mean between
x b
a and b
4 6
Example: 6 is the geometric mean between 4 and 9, since =
6 9 .
The Arithmetic mean of two numbers, a and b, is the number half-way between the
two numbers, (a + b)/2.
The geometric mean is always positive.
Example 1
Find the geometric mean between 2 and 72.
Solution 1
a x
Use =
x b .
2 x
So, = , solve by cross multiplying
x 72
2• 72 = x • x
2
144 = x
144 =
x = 12
2
x
The geometric mean is 12.
Example 2
10 is the geometric mean between 25 and what other number?
Solution 2
a x
Use =
x b .
8-7 Similarity in Right Triangles

Mrs. Aitken’s Integrated 2
Unit 8 Similar and Congruent Triangles
25 10
So, = , solve by cross multiplying
10 b
25 10
=
10 b
25b
= 100
b = 4
So, 10 is the geometric mean between 4 and 25.
Geometric Mean Theorem
If the altitude is drawn to the hypotenuse of a right triangle, then the
measure of the altitude is the geometric mean between the measures of the
parts of the hypotenuse.
C
AD
CD
CD
=
BD
A
D
B
Example 3
Find the lengths x, y, and z.
Solution 3
First find x. Use the geometric mean theorem to write a
proportion involving x because x is the altitude of ΔABC.
6.5 x
=
x 26
2
x = 169
x = 13
Use the value of x and the Pythagorean theorem to find y.
2 2 2
y = x + 26
2 2 2
y = 13 + 26
2
y = 845
y ≈ 29.1
Use the value of x and the Pythagorean theorem to find z.
8-7 Similarity in Right Triangles

Mrs. Aitken’s Integrated 2
Unit 8 Similar and Congruent Triangles
z = x + 6.5
2 2 2
z = 13 + 6.5
2 2 2
2
z = 211.25
z = 14.5
The lengths of x, y, and z are 13, about 29.1, and about 14.5.
Example 4
Find the lengths of m, n, and p.
Solution 4
a x
=
x b
First find m.
12 m
=
m 3
2
m = 36
2
m =
m = 6
36
Use the Pythagorean theorem to find n and p.
2 2
12 + 6
2
= p
2 2
6 + 3
2
= n
2
144+ 36 = p
2
180 = p
2
36 + 9 = n
2
45 = n
180 =
2
p
p = 6 5 = 13.4
2
45 = n
n = 3 5 = 6.7
8-7 Similarity in Right Triangles