Tunneling
Tunneling
Tunneling
You also want an ePaper? Increase the reach of your titles
YUMPU automatically turns print PDFs into web optimized ePapers that Google loves.
Chemistry 460<br />
Spring 2013<br />
Dr. Jean M. Standard<br />
February 5, 2013<br />
<strong>Tunneling</strong><br />
Definition of <strong>Tunneling</strong><br />
<strong>Tunneling</strong> is defined to be penetration of the wavefunction into a classically forbidden region. Since the<br />
wavefunction is non-zero in the classically forbidden region, the probability density ψ *ψ also is non-zero; thus,<br />
there is a finite probability that the particle will exist in a region that it could never be in if it were obeying classical<br />
mechanics. In this situation, we say that the particle has tunneled into the classically forbidden region. [A region is<br />
said to be classically forbidden if the particle’s energy is less than the potential energy in the region, E < V .]<br />
€<br />
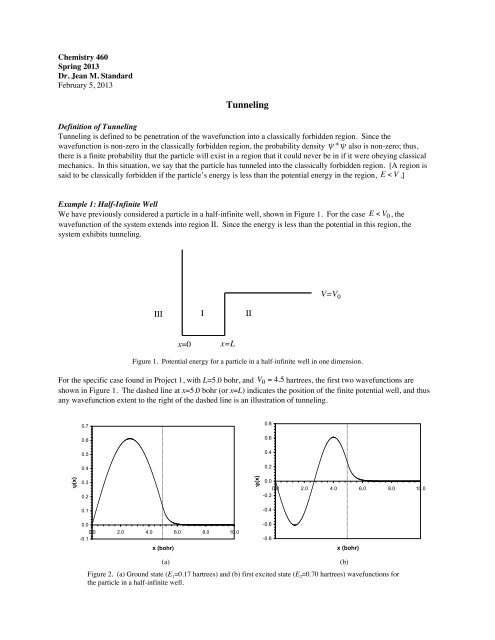
Example 1: Half-Infinite Well<br />
€<br />
We have previously considered a particle in a half-infinite well, shown in Figure 1. For the case E < V 0 , the<br />
wavefunction of the system extends into region II. Since the energy is less than the potential in this region, the<br />
system exhibits tunneling.<br />
€<br />
III I II<br />
V=V 0<br />
x=0 x=L<br />
Figure 1. Potential energy for a particle in a half-infinite well in one dimension.<br />
For the specific case found in Project 1, with L=5.0 bohr, and V 0 = 4.5 hartrees, the first two wavefunctions are<br />
shown in Figure 1. The dashed line at x=5.0 bohr (or x=L) indicates the position of the finite potential well, and thus<br />
any wavefunction extent to the right of the dashed line is an illustration of tunneling.<br />
0.7<br />
€<br />
0.8<br />
0.6<br />
0.6<br />
0.5<br />
0.4<br />
0.4<br />
0.2<br />
ψ(x)<br />
0.3<br />
0.2<br />
ψ(x)<br />
0.0<br />
0.0 2.0 4.0 6.0 8.0 10.0<br />
-0.2<br />
0.1<br />
-0.4<br />
0.0<br />
0.0 2.0 4.0 6.0 8.0 10.0<br />
-0.1<br />
x (bohr)<br />
-0.6<br />
-0.8<br />
x (bohr)<br />
(a) (b)<br />
Figure 2. (a) Ground state (E 1 =0.17 hartrees) and (b) first excited state (E 2 =0.70 hartrees) wavefunctions for<br />
the particle in a half-infinite well.
2<br />
For energies closer to the top of the well, the tunneling proportion increases. Shown in Figure 3 are the<br />
wavefunctions corresponding to the fourth and fifth energy levels for this system.<br />
0.8<br />
0.8<br />
0.6<br />
0.6<br />
0.4<br />
0.4<br />
0.2<br />
0.2<br />
ψ(x)<br />
0.0<br />
0.0 2.0 4.0 6.0 8.0 10.0<br />
-0.2<br />
ψ(x)<br />
0.0<br />
0.0 2.0 4.0 6.0 8.0 10.0<br />
-0.2<br />
-0.4<br />
-0.4<br />
-0.6<br />
-0.6<br />
-0.8<br />
-0.8<br />
x (bohr)<br />
x (bohr)<br />
(a) (b)<br />
Figure 3. (a) Third excited state (E 4 =2.75 hartrees) and (b) fourth excited state (E 5 =4.16 hartrees)<br />
wavefunctions for the particle in a half-infinite well.<br />
Notice that for the fourth excited state, with an energy very close to the top of the barrier (E 5 =4.16 hartrees<br />
compared to a barrier of 4.5 hartrees), the tunneling is substantial. For this system, we can determine the tunneling<br />
probability by integrating the probability density from x=L to x= ∞. For the 1 st energy level the tunneling probability<br />
is 0.0025, while for the 5 th energy level the tunneling probability is 0.18.<br />
€<br />
Example 2: <strong>Tunneling</strong> Through a Barrier of Finite Width<br />
Another simple example of tunneling is the case of a barrier of finite width, illustrated in Figure 4.<br />
V=V 0<br />
I II III<br />
x=0 x=L<br />
Figure 4. Potential energy for a particle interacting with a finite barrier in one dimension.<br />
The potential energy of the system may be described by the following equation,<br />
V (x) =<br />
⎧⎧ 0,<br />
⎪⎪<br />
⎨⎨ V 0 ,<br />
⎪⎪<br />
⎩⎩ 0,<br />
x < 0<br />
0 ≤ x ≤ L<br />
x > L<br />
⎫⎫<br />
⎪⎪<br />
⎬⎬ .<br />
⎪⎪<br />
⎭⎭<br />
The method of solution is similar to what we did for the particle in a half-infinite well. However, there are two<br />
degenerate solutions, one in which the particle is initially traveling to the right and one in which the particle is<br />
€<br />
initially traveling to the left. Only one of these degenerate cases needs to be considered. Here, we will assume that<br />
the particle is traveling to the right, so it is initially incident on the barrier from region I.
3<br />
For the case<br />
E < V 0 , the general solutions may be given as<br />
€<br />
ψ I ( x) = A e ik1x + Be −ik 1x<br />
ψ II ( x) = C e k2x + De −k 2x<br />
ψ III ( x) = F e ik 1 x .<br />
The wave vectors<br />
k 1 and<br />
k 2 are defined as<br />
€<br />
€<br />
€<br />
k 1 =<br />
2mE<br />
<br />
and k 2 =<br />
2m ( V 0 − E)<br />
<br />
.<br />
In region I, and in general for unbounded regions where the potential energy is zero, we use exponentials with<br />
imaginary exponents as the preferred form of the wavefunction. The two parts of the wavefunction written in this<br />
€<br />
form can be related to waves traveling in the positive and negative x directions, respectively.<br />
Notice that in region II, we have used exponentials with real rather than imaginary exponents. This is the preferred<br />
form in regions where the total energy E is less than the potential energy V 0 .<br />
Also notice that in region III, there is no wave traveling to the left. This is because we started with a wave traveling<br />
to the right, and in region III, no wave traveling to the left can form because there is nothing for the wave to reflect<br />
€<br />
from.<br />
Matching the wavefunctions and their first derivatives at the boundaries x=0 and x=L yields conditions among the<br />
arbitrary constants A, B, C, D, and F. Of particular interest is a quantity called the transmission coefficient T. The<br />
transmission coefficient T is related to the ratio of the probability density current that is transmitted through the<br />
barrier to the incident probability density current. The probability density current S is defined as<br />
S = v ψ *ψ ,<br />
where v is the particle velocity. Thus, the transmission coefficient T is<br />
€<br />
T = S tr<br />
S in<br />
,<br />
€<br />
where S tr is the transmitted probability density current and S in is the incident probability density current. For the<br />
specific case here, the transmitted wave in region € III has the form Fe i k 1x . Since this form has a momentum<br />
eigenvalue given by k 1 , the velocity is<br />
€<br />
v € tr = p tr<br />
m = k 1<br />
m .<br />
€<br />
Similarly, the incident wave in region I has the form<br />
k 1 , the velocity is<br />
€<br />
Ae i k 1x . Since this form has a momentum eigenvalue given by<br />
€<br />
€<br />
v in = p in<br />
m = k 1<br />
m .<br />
€
4<br />
Substituting, we can calculate the transmission coefficient T,<br />
T = S tr<br />
S in<br />
= v trψ * tr ψ tr<br />
v in ψ * in ψ in<br />
=<br />
T = F A<br />
k 1<br />
m F * e−ik 1x Fe<br />
ik 1 x<br />
k 1<br />
m A * e−ik 1 x Ae ik 1 x<br />
2<br />
.<br />
Using the relations obtained from matching the wavefunctions and first derivatives at the boundaries, the ratio of<br />
F/A may be determined. For this system, the transmission coefficient is<br />
€<br />
T = F A<br />
2<br />
,<br />
or T =<br />
1 +<br />
1<br />
sinh 2 .<br />
( k 2 L)<br />
4E ⎛⎛<br />
1 − E ⎞⎞<br />
⎜⎜ ⎟⎟<br />
V 0 ⎝⎝ V 0 ⎠⎠<br />
In this equation, the function sinh is the hyperbolic sine function. It is defined<br />
€<br />
sinhbx = ebx − e −bx<br />
.<br />
2<br />
Though not appearing in this expression, the hyperbolic cosine function, cosh, is defined in a similar fashion,<br />
€<br />
coshbx = ebx + e −bx<br />
.<br />
2<br />
For values of the energy less than V 0 , the transmission coefficient only gets large for energies close to the top of the<br />
barrier, as illustrated in Figure 5.<br />
€<br />
€<br />
0.020<br />
0.018<br />
T<br />
0.016<br />
0.014<br />
0.012<br />
0.010<br />
0.008<br />
0.006<br />
0.004<br />
0.002<br />
0.000<br />
0.0 0.2 0.4 0.6 0.8 1.0 1.2<br />
E/V 0<br />
Figure 5. Transmission coefficient for a particle tunneling through a finite barrier in one dimension.
5<br />
Example 3: Double Well Potential<br />
A common model that is used to represent inversion in molecules like ammonia is the double well potential shown<br />
in Figure 6.<br />
V=V 0<br />
L<br />
x=0<br />
x=a x=c x=d<br />
Figure 6. Double well potential.<br />
This potential mimics the inversion of ammonia through its planar form, illustrated in Figure 7.<br />
(a)<br />
(b)<br />
Figure 7. (a) Inversion motion of ammonia through the planar transition state. (b) Potential energy of<br />
inversion of ammonia as a function of the out-of-plane angle.<br />
Classically, the ammonia molecule in its ground state does not have enough energy to go over the barrier.<br />
Therefore, a classical ammonia molecule would not experience inversion. In quantum mechanics, though, the<br />
ammonia molecule experiences tunneling through the barrier and so undergoes inversion.