Galois Theory: A Study of Cyclotomic Field ... - Scripps College
Galois Theory: A Study of Cyclotomic Field ... - Scripps College
Galois Theory: A Study of Cyclotomic Field ... - Scripps College
You also want an ePaper? Increase the reach of your titles
YUMPU automatically turns print PDFs into web optimized ePapers that Google loves.
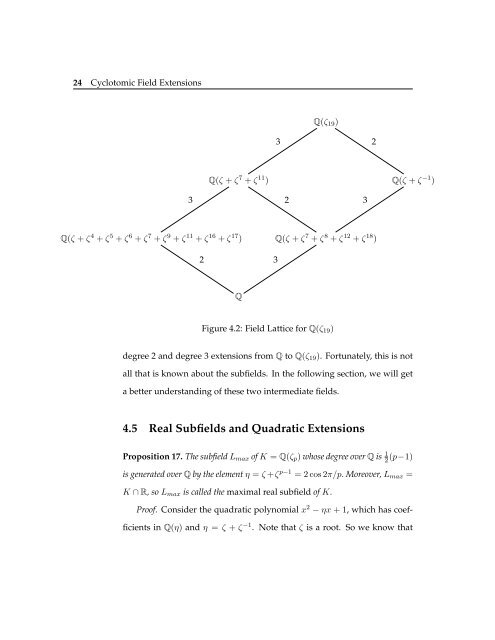
24 <strong>Cyclotomic</strong> <strong>Field</strong> Extensions<br />
Q(ζ 19 )<br />
3<br />
2<br />
Q(ζ + ζ 7 + ζ 11 )<br />
Q(ζ + ζ −1 )<br />
3 2<br />
3<br />
Q(ζ + ζ 4 + ζ 5 + ζ 6 + ζ 7 + ζ 9 + ζ 11 + ζ 16 + ζ 17 )<br />
2<br />
Q(ζ + ζ 7 + ζ 8 + ζ 12 + ζ 18 )<br />
3<br />
Q<br />
Figure 4.2: <strong>Field</strong> Lattice for Q(ζ 19 )<br />
degree 2 and degree 3 extensions from Q to Q(ζ 19 ). Fortunately, this is not<br />
all that is known about the subfields. In the following section, we will get<br />
a better understanding <strong>of</strong> these two intermediate fields.<br />
4.5 Real Subfields and Quadratic Extensions<br />
Proposition 17. The subfield L max <strong>of</strong> K = Q(ζ p ) whose degree over Q is 1 2 (p−1)<br />
is generated over Q by the element η = ζ +ζ p−1 = 2 cos 2π/p. Moreover, L max =<br />
K ∩ R, so L max is called the maximal real subfield <strong>of</strong> K.<br />
Pro<strong>of</strong>. Consider the quadratic polynomial x 2 − ηx + 1, which has coefficients<br />
in Q(η) and η = ζ + ζ −1 . Note that ζ is a root. So we know that