Chapter 4 - UCSB HEP

WORK AND ENERGY
Example 4.8
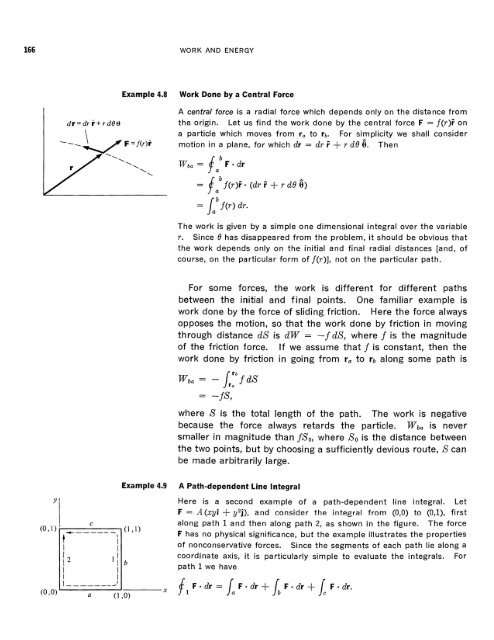
Work Pone by a Central Force
A central force is a radial force which depends only on the distance from
dr=dri+rd@t) the origin. Let us find the work done by the central force F = f(r)F on
\ a particle which moves from r, to rb. For simplicity we shall consider
- f F=fIr)i motion in a wlane. for which dr = dr + r dB 6. Then
= f 'f(r)i. (dr i 4- T dB 6)
a
The work is given by a simple one dimensional integral over the variable
T. Since 8 has disappeared from the problem, it should be obvious that
the work depends only on the initial and final radial distances [and, of
course, on the particular form of f(r)], not on the particular path.
For some forces, the work is different for different paths
between the initial and final points. One familiar example is
work done by the force of sliding friction. Here the force always
opposes the motion, so that the work done by friction in moving
through distance dS is dW = -f dS, where f is the magnitude
of the frEction force. If we assume that f is constant, then the
work done by friction in going from r, to rb along some path is
where S is the total length of the path. The work is negative
because the force always retards the particle. Wa, is never
smaller in magnitude than fSo, where So is the distance between
the two points, but by choosing a sufficiently devious route, 8 can
be made arbitrarily large.
(QJI
Example 4.9
A Path-dependent Line Integral
Here is a second example of a path-dependent line integral. Let
F = A(q0 -j- p2j), and consider the integral from (0,O) to (O,I), first
1 c along path 1 and then along path 2, as shown in the figure. The force
------- I(1,l)
I
1
F has no physical significance, but the example illustrates the properties
I.
I
of nonconservative forces. Since the segments of each path lie along a
coordinate axis, it is particularly simple to evaluate the integrals. For
I 1 : b
1 I
path 1 we have