Alain Connes (pdf)

More documents

Recommendations

Info

$Øvelse 1: Få LATEX op at stå Øvelse 2: At skrive matematiske ...$

character of the KO-homology fundamental class contains all the rational information on the Pontrjagin classes. The characteristic property of differentiable manifolds which is carried over to the noncommutative case is Poincaré duality in KO-homology. Moreover, as we saw above in the discussion of topology, K-homology admits a fairly simple definition in terms of Hilbert space and Fredholm representations of algebras, as gradually emerged from the work of Atiyah, Singer, Brown-Douglas-Fillmore, Miscenko, and Kasparov. For an ordinary manifold the choice of the fundamental cycle in K-homology is a refinement of the choice of orientation of the manifold and in its simplest form is a choice of spin structure. Of course the role of a spin structure is to allow for the construction of the corresponding Dirac operator which gives a corresponding Fredholm representation of the algebra of smooth functions. The origin of the construction of the Dirac operator was the extraction of a square root for a second order differential operator like the Laplacian. What is rewarding is that this will not only guide us towards the notion of noncommutative manifold but also to a formula, of operator theoretic nature, for the line element ds. In the Riemannian case one gives the Taylor expansion of the square ds 2 of the infinitesimal line element, in our framework the extraction of square root effected by the Dirac operator allows us to deal directly with ds itself. 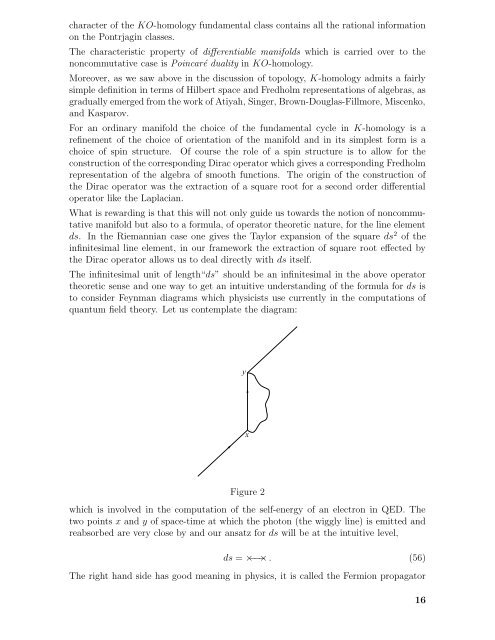The infinitesimal unit of length“ds” should be an infinitesimal in the above operator theoretic sense and one way to get an intuitive understanding of the formula for ds is to consider Feynman diagrams which physicists use currently in the computations of quantum field theory. Let us contemplate the diagram: y x Figure 2 which is involved in the computation of the self-energy of an electron in QED. The two points x and y of space-time at which the photon (the wiggly line) is emitted and reabsorbed are very close by and our ansatz for ds will be at the intuitive level, ds = ×−−−× . (56) The right hand side has good meaning in physics, it is called the Fermion propagator 16
and is given by where D is the Dirac operator. We thus arrive at the following basic ansatz, ×−−−× = D −1 (57) ds = D −1 . (58) In some sense it is simpler than the ansatz giving ds 2 as g µν dx µ dx ν , the point being that the spin structure allows really to extract the square root of ds 2 (as is well known Dirac found the corresponding operator as a differential square root of a Laplacian). The first thing we need to do is to check that we are still able to measure distances with our “unit of length” ds. In fact we saw in the discussion of the quantized calculus that variables with continuous range cant commute with “infinitesimals” such as ds and it is thus not very surprising that this lack of commutativity allows to compute, in the classical Riemannian case, the geodesic distance d(x, y) between two points. The precise formula is d(x, y) = Sup {|f(x) − f(y)| ; f ∈ A , ‖[D, f]‖ ≤ 1} (59) where D = ds −1 as above and A is the algebra of smooth functions. Note that ds has the dimension of a length L, D has dimension L −1 and the above expression for d(x, y) also has the dimension of a length. Thus we see in the classical geometric case that both the fundamental cycle in K- homology and the metric are encoded in the spectral triple (A, H, D) where A is the algebra of functions acting in the Hilbert space H of spinors, while D is the Dirac operator. To get familiar with this notion one should check that one recovers the volume form of the Riemannian metric by the equality (valid up to a normalization constant [12]) ∫ ∫ −f |ds| n = f √ g d n x (60) M n but the first interesting point is that besides this coherence with the usual computations there are new simple questions we can ask now such as ”what is the two-dimensional measure of a four manifold” in other words ”what is its area ” Thus one should compute ∫ − ds 2 (61) It is obvious from invariant theory that this should be proportional to the Hilbert– Einstein action, the direct computation was done by D. Kastler and gives, ∫ − ds 2 = −1 ∫ r √ g d 4 x (62) 48π 2 M 4 where as above dv = √ g d 4 x is the volume form, ds = D −1 the length element, i.e. the inverse of the Dirac operator and r is the scalar curvature. In the general framework of Noncommutative Geometry the confluence of the Hilbert space incarnation of the two notions of metric and fundamental class for a manifold lead very naturally to define a geometric space as given by a spectral triple: (A, H, D) (63) 17
Page 1 and 2: NONCOMMUTATIVE GEOMETRY Alain Conne
Page 3 and 4: topological spaces. They are given
Page 5 and 6: i.e. X is the quotient of S 1 by th
Page 7 and 8: and the δ j are still derivations
Page 9 and 10: What I mean is that it admits a god
Page 11 and 12: Lie groups. He also gave the first
Page 13 and 14: Of course such a dictionary that tr
Page 15: In practice the choice of the limit
Page 19 and 20: There is a very important test of o
Page 21 and 22: in [18], encoded by a spectral trip
Page 23 and 24: [11] A. Connes: Cyclic cohomology a

Alain Connes (pdf)

Create successful ePaper yourself

Delete template?

Save as template?