Version One â Homework 1 â Juyang Huang â 24018 â Jan 16 ...

Version One – Homework 1 – Juyang Huang – 24018 – Jan 16, 2008 1 

This print-out should have 66 questions. 

Multiple-choice questions may continue on 

the next column or page – find all choices 

before answering. The due time is Central 

time. 

Charge in Lightning 03 

23:01, trigonometry, numeric, > 1 min, normal. 

001 (part 1 of 1) 10 points 

A strong lightning bolt transfers about 25 C 

to Earth. 

The charge on an electron is 1.60218 × 

10 −19 C. 

How many electrons are transferred 

Correct answer: 1.56038 × 10 20 . 

Explanation: 

Let : q = 25 C . 

The charge is proportional to the number 

of electrons, so 

keywords: 

q = n q e 

n = q q e 

−25 C 

= 

−1.60218 × 10 −19 C 

= 1.56038 × 10 20 . 

AP EM 1993 MC 55 

23:04, trigonometry, multiple choice, < 1 min, 

fixed. 


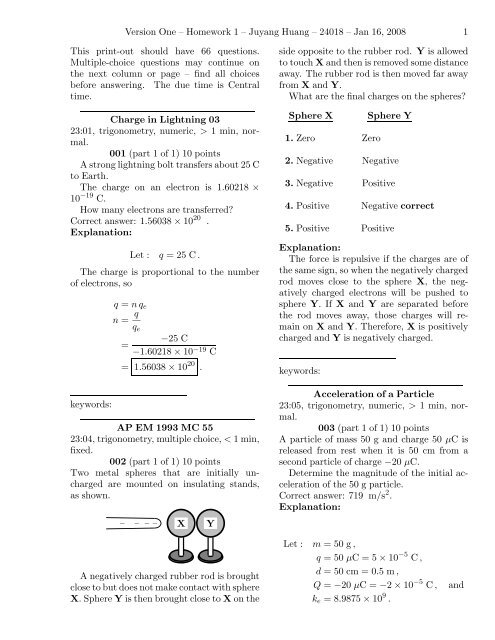
Two metal spheres that are initially uncharged 

are mounted on insulating stands, 

as shown. 

− 

− 

− − 

X 

Y 

side opposite to the rubber rod. Y is allowed 

to touch X and then is removed some distance 

away. The rubber rod is then moved far away 

from X and Y. 

What are the final charges on the spheres 

Sphere X 

1. Zero Zero 

Sphere Y 

2. Negative Negative 

3. Negative Positive 

4. Positive Negative correct 

5. Positive Positive 

Explanation: 

The force is repulsive if the charges are of 

the same sign, so when the negatively charged 

rod moves close to the sphere X, the negatively 

charged electrons will be pushed to 

sphere Y. If X and Y are separated before 

the rod moves away, those charges will remain 

on X and Y. Therefore, X is positively 

charged and Y is negatively charged. 

keywords: 

Acceleration of a Particle 



A particle of mass 50 g and charge 50 µC is 

released from rest when it is 50 cm from a 

second particle of charge −20 µC. 

Determine the magnitude of the initial acceleration 

of the 50 g particle. 

Correct answer: 719 m/s 2 . 

Explanation: 

A negatively charged rubber rod is brought 

close to but does not make contact with sphere 

X. Sphere Y is then brought close to X on the 

Let : m = 50 g , 

q = 50 µC = 5 × 10 −5 C , 

d = 50 cm = 0.5 m , 

Q = −20 µC = −2 × 10 −5 C , 

k e = 8.9875 × 10 9 . 

and


The force exerted on the particle is 

F = k e 

|q 1 | |q 2 | 

r 2 

‖⃗a‖ = k e 

‖⃗q‖ ‖ ⃗ Q‖ 

m d 2 

keywords: 

= m a 

= k e 

∣ ∣ 5 × 10 −5 C ∣ ∣ ∣ ∣−2 × 10 −5 C ∣ ∣ 

(0.05 kg) (0.5 m 2 ) 

= 719 m/s 2 . 

Hanging Charges 



Two identical small charged spheres hang in 

equilibrium with equal masses as shown in 

the figure. The length of the strings are equal 

and the angle (shown in the figure) with the 

vertical is identical. 

The acceleration of gravity is 9.8 m/s 2 

and the value of Coulomb’s constant is 

8.98755 × 10 9 N m 2 /C 2 . 

q 

m 

θ 

a 

L 

q 

m 

From the right triangle in the figure above, 

we see that 

sin θ = a L . 

Therefore 

a = L sin θ 

= (0.15 m) sin(5 ◦ ) 

= 0.0130734 m . 

The separation of the spheres is r = 2 a = 

0.0261467 m . The forces acting on one of the 

spheres are shown in the figure below. 

T cos θ 

F 

e 

θ 

mg 

T 

θ 

T sin θ 

Because the sphere is in equilibrium, the 

resultant of the forces in the horizontal and 

vertical directions must separately add up to 

zero: 

∑ 

Fx = T sin θ − F e = 0 

0.15 m 

∑ 

Fy = T cos θ − m g = 0 . 

5 ◦ 

0.03 kg 0.03 kg 

Find the magnitude of the charge on each 

sphere. 

Correct answer: 4.4233 × 10 −8 C. 

Explanation: 

Let : L = 0.15 m , 

m = 0.03 kg , 

θ = 5 ◦ . 

and 

From the second equation in the system 

above, we see that T = 

m g , so T can be 

cos θ 

eliminated from the first equation if we make 

this substitution. This gives a value 

F e = m g tan θ 

= (0.03 kg) ( 9.8 m/s 2) tan(5 ◦ ) 

= 0.0257217 N , 

for the electric force. 

From Coulomb’s law, the electric force between 

the charges has magnitude 

|F e | = k e 

|q| 2 

r 2 ,


where |q| is the magnitude of the charge on 

each sphere. 

Note: The term |q| 2 arises here because the 

charge is the same on both spheres. 

This equation can be solved for |q| to give 

√ 

|F e | r 

|q| = 

2 

= 

keywords: 

k e 

√ 

(0.0257217 N) (0.0261467 m) 2 

(8.98755 × 10 9 N m 2 /C 2 ) 

= 4.4233 × 10 −8 C . 

Serway CP 15 11 



Three charges are arranged in a triangle as 

shown. 

The Coulomb constant is 8.98755 × 

10 9 N · m 2 /C 2 . 

y 

5 nC 

+ 

− 

−3 nC 

0.3 m 

0.1 m 

+ 

6 nC 

What is the net electrostatic force on the 

charge at the origin 

Correct answer: 1.38102 × 10 −5 N. 

Explanation: 

x 

F 1,2 θ 

F 1,3 

F 

The repulsive force 

q 1 q 2 

F 1,2 = k C 

r1,2 

2 

= 8.98755 × 10 9 N · m 2 /C 2 

( 

5 × 10 −9 C ) ( 6 × 10 −9 C ) 

× 

(0.3 m) 2 

= 2.99585 × 10 −6 N 

acts along the negative x-axis, and the attractive 

force 

q 1 |q 3 | 

F 1,3 = k C 

r1,3 

2 

= 8.98755 × 10 9 N · m 2 /C 2 

( 

5 × 10 −9 C ) ( −3 × 10 −9 C ) 

× 

(0.1 m) 2 

= −1.34813 × 10 −5 N 

acts along the negative y-axis. 

Thus 

F net = [ (2.99585 × 10 −6 N) 2 

+(−1.34813 × 10 −5 N) 2] 1/2 

= 1.38102 × 10 −5 N . 

Let : q 1 = 5 nC = 5 × 10 −9 C , 

q 2 = 6 nC = 6 × 10 −9 C , 

q 3 = −3 nC = −3 × 10 −9 C , 

r 1,2 = 0.3 m , 

r 1,3 = 0.1 m , and 

k C = 8.98755 × 10 9 N · m 2 /C 2 . 


What is the direction of this force (as an angle 

between −180 ◦ and 180 ◦ measured from the 

positive x-axis, with counterclockwise positive) 

Correct answer: −102.529 ◦ . 

Explanation:


The electric field strength E ∝ 1 r 2 , so 

tan θ = F 1,3 

F 1,2 

( ) 

θ = tan −1 F1,3 

F 1,2 

( 1.34813 × 10 

= tan −1 −5 ) 

N 

2.99585 × 10 −6 N 

= 77.4712 ◦ 

E A 

E B 

= 

keywords: 

1 

r 2 A 

1 

r 2 B 

= r2 B 

r 2 A 

= 

(2 r)2 

r 2 = 4 . 

below the negative x-axis. From the positive 

x-axis, the angle is 

−180 ◦ + 77.4712 ◦ = −102.529 ◦ . 

Two Charge Field 

23:13, trigonometry, multiple choice, > 1 min, 

wording-variable. 


Two point-charges at fixed locations produce 

an electric field as shown below. 

keywords: 

AP B 1993 MC 68 


fixed. 


The diagram shows an isolated, positive 

charge Q, where point B is twice as far away 

from Q as point A. 

A 

X 

B 

+Q A B 

Y 

0 10 cm 20 cm 

The ratio of the electric field strength at 

point A to the electric field strength at point 

B is 

1. E A 

E B 

= 8 1 . 

2. E A 

E B 

3. E A 

E B 

= 2 1 . 

4. E A 

E B 

= 1 1 . 

5. E A 

E B 

= 1 2 . 

Explanation: 

= 4 1 . correct 

Let : r B 

= 2 r A 

. 

A negative charge placed at point X would 

move 

1. toward charge B. correct 

2. toward charge A. 

3. along an equipotential plane. 

Explanation: 

The electric field runs from a positive potential 

to a negative potential, so it points 

from a positive charge to a negative charge. 

Therefore the charge B is positive. A negative 

charge will move toward a positive potential, 

which creates lower potential energy and a 

higher kinetic energy. 


The electric field at point X is


1. stronger than the field at point Y . correct 

2. weaker than the field at point Y . 

3. the same as that the field at point Y . 

Explanation: 

The field at X is stronger than the field 

at Y , since the number of field lines per unit 

volume at X is greater than the number of 

field lines per unit volume at Y . 


Estimate the ratio of the magnitude of 

charge A to the magnitude of charge B. Your 

answer must be within ± 5%. 

Correct answer: 1.88889 . 

Explanation: 

The number of field lines is proportional to 

the magnitude of the charge. 

keywords: 

Q A 

≈ −17 = −1.88889 

Q B 9 

∣ Q A ∣∣∣ 

∣ ≈ 1.88889 . 

Q B 

Maximum force on one charge 

23:05, calculus, multiple choice, > 1 min, 

fixed. 


Charge Q is on the y axis a distance a from 

the origin and charge q is on the x axis a 

distance d from the origin. 

What is the value of d for which the x 

component of the force on q is the greatest 

1. d = 0 

2. d = a 

3. d = √ 2 a 

4. d = a 2 

5. d = a √ 

2 

correct 

6. d = q Q a 

7. d = q Q 

√ 

2 a 

8. d = q a 

Q 2 

9. d = q a 

√ 

Q 2 

Explanation: 

We have the force on charge q on the x axis 

due to charge Q on the y axis 

⃗F = 1 

4 π ɛ 0 

q Q 

r 2 ˆr , 

where r = √ a 2 + d 2 . So the x component of 

the force on q is 

F x = 1 q Q 

4 π ɛ 0 r 2 cos θ 

= 1 

4 π ɛ 0 

q Q 

a 2 + d 2 

d 

√ 

a 2 + d 2 

= 1 

4 π ɛ 0 

q Q d 

(a 2 + d 2 ) 3/2 . 

For maximum x component of the force, 

∂ F x 

= 0 is required. Therefore 

∂d 

keywords: 

∂F x 

∂d = 

q Q a 2 − 2 d 2 

4 π ɛ 0 (a 2 + d 2 ) 5/2 = 0 

a 2 − 2 d 2 = 0 

d = √ a . 2 

Charged Semicircle 

23:10, calculus, numeric, > 1 min, normal. 


Consider the setup shown in the figure below, 

where the arc is a semicircle with radius 

r. The total charge Q is negative, and distributed 

uniformly on the semicircle. The 

charge on a small segment with angle ∆θ is 

labeled ∆q.


y 

y 

∆θ A 

k |∆q| cos θ 

II I 

4. ∆E x = 

− 

x 

r 

θ 

r 

− 

III IV 

k |∆q| sin θ 

5. ∆E 

x 

x = 

r 

O 

6. ∆E x = k |∆q| r 2 

− −− 

−− 

B 

7. ∆E x = k |∆q| (sin θ) r 2 

− 

−−−− 

− 

− −− 

− 

∆q is given by 

1. None of these 

2. ∆q = Q 

3. ∆q = Q ∆θ 

2π 

4. ∆q = 2 Q ∆θ 

π 

5. ∆q = Q ∆θ correct 

π 

6. ∆q = Q 2 π 

8. ∆E x = k |∆q| (cos θ) r 2 

9. ∆E x = k |∆q| (cos θ) r 

10. ∆E x = k |∆q| (sin θ) r 

Explanation: 

Negative charge attracts a positive test 

charge. At O, ∆E points toward ∆q . According 

to the sketch, the vector ∆E x is pointing 

along the negative x axis. The magnitude of 

the ∆E x is given by 

∆E x = ∆E cos θ = k |∆q| 

r 2 cos θ . 

7. ∆q = 2 Q π 

8. ∆q = Q π 

9. ∆q = 2 π Q 

10. ∆q = π Q 

Explanation: 

The angle of a semicircle is π, thus the 

charge on a small segment with angle ∆θ is 

∆q = Q ∆θ 

π 


The magnitude of the x-component of the 

electric field at the center, due to ∆q, is given 

by 

1. ∆E x = k |∆q| 

r 2 

2. ∆E x = 

3. ∆E x = 

k |∆q| sin θ 

r 2 


r 2 

. 

correct 


Determine the magnitude of the electric field 

at O . The total charge is −7.5 µC, the radius 

of the semicircle is 14 cm, and the Coulomb 

constant is 8.98755 × 10 9 N · m 2 /C 2 . 

Correct answer: 2.18941 × 10 6 N/C. 

Explanation: 

Let : Q = −7.5 µC , 

r = 14 cm , and 

k = 8.98755 × 10 9 N · m 2 /C 2 . 

By symmetry of the semicircle, the y- 

component of the electric field at the center 

is 

E y = 0 . 

Combining part 1 and part 2, 

∆E x = 


r 2 

= k |Q| 

π r 2 

cos θ ∆θ


Therefore, the magnitude of the electric field 

at the center is given by 

E = E x = 

= 2 k |Q| 

π r 2 . 

∫ π/2 

−π/2 

k |Q| 

π r 2 

cos θ dθ 

For the above values, the magnitude is given 

by 

E = 2 ( 8.98755 × 10 9 N · m 2 /C 2) |(−7.5 µC)| 

π (14 cm) 2 

= 2.18941 × 10 6 N/C . 

The direction is along negative x axis. 

y 

y 

∆θ A II I 

− 

x 

θ 

r 

− E 

III IV 

x 

O 

keywords: 

− 

−−−− 

− 

− −− 

− 

− −− 

−− 

B 

Flux Through a Pyramid 



A (6 m by 6 m) square base pyramid with 

height of 4 m is placed in a vertical electric 

field of 52 N/C. 

6 m 

52 N/C 

4 m 

Calculate the total electric flux which goes 

out through the pyramid’s four slanted surfaces. 

Correct answer: 1872 N m 2 /C. 

Explanation: 

By Gauss’ law, 

Let : s = 6 m , 

h = 4 m , and 

E = 52 N/C . 

Φ = ⃗ E · ⃗A 

Since there is no charge contained in the pyramid, 

the net flux through the pyramid must 

be 0 N/C. Since the field is vertical, the flux 

through the base of the pyramid is equal and 

opposite to the flux through the four sides. 

Thus we calculate the flux through the base 

of the pyramid, which is 

keywords: 

Φ = E A = E s 2 

= (52 N/C) (6 m) 2 

= 1872 N m 2 /C . 

Flux Through a Submarine 


016 (part 1 of 1) 10 points 

The following charges are located inside a submarine: 

5 µC, −9 µC, 27 µC, and −84 µC. 

Calculate the net electric flux through the 

submarine. 

Correct answer: −6.88954 × 10 6 N · m 2 /C. 

Explanation: 

Let : q 1 = 5 µC = 5 × 10 −6 C , 

q 2 = −9 µC = −9 × 10 −6 C , 

q 3 = 27 µC = 2.7 × 10 −5 C , 

q 4 = −84 µC = −8.4 × 10 −5 C . 

From Gauss’s Law: 

Φ = q 1 + q 2 + q 3 + q 4 

ɛ 0 

= (5 × 10−6 C) + (−9 × 10 −6 C) 

8.854 × 10 −12 C 2 /N · m 2 

and 

+ (2.7 × 10−5 C) + (−8.4 × 10 −5 C) 

8.854 × 10 −12 C 2 /N · m 2 

= −6.88954 × 10 6 N · m 2 /C .


keywords: 

Long Cylindrical Insulator 03 



Consider a long, uniformly charged, cylindrical 

insulator of radius R with charge density 

1 µC/m 3 . (The volume of a cylinder with 

radius r and length l is V = π r 2 l.) 

The value of the Permittivity of free space 

is 8.85419 × 10 −12 C 2 /N · m 2 

R 

1 cm 

What is the magnitude of the electric field 

inside the insulator at a distance 1 cm from 

the axis (1 cm < R) 

Correct answer: 564.705 N/C. 

Explanation: 

Let : r = 1 cm = 0.01 m , 

ρ = 1 µC/m 3 

= 1 × 10 −6 C/m 3 , and 

ɛ 0 = 8.85419 × 10 −12 C 2 /N · m 2 . 

Consider a cylindrical Gaussian surface of 

radius r and length l much less than the 

length of the insulator so that the component 

of the electric field parallel to the axis is 

negligible. 

R 

r 

l 

The flux leaving the ends of the Gaussian 

cylinder is negligible, and the only contribution 

to the flux is from the side of the cylinder. 

Since the field is perpendicular to this surface, 

the flux is 

Φ s = 2 π r l E , 

and the charge enclosed by the surface is 

Using Gauss’ law, 

Q enc = π r 2 l ρ . 

Φ s = Q enc 

ɛ 0 

2 π r l E = π r2 l ρ 

ɛ 0 

. 

Thus 

E = 

ρ r 

2 ɛ 

( 0 

1 × 10 −6 C/m 3) (0.01 m) 

= 

2 (8.85419 × 10 −12 C 2 /N · m 2 ) 

keywords: 

= 564.705 N/C . 

Uniformly Charged Sphere 04 


fixed. 


Given : 

V sphere = 4 π R3 

, and 

3 

A sphere = 4 π R 2 . 

Consider a sphere, which is an insulator, 

where charge is uniformly distributed 

throughout. 

Consider a spherical Gaussian surface with 

radius R , which is concentric to the sphere 

2 

with a radius R. 

Q is the total 

charge inside 

the sphere. 

p 

R 

R 

2


The total amount of flux flowing through 

the Gaussian surface is given by 

6. ‖ E‖ ⃗ = k Q2 

1. Φ = Q 2 R 2 . 

. 

Explanation: 

ɛ 0 Gauss’s Law gives us 

2. Φ = Q . 

4 ɛ 

4 π r 2 E = Q encl 

0 ɛ 0 

3. Φ = Q 

( 

. 

4 R 

2 ɛ 0 

4. Φ = Q 

= Q 3 π 2 

. correct 

ɛ 0 

4 

8 ɛ 0 

5. Φ = 2 Q 

3 π R3 

. 

= Q , 

ɛ 0 8 ɛ 0 

Q 

E = ( ) 2 R 

4 π 8 ɛ 0 

2 

Q 

= 

Φ = 4 π r 2 4 π ɛ 

E 

0 2 R 2 

= Q encl 

= k Q 

. 

2 R 

ɛ 2 . 

0 

Φ = Q encl 

keywords: 

ɛ 

⎡ 0 

( ) 3 

⎤ 

4 π R 

= Q 3 2 ⎢ 

ɛ 0 ⎣ 4 π 

⎥ 

⎦ 

3 R3 

= Q . 

8 ɛ 0 


The magnitude of the electric field ‖ E‖ ⃗ at R 2 

q 2 

is given by 

1. ‖ E‖ ⃗ = k Q 

2 R 2 . correct 

q 1 

2. ‖ E‖ ⃗ = k Q 

R 2 . 

O 

3. ‖ E‖ ⃗ = 2 k Q 

R 2 . 

A 

4. ‖ E‖ ⃗ = 2 k Q2 

B 

R 2 . 

C 

5. ‖ E‖ ⃗ = k Q2 

R 2 . 

6. Φ = 4 Q 

ɛ 0 

. 

Explanation: 

Basic Concept: Gauss’ Law. 

Solution: For spherical symmetric case, 

) 3 

Shell Game 01 v2 


fixed. 


Consider the following spherically symmetric 

situation: We have a charge q 1 on a metallic 

ball at the center, inside of a conducting shell 

of inner radius R 2 and outer radius R 3 . There 

is a total charge of q 2 on the shell. 

R 3 , q 2 

′′ 

R 2 , q 2 

′ 

R 1 , q 1 

a 

b 

c


Find E at A where OA = a. 

1. E A 

= k q 1 

a 2 correct 

2. E A 

= k q 1 

2 a 2 

3. E A 

= k q 1 

b 2 

4. E A 

= k q 1 

c 2 

5. E A 

= 0 

6. E A 

= k q 1 

3 a 2 

q 1 

7. E A 

= k √ 

2 a 2 

8. E A 

= k 2 q 1 

a 2 

9. E A 

= k 3 q 1 

a 2 

10. E A 

= k 4 q 1 

a 2 

Explanation: 

Pick a Gaussian surface (sphere since we 

are in spherical symmetry) center at the point 

charge and of radius a. This surface contains 

only the point charge, so q encl = q 1 . The 

formula for E gives 

8. E B 

= k q 1 − q 2 

b 2 

9. E B 

= k 3 q 1 

b 2 

10. E B 

= k 4 q 1 

b 2 

Explanation: 

For an electrostatic situation, inside of a 

conductor, there is no charge; i.e., q inside = 0. 

Also, ⃗ E inside = 0 and there is no flux inside, 

Φ inside = 0. 

Thus 

E B 

= 0 . 

Notice also that since the electric field at B 

is zero, the total enclosed charge is zero, or 

q 1 + q ′ 2 = 0. Therefore 

q ′ 2 = −q 1 . 

This verifies that the charge on the inner 

surface of a conducting shell is −q 1 , where 

q 1 is the charge is the charge enclosed by the 

shell. 


Find E at C, where OC = c. 

1. E C 

= 0 

E A 

= k q 1 

a 2 . 


Find E at B, where OB = b. 

1. E B 

= 0 correct 

2. E B 

= k q 1 

a 2 

3. E B 

= k q 1 

b 2 

4. E B 

= k q 1 

2 b 2 

5. E B 

= k q 1 

c 2 

6. E B 

= k q 2 

2 b 2 

7. E B 

= k q 1 + q 2 

√ 

2 b 2 

2. E C 

= k q 1 

a 2 

3. E C 

= k q 1 + q 2 

b 2 

4. E C 

= k q 1 − q 2 

2 a 2 

5. E C 

= k q 1 

c 2 

6. E C 

= k q 1 

2 b 2 

7. E C 

= k q 1 + q 2 

c 2 

8. E C 

= k q 1 − q 2 

c 2 

9. E C 

= k 3 q 1 

c 2 

10. E C 

= k 4 q 1 

c 2 

Explanation: 

correct


Here the Gaussian surface is a sphere centered 

at the point charge q 1 and of radius c. 

The enclosed charge in this sphere is all the 

charge, or q 1 + q 2 . The electric field at C is 

keywords: 

E C 

= k q 1 + q 2 

c 2 . 

Solid Conducting Sphere 


fixed. 


A positive charge of 10 −6 coulomb is placed 

on an insulated solid conducting sphere. 

Which of the following is true 

1. When a second conducting sphere is 

connected by a conducting wire to the first 

sphere, charge is transferred until the electric 

potentials of the two spheres are equal. 

correct 

2. The electric field inside the sphere is constant 

in magnitude, but not zero. 

3. The electric field in the region surrounding 

the sphere increases with increasing distance 

from the sphere. 

4. An insulated metal object acquires a net 

positive charge when brought near to, but not 

in contact with, the sphere. 

5. The charge resides uniformly throughout 

the sphere. 

Explanation: 

Every point in the conductor becomes equipotential, 

and the electric field is defined as 

the gradient of the electric potential, so inside 

the conducting sphere, all points are equipotential 

and there is no electric field. 

Outside the conducting sphere, the electric 

field is the same when there are net charges at 

the center of the sphere, so the electric field 

decreases with increasing distance from the 

sphere. 

If there is no net charge on the insulated 

metal object when brought near to, but not 

in contact with the sphere, there is also no 

net charge on it. Only the charge distribution 

changes. 

Since there is repulsion among like charges, 

charges reside uniformly on the surface of the 

sphere. 

keywords: 

Field From a Charged Plate JM 


fixed. 


A uniformly charged conducting plate with 

area A has a total charge Q which is positive. 

The figure below shows a cross-sectional view 

of the plane and the electric field lines due to 

the charge on the plane. The figure is not 

drawn to scale. 

+Q 

+ 

E 

E 

+ 

+ 

+ P 

+ 

+ 

Find the magnitude of the field at point P , 

which is a distance a from the plate. Assume 

that a is very small when compared to the 

dimensions of the plate, such that edge effects 

can be ignored. 

1. ‖ ⃗ E‖ = Q 

ɛ 0 A 

2. ‖ ⃗ E‖ = Q 

2 ɛ 0 A correct 

3. ‖ E‖ ⃗ = Q 

4 ɛ 0 A 

4. ‖ E‖ ⃗ Q 

= 

4 π ɛ 0 a 2 

5. ‖ ⃗ E‖ = Q 

4 π ɛ 0 a 

6. ‖ ⃗ E‖ = 2 ɛ 0 Q A 

7. ‖ ⃗ E‖ = ɛ 0 Q A

¡ 


8. ‖ ⃗ E‖ = 4 π ɛ 0 a 2 Q 

9. ‖ ⃗ E‖ = 4 π ɛ 0 a Q 

Coaxial Cable 01 

24:05, calculus, multiple choice, < 1 min, normal. 


A long coaxial cable consists of an inner cylindrical 

conductor with radius R 1 and an outer 

cylindrical conductor shell with inner radius 

R 2 and outer radius R 3 as shown. The cable 

extends out perpendicular to the plane 

shown. The charge on the inner conductor 

per unit length along the cable is λ and the 

corresponding charge on the outer conductor 

per unit length is −λ (same in magnitudes 

but with opposite signs) and λ > 0. 

−Q 

10. ‖ ⃗ E‖ = ɛ 0 Q a 2 

Explanation: 

Basic Concepts Gauss’ Law, electrostatic 

properties of conductors. 

Solution: Let us consider the Gaussian 

surface shown in the figure. 

+Q 

+ 

+ 

E + E 

+ S 

+ 

+ 

Due to the symmetry of the problem, there 

is an electric flux only through the right and 

left surfaces and these two are equal. If the 

cross section of the surface is S, then Gauss’ 

Law states that 

keywords: 

Φ TOTAL 

= 2 E S 

= 1 ɛ 0 

Q 

A S , so 

E = 

Q 

2 ɛ 0 A . 

Q 

R 2 

R 3 

R 1 

Find the magnitude of the electric field at 

the point a distance r 1 from the axis of the 

inner conductor, where R 1 < r 1 < R 2 . 

1. E = 0 

λ 

2. E = correct 

2 π ɛ 0 r 1 

3. E = 

λ 

√ 

2 π ɛ0 r 1 

4. E = 

λ 

√ 

3 π ɛ0 r 1 

5. E = 

2 λ 

√ 

3 π ɛ0 r 1 

6. E = λ R 1 

4 π ɛ 0 r 1 

2 

7. E = λ R 1 

3 π ɛ 0 r 1 

2 

8. E = λ2 R 1 

4 π ɛ 0 r 1 

2 

9. E = 

λ 

2 π ɛ 0 R 1 

10. None of these. 

Explanation: 

Pick a cylindrical Gaussian surface with the 

radius r 1 and apply the Gauss’s law; we obtain 

E · l · 2 π r 1 = Q ɛ 0 

λ 

E = . 

2 π ɛ 0 r 1 


The electric field vector points 

1. in the negative ˆr direction


2. in the positive ˆr direction correct 

Explanation: 

The field points from positive charge to 

negative change. 

Since the center conductor is negatively 

charged, the electric field vector points in the 

negative ˆr direction. 


Find the magnitude of the electric field at the 

point a distance r 2 from the axis of the inner 

conductor, where R 3 < r 2 . 

1. E = 0 correct 

2. E = 

3. E = 

4. E = 

5. E = 

λ 

2 π ɛ 0 r 2 

λ 

√ 

2 π ɛ0 r 2 

λ 

√ 

3 π ɛ0 r 2 

2 λ 

√ 

3 π ɛ0 r 2 

6. E = λ R 1 

4 π ɛ 0 r 2 

2 

7. E = λ R 1 

3 π ɛ 0 r 2 

2 

8. E = λ2 R 1 

4 π ɛ 0 r 2 

2 

9. E = 

λ 

2 π ɛ 0 R 1 


Explanation: 

Pick a cylindrical Gaussian surface with the 

radius r 2 and apply the Gauss’s law. Because 

there is no net charge inside the Gaussian 

surface, the electric field E = 0 . 


For a 100 m length of coaxial cable with inner 

radius 1 mm and outer radius 1.5 mm. 

Find the capacitance C of the cable. 

Correct answer: 13.7207 nF. 

Explanation: 

Let : l = 100 m , 

R 1 = 1 mm , 

R 2 = 1.5 mm . 

and 

We calculate the potential across the capacitor 

by integrating −E · ds. We may choose 

a path of integration along a radius; i.e., 

−E · ds = −Edr. 

V = − 1 ∫ 

q 

R1 

2 π ɛ 0 l R 2 

∣ 

= − 1 q 

2 π ɛ 0 l 

ln r 

∣ 

= q 

2 π ɛ 0 l ln R 2 

R 1 

. 

dr 

r 

R 1 

R 2 

Since C = q , we obtain the capacitance 

V 

C = 

keywords: 

2 π ɛ 0 l 

( ) 

R2 

ln 

R 1 

= 2 π (8.85419 × 10−12 c 2 /N · m 2 ) 

( ) 1.5 mm 

ln 

1 mm 

× (100 m) 

= 13.7207 nF . 

Charge in a Closed Surface 



A closed surface with dimensions a = b = 

0.4 m and c = 0.36 m is located as in the figure. 

The electric field throughout the region 

is nonuniform and given by ⃗ E = (α + β x 2 ) î 

where x is in meters, α = 3 N/C, and 

β = 2 N/(C m 2 ).


y 

a 

E 

× (0.133632 N m 2 /C) 

= 1.1832 × 10 −12 C . 

z 

c 

What is the magnitude of the net charge 

enclosed by the surface 


Explanation: 

Let : a = b = 0.4 m , 

c = 0.36 m , 

α = 3 N/C , and 

β = 2 N/(C m 2 ) . 

The electric field throughout the region is 

directed along the x-axis and the direction of 

d A ⃗ is perpendicular to its surface. Therefore, 

⃗E is parallel to d A ⃗ over the four faces of 

the surface which are perpendicular to the 

yz plane, and E ⃗ is perpendicular to d A ⃗ over 

the two faces which are parallel to the yz 

plane. That is, only the left and right sides 

of the right rectangular parallel piped which 

encloses the charge will contribute to the flux. 

The net electric flux through the cube is 

∫ 

∫ 

∆Φ = E x d A ⊥ − E x d A ⊥ 

right side 

b 

a 

left side 

= a b [ α + β(a + c) 2 − α − β a 2] 

= a b β (2 a c + c 2 ) 

= a b c β (2 a + c) 

= (0.4 m) (0.4 m) (0.36 m) 

× [2 N/(C m 2 )] [2 (0.4 m) + 0.36 m] 

= 0.133632 N m 2 /C , 

so the enclosed charge is 

q = ɛ 0 ∆Φ 

= [8.85419 × 10 −12 C 2 /(N m 2 )] 

x 


What is the sign of the charge enclosed in the 

surface 

1. positive correct 

2. negative 

3. Cannot be determined 

Explanation: 

Since there is more flux coming out of the 

surface than going into the surface, the sign 

of the enclosed charge must be positive. 

Flux Through a Loop 01 



A 40 cm diameter loop is rotated in a uniform 

electric field until the position of maximum 

electric flux is found. The flux in this position 

is measured to be 520000 N · m 2 /C. 

What is the electric field strength 

Correct answer: 4.13803 × 10 6 N/C. 

Explanation: 

Let : r = 20 cm = 0.2 m and 

By Gauss’ law, 

∮ 

Φ = 

Φ = 520000 N · m 2 /C . 

⃗E · d ⃗ A 

The position of maximum electric flux will be 

that position in which the plane of the loop is 

perpendicular to the electric field; i.e., when 

⃗E · d ⃗ A = E dA. Since the field is constant, 

E = 

Φ = E A = Eπ r 2 

Φ 

π r 2 

= 520000 N · m2 /C 

π (0.2 m) 2 

= 4.13803 × 10 6 N/C .


keywords: 

Three Point Charges 17 


normal. 


Consider three point charges at the vertices of 

an equilateral triangle. Let the potential be 

zero at infinity. 

The value of the Coulomb constant is 

8.98755 × 10 9 N · m 2 /C 2 . 

1.5 µC 

ĵ 

60 ◦ 

0.2 m 

3 µC P −5 µC 

What is the electrostatic potential at the 

point P at the center of the base of the equilateral 

triangle given in the diagram 

Correct answer: −101917 V. 

Explanation: 

Let : q 1 = 1.5 µC = 1.5 × 10 −6 C , 

q 2 = 3 µC = 3 × 10 −6 C , 

q 3 = −5 µC = −5 × 10 −6 C , 

a = 0.2 m , and 

k e = 8.98755 × 10 9 N · m 2 /C 2 . 

The potential at P is given by 

∑ q i 

V = k e . 

r i 

From the sketch below, the height h is given 

by √ 

( a 

) √ 

2 3 

h = a 2 − = 

2 2 a . 

Notice that q 1 > 0,q 2 > 0, and q 3 < 0. 

( 

q1 

V P = k e 

h + q 2 

a/2 + q ) 

3 

a/2 

( ) 

q1 

√ + q 2 + q 3 

3 

= 2 k e 

a 

= 2 ( 8.98755 × 10 9 N · m 2 /C 2) 

0.2 m 

i 

î 

× 

( 1.5 × 10 −6 C 

√ 

3 

= −101917 V . 

+ 3 × 10 −6 C 

−5 × 10 −6 C ) 


What is the vertical component of the electric 

force on the 1.5 µC charge due to the 3 µC 

charge 

1. F = k e (1.5 µC) (3 µC) 

(0.2 m) 2 cot 30 ◦ 

2. F = k e (1.5 µC) (3 µC) 

(0.2 m) 2 cot 60 ◦ 

3. F = k e (1.5 µC) (3 µC) 

(0.2 m) 2 cos 30 ◦ correct 

4. F = k e (1.5 µC) (3 µC) 

(0.2 m) 2 cos 60 ◦ 

5. F = k e (1.5 µC) (3 µC) 

(0.2 m) 2 tan 30 ◦ 

6. F = k e (1.5 µC) (3 µC) 

(0.2 m) 2 tan 60 ◦ 

7. F = k e (1.5 µC) (3 µC) 

(0.2 m) 2 

8. F = k e (1.5 µC) (3 µC) 

(0.2 m) 2 sin 45 ◦ 

9. F = k e (1.5 µC) (3 µC) 

(0.2 m) 2 tan 45 ◦ 

10. F = k e (1.5 µC) (3 µC) 

(0.2 m) 2 cot 45 ◦ 

Explanation: 

F v = F cos α 

= k e q 1 q 2 

r 2 

cos α 

= k e (1.5 µC) (3 µC) 

(0.2 m) 2 cos 30 ◦ 


Find the total electrostatic energy of the system, 

again with the zero reference at infinity. 

Correct answer: −0.80888 J.

Version One – Homework 1 – Juyang Huang – 24018 – Jan 16, 2008 16 

Explanation: 

keywords: 

The total electrostatic energy of the system 

is the sum of the electrostatic energies 

between each pair of charges: 

U = U 12 + U 23 + U 31 

The electrostatic energy between the charges 

q i and q j is given by 

U ij = 

q i q j 

4 π ɛ 0 r 

−Q 

where r is the distance between the charges, 

so, since k e = 1 , 

4 π ɛ 0 

] 

U = k e a 

[q 1 q 2 + q 2 q 3 + q 3 q 1 

= ( 8.98755 × 10 9 N · m 2 /C 2) 

[ 

× (0.2 m) (1.5 × 10 −6 C) (3 × 10 −6 C) 

+ (3 × 10 −6 C) (−5 × 10 −6 C) 

] 

+ (−5 × 10 −6 C) (1.5 × 10 −6 C) 

= −0.80888 J . 

1. No charge flows. 

keywords: 

Moving a Charge 



It takes 120 J of work to move 1 C of charge 

from a positive plate to a negative plate. 

What voltage difference exists between the 

plates 

Correct answer: 120 V. 

Explanation: 

Let : W = 120 J and 

q = 1 C . 

The voltage difference is 

V = W q = 120 J 

1 C = 120 V . 

AP B 1993 MC 70 


fixed. 


Two negatively charged spheres with different 

radii are shown in the figure below. 

−Q 

The two conductors are now conneted by a 

wire. 

Which of the following occurs when the two 

spheres are connected with a conducting wire 

2. Negative charge flows from the larger 

sphere to the smaller sphere until the electric 

field at the surface of each sphere is the 

same. 

3. Negative charge flows from the larger 

sphere to the smaller sphere until the electric 

potential of each sphere is the same. 

4. Negative charge flows from the smaller 

sphere to the larger sphere until the electric 

field at the surface of each sphere is the 

same. 

5. Negative charge flows from the smaller 

sphere to the larger sphere until the electric 

potential of each sphere is the same. correct 

Explanation: 

When the wire is connected, charge will flow 

until each surface is at the same potential. 

When disconnected the potential of each


sphere is given by 

V = k e q 

r . 

The smaller sphere is at a more negative potential 

than the larger sphere, so negative 

charge will flow from the smaller sphere to 

the large one until they are at the same potential. 

keywords: 

Equipotential Surfaces 02 


fixed. 


Consider the figure 

+Q A −Q 

+ − 

y 

+ − 

+ − 

x 

+ − 

C D 

+ − 

+ − 

#1 B #2 

Of the following elements, identify all that 

correspond to an equipotential line or surface. 

1. line AB only correct 

2. line CD only 

3. both AB and CD 

4. neither AB nor CD 

Explanation: 

Consider the electric field 

+Q A −Q 

+ − 

y 

+ − 

+ − 

+ 

C D 

− 

+ − 

+ − 

#1 B #2 

An equipotential line or surface (AB) is 

normal to the electric field lines. 

x 


Consider the figure 

C 

− A 

−q 

B 

+ 

+q 

D 

Of the following elements, identify all that 

correspond to an equipotential line or surface. 

1. line AB only 

2. line CD only correct 

3. both AB and CD 

4. neither AB nor CD 

Explanation: 

Consider the electric field: 

A 

− 

An equipotential line or surface (CD) is 

normal to the electric field lines. 

keywords: 

Starting a Car 03 



The gap between electrodes in a spark plug 

is 0.06 cm. To produce an electric spark 

in a gasoline-air mixture, an electric field of 

3 × 10 6 V/m must be achieved. 

On starting a car, what is the magnitude of 

the minimum voltage difference that must be 

C 

D 

B 

+


supplied by the ignition circuit 


Explanation: 

Let : E = 3 × 10 6 V/m and 

d = 0.06 cm = 0.0006 m . 

Assuming the electric field between the two 

electrodes is constant, then the potential difference 

between the electrodes is 

keywords: 

V = E d 

= ( 3 × 10 6 V/m ) (0.0006 m) 

= 1800 V . 

Accelerating an Electron 



Through what potential difference would an 

electron need to be accelerated for it to 

achieve a speed of 4 % of the speed of light 

(2.99792 × 10 8 m/s), starting from rest 

Correct answer: 408.799 V. 

Explanation: 

Let : s = 4% = 0.04 , 

c = 2.99792 × 10 8 m/s , 

m e = 9.10939 × 10 −31 kg , 

q e = 1.60218 × 10 −19 C . 

The speed of the electron is 

v = 0.04 c 

= 0.04 ( 2.99792 × 10 8 m/s ) 

= 1.19917 × 10 7 m/s , 

By conservation of energy 

1 

2 m e v 2 = −(−q e ) ∆V 

and 

v 2 

∆V = m e 

2 q e 

= ( 9.10939 × 10 −31 kg ) 

( 

1.19917 × 10 7 m/s ) 2 

× 

2 (1.60218 × 10 −19 C) 

= 408.799 V . 

keywords: 

Point Charge 



At distance r from a point charge q, the electric 

potential is 600 V and the magnitude of 

the electric field is 200 N/C. 

Determine the value of q. 


Explanation: 

Let : k e = 8.98755 × 10 9 N · m 2 /C 2 , 

V = 600 V , and 

e = 200 N/C . 

E = k e q 

r 2 and V = k e q 

r , so that V E = r. 

The potential is 

V = k e q 

r 

= k e q 

V 

E 

= k e q E 

V 

q = V 2 

k e E 

(600 V) 2 

= 

(8.98755 × 10 9 N · m 2 /C 2 ) (200 N/C) 

= 2.00277 × 10 −7 C . 

keywords: 

Conducting Spheres 02 




Consider two “solid” conducting spheres with 

radii r 1 = 4 R and r 2 = 3 R ; i.e., 

r 2 

r 1 

= 3 R 

4 R = 3 4 .


The two spheres are separated by a large 

distance so that the field and the potential at 

the surface of sphere #1 only depends on the 

charge on #1 and the corresponding quantities 

on #2 only depend on the charge on 

#2. 

Place an equal amount of charge on both 

spheres, q 1 = q 2 = Q . 

#1 

r 1 

q 1 

r 2 

q 2 #2 

After the electrostatic equilibrium on each 

sphere has been established, what is the ratio 

of the potentials V 2 

at the “centers” of the 

V 1 

two solid conducting spheres 

1. V 2 

V 1 

= 4 3 correct 

2. V 2 

V 1 

= 3 4 

3. V 2 

V 1 

= 3 2 

4. V 2 

V 1 

= 3 8 

5. V 2 

= 16 V 1 9 

6. V 2 

= 9 

V 1 16 

7. V 2 

= 9 V 1 8 

8. V 2 

= 9 

V 1 32 

9. V 2 

V 1 

= 1 

Explanation: 

For a solid conducting sphere, the charge is 

uniformly distributed at the surface. From 

Gauss’ Law, the electric field outside the 

sphere is given by E(r) = k Q r 2 , where Q 

is the total charge on the sphere and r is the 

distance from the center of the sphere. By integration 

with respect to r, the potential can 

be expressed as V (r) = k Q , so the potential 

r 

at the surface of the sphere is 

V (r) = k Q r , (1) 

where R is radius of the sphere and r ≤ R . 

For the electrostatic case, the potential is 

constant throughout a conducting body, so 

the potential at the center is the same as 

anywhere on the conductor. 

Thus at two centers 

k q 2 

V 2 r 

= 2 

V 1 k q 1 

r 1 

= r 1 

r 2 

= 4 R 

3 R 

= 4 3 . 


What is the ratio of the electric fields E 2 

E 1 

at 

the “surfaces” of the two spheres 

1. E 2 

E 1 

= 16 9 correct 

2. E 2 

E 1 

= 9 

16 

3. E 2 

= 9 E 1 8 

4. E 2 

= 9 

E 1 32 

5. E 2 

E 1 

= 4 3 

6. E 2 

E 1 

= 3 4 

7. E 2 

E 1 

= 3 2 

8. E 2 

E 1 

= 3 8 

9. E 2 

E 1 

= 1 

Explanation:


For a conducting sphere, the charge is uniformly 

distributed at the surface. Based on 

Gauss’ law, the electric field on the surface of 

a conducting sphere of radius R with charge 

Q is 

Q 

E(r) = k e , where r ≥ R . (2) 

r2 Thus on the surface r = R of the two 

spheres, 

E 2 

E 1 

= 

k q 2 

r 2 2 

k q 1 

( 

r1 

r 2 1 

) 2 

= 

r 2 

( 4 R 

= 

3 R 

( 2 4 

= 

3) 

= 16 9 . 

) 2 


Now “connect” the two spheres with a wire. 

r 1 

r 2 

5. E 2 

= 16 E 1 9 

6. E 2 

= 9 

E 1 16 

7. E 2 

= 9 E 1 8 

8. E 2 

= 9 

E 1 32 

9. E 2 

E 1 

= 1 

Explanation: 

When the spheres are connected by a wire, 

charge will flow from one to the other until 

the potential on both spheres is the same. 

As noted, V 2 

V 1 

= 1, defines equilibrium. 

The spheres are connected by a wire and no 

current is flowing (at equilibrium), therefore 

the ends of the wire are at the same potential 

V 2 = V 1 . (3) 

For a conducting sphere, the charge is uniformly 

distributed at the surface. Based on 

Gauss’ law, on the surface of a conducting 

sphere of radius R with charge Q is 

Q 

E(r) = k e , where r ≥ R , and 

r2 V (r) = k Q r , where r ≤ R . 

#1 

q 1 

q 2 #2 

Thus on the surface r = R of the two 

spheres, 

There will be a flow of charge through the 

wire until equilibrium is established. 

What is the ratio of the electric fields E 2 

E 1 

at 

the “surfaces” of the two spheres 

1. E 2 

E 1 

= 4 3 correct 

2. E 2 

E 1 

= 3 4 

3. E 2 

E 1 

= 3 2 

4. E 2 

E 1 

= 3 8 

E 2 

E 1 

= 

= 

= 

= r 1 

r 2 

k q 2 

r2 

2 

k q 1 

r1 

2 

k q 2 1 

r 2 r 2 

k q 1 1 

r 1 r 1 

V 2 

1 

r 2 

V 1 

1 

r 1 

, since V 1 = V 2 

(4)


= 4 R 

3 R 

= 4 3 . 

7 

4 q 1 = 2 Q 

q 1 = 8 7 Q . 


Now, what is the charge q 1 on sphere #1 

1. q 1 = 8 7 Q correct 

2. q 1 = 6 7 Q 

3. q 1 = 7 8 Q 

4. q 1 = 7 6 Q 

5. q 1 = 4 7 Q 

6. q 1 = 3 7 Q 

7. q 1 = 7 4 Q 

8. q 1 = 7 3 Q 

9. q 1 = Q 

Explanation: 

When the spheres are connected by a wire, 

charge will flow from one to the other until 

the potential on both spheres is the same. 

In this case, this implies that 

k e 

q 1 

r 1 

= k e 

q 2 

r 2 

, 

q 2 = r 2 

r 1 

q 1 

= 3 R 

4 R q 1 

or 

= 3 4 q 1 . (5) 

The total charge of the system remains constant; 

i.e., from the initial condition q 1 = 

q 2 = Q, the total change on both spheres is 

q 1 + q 2 = 2 Q. Using q 2 from Eq. 5, we have 

q 1 + q 2 = 2 Q 

q 1 + 3 4 q 1 = 2 Q 

And the charge on sphere # 2 is q 2 = 6 7 Q , 

since q 1 + q 2 = 8 7 Q + 6 7 Q = 2 Q . 

Check Eq. 4: On the surfaces of the two 

spheres, 

( ) ( ) 2 

E 2 q2 r1 

= 

E 1 q 1 r 2 

⎛ ⎞ 

6 

⎜ 

= ⎝ 

7 Q ( ) 2 

⎟ 4 R 

8 

⎠ 

7 Q 3 R 

( ) ( 2 3 4 

= 

4 3) 

= 4 3 . 

Third of eighteen versions. 

keywords: 

Change in Potential e2 

25:04, calculus, multiple choice, < 1 min, normal. 


A uniform electric field of magnitude 250 V/m 

is directed in the positive x-direction. Suppose 

a 12 µC charge moves from the origin to 

point A at the coordinates, (20 cm, 50 cm). 

y 

250 V/m 

O 

(20 cm, 50 cm) 

A 

What is the absolute value of the change in 

potential from the origin to point A 


Explanation: 

Let : x = 20 cm , 

y = 50 cm , 

‖ ⃗ E‖ = 250 V/m . 

and 

x


The potential difference from O to A is 

defined as 

∆V = V A − V O = − 

∫ A 

O 

⃗E · d⃗s . 

We know that E ⃗ = (250 V/m) î . We need 

to choose a path to integrate along. Because 

the electric force is conservative, it doesn’t 

matter which path we take; they all give the 

same answer. There are two choices of path 

for which the math is simple (see the figure 

below.) 

y 

E 

O 

Path I: 

II 

I 

(x, y) 

B 

A 

I 

x 

which is the same as the result for the other 

path. 

keywords: 

Potential Diagrams 02 




Consider a sphere with radius R and charge 

Q 

Q 

V A − V O = (V A − V B ) + (V B − V O ), 

From O to B, ⃗ E and d⃗s are both along the 

x-axis, so ⃗ E · d⃗s = E dx. From B to A, ⃗ E and 

d⃗s are perpendicular, so ⃗ E · d⃗s = 0. 

and the following graphs: 

∝ 1 r 

∫ B 

V A − V O = − 

= − 

= −E 

O 

∫ x 

0∫ x 

O 

⃗E · d⃗s − 

E dx − 

∫ A 

B 

∫ y 

0 

0 dy 

dx = −E ∆x 

= −(250 V/m) (0.2 m) 

= −50 V . 

⃗E · d⃗s 

Q. 

G. 

0 

R 

∝ 1 r 

r 

The absolute value is 

|∆V | = 50 V . 

0 

R 

r 

Path II: In this case, E ⃗ · d⃗s = E cos θ ds . 

where cos θ = x ⇒ x = l cos θ . 

l 

∝ 1 r 2 

∫ l 

V A − V O = −E cos θ ds 

O 

= −E l cos θ 

= −E x . 

X . 

0 

R 

r


∝ 1 r 

1. Y correct 

2. S 

P. 

3. L 

0 

R 

r 

4. X 

Z. 

M. 

Y. 

S. 

L. 

0 

0 

0 

0 

0 

R 

∝ 1 r 

R 

R 

R 

R 

∝ 1 r 

∝ 1 r 2 

∝ 1 r 2 

∝ 1 r 2 

∝ 1 r 2 

Which diagram describes the electric field 

vs radial distance [E(r) function] for a conducting 

sphere 

r 

r 

r 

r 

r 

5. Z 

6. G 

7. Q 

8. P 

9. M 

Explanation: 

The electric field for R < r with 

the sphere conducting and/or uniformly 

non-conducting: Because the charge distribution 

is spherically symmetric, we select a 

spherical gaussian surface of radius R < r, 

concentric with the conducting sphere. The 

electric field due to the conducting sphere is 

directed radially outward by symmetry and is 

therefore normal to the surface at every point. 

Thus, E ⃗ is parallel to dA ⃗ at each point. Therefore 

E ⃗ · dA ⃗ = E dA and Gauss’s law, where E 

is constant everywhere on the surface, gives 

∮ 

Φ E = ⃗E · dA 

⃗ 

∮ 

= E dA 

∮ 

= E dA 

= E ( 4 π r 2) = q in 

, 

ɛ 0 

where we have used the fact that the surface 

area of a sphere A = 4 π r 2 . Now, we solve for 

the electric field 

E = 

q in 

4 π ɛ 0 r 2 

= 

Q 

, where R < r . (1) 

4 π ɛ 0 r2 This is the familiar electric field due to a point 

charge that was used to develop Coulomb’s 

law.


as in Part 1. 

The electric field for r < R with the 

E = 

Q 

4 π ɛ 0 r 2 , where R < r , (1) = k Q r , 

R3 where R < r . (3) 

sphere conducting: In the region inside The electric field for r < R with the 

the conducting sphere, we select a spherical sphere uniformly non-conducting: In 

gaussian surface r < R, concentric with the this case we select a spherical gaussian surface 

conducting sphere. To apply Gauss’s law 

at a radius r where r < R, concentric 

in this situation, we realize that there is no with the uniformly charged non-conducting 

charge within the gaussian surface (q in = 0), sphere. Let us denote the volume of this 

which implies that 

sphere by V ′ . To apply Gauss’s law in this 

E = 0 , where r < R . (2) 

situation, it is important to recognize that the 

charge q in within the gaussian surface of the 

E ∝ 1 volume V ′ is less than Q. Using the volume 

r 2 

charge density ρ ≡ Q 

E 

V , we calculate q in : 

Y. 

q in = ρ V 

( ′ 

) 4 

0 R 

r 

= ρ 

3 π r3 . 


By symmetry, the magnitude of the electric 

Which diagram describes the electric field vs 

field is constant everywhere on the spherical 

radial distance [E(r) function] for a uniformly 

gaussian surface and is normal to the surface 

charged non-conducting sphere 

at each point. Therefore, Gauss’s law in the 

1. S correct 

2. L 

region r < R gives 

∮ 

∮ 

E dA = E dA 

3. X 

4. Z 

= E ( 4 π r 2) = q in 

. 

ɛ 0 

Solving for E gives 

5. G 

E = 

q in 

4 π ɛ 0 r 2 

6. Q 

ρ 4 

7. P 

= 3 π r3 

4 π ɛ 0 r 2 

8. Y 

= ρ r . 

3 ɛ 0 

9. M 

Because ρ = 

Q (by definition) and since 

Explanation: 

4 

The electric field for R < r with 

3 π R3 

the sphere conducting and/or uniformly k = 1 , this expression for E can be written 

as 

non-conducting: In the region outside the 4 π ɛ 0 

uniformly charged non-conducting sphere, we 

have the same conditions as for the conducting 

sphere when applying Gauss’s law, so 

E = 

Q r 

4 π ɛ 0 R 3


Note: This result for E differs from the one we 

obtained in the Part 3. It shows that E → 0 

as r → 0. Therefore, the result eliminates the 

problem that would exist at r = 0 if E varied 

as 1 inside the sphere as it does outside the 

r2 sphere. That is, if E ∝ 1 for r < R, the field 

r2 would be infinite at r = 0, which is physically 

impossible. Note: Also the expressions for 

Parts 1 and 2 match when r = R. 

S. 

E 

0 

R 

E ∝ 1 r 2 


Which diagram describes the electric potential 

vs radial distance [V (r) function] for a 

conducting sphere 

1. Z correct 

2. G 

3. Q 

4. P 

5. Y 

6. S 

7. L 

8. X 

9. M 

Explanation: 

The electric potential for R < r with 


non-conducting: In the previous parts we 

found that the magnitude of the electric field 

outside a charged sphere of radius R is 

E = k Q r 2 , where R < r , 

where the field is directed radially outward 

r 

when Q is positive. 

In this case, to obtain the electric potential 

at an exterior point, we use the definition for 

electric potential: 

V = − 

∫ r 

= −k Q 

= k Q r 

E dr 

∞ 

∫ r 

∞ 

dr 

r 2 

, where R < r . (4) 

Note: This result is identical to the expression 

for the electric potential due to a point charge. 

The electric potential for r < R with 

the sphere conducting: In the region inside 

the conducting sphere, the electric field E = 

0 . Therefore the electric potential everywhere 

inside the conducting sphere is constant; that 

is 

Z. 

V = V (R) = constant , where R < r . 

V 

0 

R 

V ∝ 1 r 

r 

(5) 


Which diagram describes the electric potential 

vs radial distance [V (r) function] for a 

uniformly charged non-conducting sphere 

1. G correct 

2. Q 

3. P 

4. Y 

5. S 

6. L 

7. X



V r = V R + ∆V 

= k Q ∫ r 

R − Explanation: 

E dr 

We know that the potential due to a collection 

of N point charges is given by 

R 

= k Q R − k Q ∫ r 

R 3 r dr , from Eq. 6 

R 

= k 2 Q 

2 R + k Q ( 

R 2 

2 R 3 − r 2) 

V = 1 ∑ 

N q i 

4 π ɛ 0 r 

i=1 i 

= k 3 Q 

2 R − k Q 

2 R 3 r2 = 1 ( q 

4 π ɛ 0 a + −q ) 

= 0 

a 

8. Z 

= k Q ( 

3 − r 

2 ) , 

2 R 

where r < R . 

9. M 

Explanation: 

The electric potential for R < r with 


V 

V ∝ 1 r 

non-conducting: In the region outside the 

uniformly charged non-conducting sphere, we 

G. 

have the same conditions as for the conducting 

sphere when applying the definition for 

the electric potential; therefore, 

0 R 

r 

∫ r 

V = − E dr 

keywords: 

∞ 

∫ r 

dr 

Finding Zero Potential 

= −k Q 

∞ r 2 


= k Q fixed. 

, where R < r . (4) 


r 

All of the charges shown are of equal magnitude. 

The electric potential for r < R with 

the sphere uniformly non-conducting: 

Because the potential must be continuous at 

r = R , we can use this expression to obtain 

the potential at the surface of the sphere; i.e., 

−q +q 

the potential at a point on the conducting 

sphere is V = k Q a a 

r 

From Part 2 we found that the electric field 

inside an uniformly charged non-conducting 

(a) 

sphere is 

What is the electric potential E at the origin 

Assume zero potential at infinity. 

E = k Q r , where r < R . (6) 

R3 We can use this result in the definition for 

the electric potential to evaluate the potential 

difference ∆V = V r − V R (where V R = k Q R as 

shown in Eq. 4) at some interior point of the 

1. zero correct 

2. positive 

3. negative 

sphere, so



a 

a 

−q +q 

+q 

(b) 

2a 


1. zero 

2. positive correct 

3. negative 


Explanation: 

V = 1 ( −q 

4 π ɛ 0 a + q a + q ) 

> 0 

2 a 


3. negative 


Explanation: 

V = 1 ( −q 

4 π ɛ 0 2 a + −q 

2 a + q ) 

= 0 

a 


−q 

+q 

a 

+q 

(d) 

2a 

2a 



−q 

a 

+q 

−q 

2a 

2a 

2. positive 

3. negative 


Explanation: 

V = 1 ( −q 

4 π ɛ 0 a + q 

2 a + q ) 

= 0 . 

2 a 

(c) 



2. positive 

keywords: 

Charge on a Capacitor 


055 (part 1 of 1) 10 points


A 15 pF capacitor is connected across a 75 V 

source. 

What charge is stored on it 


Explanation: 

Let : C = 15 pF = 1.5 × 10 −11 F and 

V = 75 V . 

The capacitance is 

keywords: 

C = q V 

q = C V 

= (1.5 × 10 −11 F) (75 V) 

= 1.125 × 10 −9 C 

Capacitance Comparison 02 


fixed. 


A parallel plate capacitor is connected to a 

battery. 


Explanation: 

The capacitance of a parallel plate capacitor 

is 

C = ɛ 0 

A 

d . 

Hence doubling d halves the capacitance, 

and Q = C V is also halved 

keywords: 

( 

C ′ = ɛ 0 

A 

2 d = 1 2 ɛ 0 

A 

d = 1 ) 

2 C . 

Plate Separation 



A parallel-plate capacitor has a plate area of 

12 cm 2 and a capacitance of 7 pF. 

The permittivity of a vacuum is 8.85419 × 

10 −12 C 2 /N · m 2 . 

What is the plate separation 

Correct answer: 0.00151786 m. 

Explanation: 

+Q −Q 

Let : A = 12 cm 2 = 0.0012 m 2 , 

C = 7 pF = 7 × 10 −12 F , and 

ɛ 0 = 8.85419 × 10 −12 C 2 /N · m 2 . 

¢ £ 

d 

¤ ¥ 

2 d 

If we double the plate separation, 

1. the capacitance is doubled. 

2. the electric field is doubled. 

3. the potential difference is halved. 

4. the charge on each plate is halved. correct 

C = ɛ 0 A 

d 

d = ɛ 0 A 

( C 

8.85419 × 10 −12 C 2 /N · m 2) 

= 

keywords: 

7 × 10 −12 F 

× ( 0.0012 m 2) 

= 0.00151786 m . 

AP B 1993 MC 15 16



C 

fixed. 

123 = C 12 C 3 

C 3 + C 12 

1 

= 1 + 1 = C 3 + C 12 

C 123 C 12 C 3 C 12 C 3 


(6 µF) (3 µF) 

Consider the circuit 

= 

6 µF + 3 µF 

2 µF 

= 2 µF . 

3 µF 

c 

a 

b 

C 123 and C 4 are parallel, so 

5 µF 

C = C 4 + C 123 

4 µF 

= 7 µF . 

100 V 

What is the equivalent capacitance for this 

network 

1. C equivalent = 10 7 µF 



4. C equivalent = 7 µF correct 

5. C equivalent = 14 µF 

Explanation: 

C 1 

c 

a 

C 4 

C 2 

E B 

1. Q 1 = 360 µC 

2. Q 1 = 500 µC correct 

3. Q 1 = 710 µC 

4. Q 1 = 1, 100 µC 

5. Q 1 = 1, 800 µC 

Explanation: 

Let : C 4 = 5 µF 

E B = 100 

C 3 

and 

b 

V . 

Q 4 = C 4 V 

= (5 µF) (100 V) 

Let : C 1 = 2 µF , 

C 2 = 4 µF , 

C 3 = 3 µF , 

= 500 µC . 

C 4 = 5 µF , and 

E B = 100 V . 

keywords: 

The equivalent capacitance of capacitors C 1 

and C 2 (parallel) is C 12 = C 1 + C 2 = 6 µF . 

C 12 and C 3 are in series, so 


What is the charge stored in the 5-µF lowerright 

capacitor 

The charge stored in a capacitor is given by 

Q = C V , so, 

Capacitor Circuit 02 



A capacitor network is shown below.


100 V 

9 µF 

y 

15 µF 

15 µF 

15 µF 

15 µF 

z 

15 µF 15 µF 

What is the equivalent capacitance between 

points y and z of the entire capacitor network 

Correct answer: 14.4545 µF. 

Explanation: 

Let : C a = C = 15 µF , 

C b = C = 15 µF , 

C c = C = 15 µF , 

C d = C = 15 µF , 

C e = C = 15 µF , 

C f = C = 15 µF , 

ER 

C x = 9 µF = 9 × 10 −6 F 

E B = V = 100 V . 

Cx 

C a 

Ce 

C f 

For capacitors in series, 

y 

z 

C b 

Cc 

C d 

and 

The capacitors C b , C c , and C d are in series, 

so 

1 

C bcd 

= 1 C + 1 C + 1 C = 3 C 

C bcd = 1 3 C . 

This reduces the circuit to 

ER 

Cx 

y 

z 

C a 

Ce 

C f 

Cbcd 

The capacitors C e and C bcd are parallel, so 

C bcde = C + C bcd = C + 1 3 C = 4 3 C . 


ER 

Cx 

y 

z 

C a 

C f 

Cbcde 

The capacitors C a , C bcde and C f are in series, 

so 

1 

= 1 C abcdef C + 3 

4 C + 1 C = 11 

4 C 

C abcdef = 4 

11 C . 


y 

1 

C series 

= ∑ 1 

C i 

V series = ∑ V i , 

and the individual charges are the same. 

For parallel capacitors, 

C parallel = ∑ C i 

Q parallel = ∑ Q i , 

and the individual voltages are the same. 

ER 

Cx 

Cabcdef 

z 

These capacitors are parallel, so 

C yz = C x + C abcdef 

= C x + 4 

11 C 

= 9 µF + 4 (15 µF) 

11 

= 14.4545 µF .



Case Two 

What is the charge on the 9 µF capacitor 

C 1 C ′ 2 

centered on the left directly between points y 

and z 

Correct answer: 0.0009 C. 

κ 

Explanation: 

C ≡ q V 

V 

q = C x V 

= (9 × 10 −6 F) (100 V) 

= 0.0009 C . 


2. C′ 12 

= 2 

C 12 1 + κ . 

3. C′ 12 

= κ . 

C 12 

4. C′ 12 

= 1 + κ 

C 12 2 κ . 

keywords: 

5. C′ 12 

= 1 + κ . 

C 12 2 

Capacitors in Series 

6. C′ 12 

= 

2 κ 

26:05, trigonometry, multiple choice, > 1 min, C 12 1 + κ . correct 

fixed. 

Explanation: 


Consider the two cases shown below. In Case 

One two identical capacitors are connected to 

a battery with emf V . In Case Two, a dielectric 

slab with dielectric constant κ fills the 

gap of capacitor C 2 . Let C be the resultant 

capacitance for Case One and C ′ the resultant 

capacitance for Case Two. 

Case One 

C 12 = C 1 C 2 

. 

C 

C 1 C 1 + C 2 

2 

The ratio C′ 12 

C 12 

of the resultant capacitances is 

Let : C 1 = C 2 = C and 

C ′ 2 = κ C 2 = κ C , 

where κ is dielectric constant. 

V = constant. C 1 and C 2 are in series, so 

For Case One, 

For Case Two, 

1 

C 12 

= 1 C 1 

+ 1 C 2 

= C 2 + C 1 

C 1 C 2 

C 12 = C 1 C 2 

C 1 + C 2 

= C2 

2 C = C 2 . 

V 

C ′ 12 = C 1 C ′ 2 

C 1 + C ′ 2 

= κ C2 

(1 + κ) C = κ C 

1 + κ .

Therefore 


C 12 

′ = 

2 κ 

C 12 1 + κ . 

4. U ′ 


of potential differences across 

V 2 

6. U ′ 

2 κ 

1 + κ . 

V 2 = Q 2 

= V C 12 

= V C 2 C 2 2 . 

V 2 ′ = Q′ 2 

C 2 

′ = V C′ 12 

C 2 

′ 

= V 1+κ 

κ C 

κ C = V 

1 + κ . 

V 2 

′ 

= 2 

V 2 1 + κ . 


of total energy stored in the 

U 

U = κ . 

The ratio V ′ 

2 

capacitor C 2 for the two cases is 

1. V ′ 

2 

V 2 

= 

2. V ′ 

2 

V 2 

= κ . 

3. V ′ 

2 

V 2 

= 2 

1 + κ . correct 

4. V ′ 

2 

V 2 

= 1 + κ 

2 κ . 

5. V 2 

′ 

= 1 + κ . 

V 2 2 


Explanation: 


For Case Two, 

Therefore 

The ratio U ′ 

capacitors for the two cases is 


2. U ′ 

U = 2 

1 + κ . 

3. U ′ 

U = 1 + κ 

2 κ . 

5. U ′ 

U = 1 + κ 

2 

U = 

Explanation: 


For Case Two, 

Therefore 

keywords: 

. 

2 κ 

1 + κ . correct 

U = 1 2 C 12 V 2 . 

U ′ = 1 2 C′ 12 V 2 . 

U ′ 

U = C′ 12 

C 12 

= 

2 κ 

1 + κ . 

Dielectric in a Capacitor 01 




a) An isolated capacitor has a dielectric slab κ 

between its plates. 

b) The capacitor is charged by a battery. 

c) After the capacitor is charged, the battery 

is removed. 

d) The dielectric slab is then moved half way 

out of the capacitor. 

e) Finally, the dielectric is released and is set 

free to move on its own. 

κ 

The dielectric will 

1. be pulled back into the capacitor. correct 

2. remain in place. 

κ


3. be pushed out of the capacitor. 

Explanation: 

The capacitance of a capacitor with a dielectric 

slab is 

C in = κ C out , where κ > 1 . 

NOTE 

When the battery is removed, the charge 

on the plates of the capacitor will remain 

constant. Charge is neither created nor destroyed. 

U out = 1 2 

U in = 1 2 

= 1 2 

Q 2 

C out 

, 

Q 2 

C in 

Q 2 

κ C out 

= 1 κ U out , so 

U in 

and 

where U out is with an air-filled gap and U in 

is with a dielectric-filled gap. A system will 

move to a position of lower potential energy. 

After the dielectric is moved half way out 

of the capacitor, the potential energy stored 

in the capacitor will be larger than it would 

have been with the dielectric left in place. 

Therefore, the dielectric will be pulled back 

into the capacitor. 

keywords: 

Dipole in an External Field 0 


fixed. 


A dipole (electrically neutral) is placed in an 

external field. 

(a) 

− + 

(b) 

− 

+ 

− + 

(c) 

− 

+ 

(d) 

For which situation(s) shown above is the 

net force on the dipole equal to zero 

1. (a) only 

2. (c) only 

3. (c) and (d) correct 

4. (a) and (c) 

5. (b) and (d) 

6. (a) and (d) 

7. (a), (b), and (c) 

8. (b), (c), and (d) 

9. Another combination 


Explanation: 

Basic Concepts: Field patterns of point 

charge and parallel plates of infinite extent. 

The force on a charge in the electric field is 

given by 

⃗F = q E ⃗ 

and the torque is defined as 

⃗T = ⃗r × ⃗ F 

∆E ⃗ = k ∆q 

r 2 ˆr 

⃗E = ∑ ∆ ⃗ E i . 

Symmetry of the configuration will cause 

some component of the electric field to be 

zero.


Gauss’ law states 

∮ 

Φ S = 

⃗E · d ⃗ A = Q ɛ 0 

. 

Solutions: The electric dipole consists of 

two equal and opposite charges separated by 

a distance. In either situation (c) or (d), the 

electric field is uniform and parallel everywhere. 

Thus, the electric force on one charge 

is equal but opposite to that on another so 

that the net force on the whole dipole is zero. 

By contrast, electric fields are nonuniform for 

situations both (a) and (b). 

keywords:

Version One â Homework 1 â Juyang Huang â 24018 â Jan 16 ...

Create successful ePaper yourself

Delete template?

Save as template?

Version One â Homework 1 â Juyang Huang â 24018 â Jan 16 ...