Math Review for Physical Chemistry
Math Review for Physical Chemistry
Math Review for Physical Chemistry
Create successful ePaper yourself
Turn your PDF publications into a flip-book with our unique Google optimized e-Paper software.
<strong>Chemistry</strong> 362<br />
Spring 2013<br />
Dr. Jean M. Standard<br />
January 28, 2013<br />
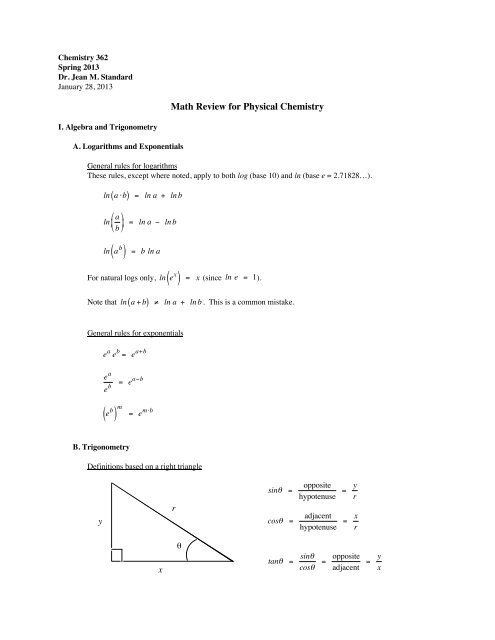
<strong>Math</strong> <strong>Review</strong> <strong>for</strong> <strong>Physical</strong> <strong>Chemistry</strong><br />
I. Algebra and Trigonometry<br />
A. Logarithms and Exponentials<br />
General rules <strong>for</strong> logarithms<br />
These rules, except where noted, apply to both log (base 10) and ln (base e = 2.71828…).<br />
ln ( a ⋅b) = ln a + ln b<br />
€<br />
€<br />
€<br />
⎛⎛<br />
ln a ⎞⎞<br />
⎜⎜ ⎟⎟ = ln a − ln b<br />
⎝⎝ b ⎠⎠<br />
ln ( a b<br />
) = b ln a<br />
For natural logs only,<br />
Note that<br />
ln a + b<br />
€<br />
ln ( e x<br />
) = x (since<br />
€<br />
ln e = 1).<br />
( ) ≠ ln a + ln b . This is a common mistake.<br />
General rules <strong>for</strong> exponentials<br />
€<br />
e a e b = e a+b<br />
€<br />
e a<br />
e b<br />
= e a−b<br />
€<br />
( e b<br />
) m = e m⋅b<br />
B. Trigonometry<br />
€<br />
Definitions based on a right triangle<br />
y<br />
r<br />
€<br />
sinθ =<br />
cosθ =<br />
opposite<br />
hypotenuse = y r<br />
adjacent<br />
hypotenuse<br />
= x r<br />
x<br />
θ<br />
€<br />
tanθ = sinθ<br />
cosθ<br />
= opposite<br />
adjacent<br />
= y x<br />
€
2<br />
Other trigonometric function definitions<br />
cotθ =<br />
1<br />
tanθ<br />
= cosθ<br />
sinθ<br />
€<br />
€<br />
secθ =<br />
cscθ =<br />
1<br />
cosθ<br />
1<br />
sinθ<br />
€<br />
Trigonometric Identities<br />
sin 2 θ + cos 2 θ = 1<br />
sin2θ = 2sinθ cosθ<br />
€<br />
cos2θ = cos 2 θ − sin 2 θ<br />
€<br />
II. Calculus [See also <strong>Math</strong>ematical Background 1 and 2 in your text.]<br />
€<br />
A. Derivatives<br />
Derivatives of common functions<br />
d<br />
dx x n<br />
= n x n−1<br />
€<br />
€<br />
€<br />
€<br />
d<br />
dx eax<br />
= a e ax<br />
d<br />
dx ln x = 1 x<br />
d<br />
sin x = cos x<br />
dx<br />
d<br />
cos x = − sin x<br />
dx<br />
€<br />
€<br />
€<br />
€<br />
General rules <strong>for</strong> manipulation of derivatives<br />
d<br />
dx c ⋅ f x<br />
d<br />
dx<br />
d<br />
dx<br />
[ ( )]<br />
= c ⋅ f ʹ′ ( x) (c is a constant)<br />
[ f ( x)<br />
+ g( x)<br />
] =<br />
[ f ( x)<br />
⋅ g( x)<br />
] = f x<br />
d<br />
dx f u x<br />
( ( ))<br />
= df<br />
du ⋅ du<br />
dx<br />
d<br />
dx f x ( ) + d dx g x ( )<br />
( ) ⋅ g ʹ′ ( x) + g( x) ⋅ f ʹ′ ( x) (the Product Rule)<br />
(the Chain Rule)<br />
€
3<br />
B. Integrals<br />
Integrals of common functions<br />
Note that since these are indefinite integrals, they all should include an overall constant of integration.<br />
∫ x n dx =<br />
1<br />
n +1 x n+1<br />
€<br />
∫ e bx dx = 1 b ebx<br />
€<br />
1<br />
∫<br />
x dx = ln x<br />
€<br />
∫ sin x dx = − cos x<br />
€<br />
∫ cos x dx = sin x<br />
€<br />
€<br />
General rules <strong>for</strong> manipulation of integrals<br />
∫ c ⋅ f ( x) dx = c ⋅ f ( x) dx<br />
∫<br />
(c is a constant)<br />
∫ [ f ( x) + g( x)<br />
] dx = ∫ f ( x) dx + ∫ g x<br />
( ) dx<br />
€
4<br />
Some More Definite and Indefinite Integrals<br />
1.<br />
∞<br />
∫<br />
0<br />
e −bx<br />
dx = 1 b<br />
€<br />
2.<br />
∞<br />
∫ x n e −bx dx =<br />
0<br />
n!<br />
b n+1<br />
€<br />
3.<br />
∞<br />
∫ e −bx 2<br />
dx = 1 2<br />
0<br />
⎛⎛ π ⎞⎞<br />
⎜⎜ ⎟⎟<br />
⎝⎝ b ⎠⎠<br />
1<br />
2<br />
€<br />
4.<br />
∞<br />
∫ x e −bx 2<br />
dx =<br />
0<br />
1<br />
2b<br />
€<br />
5.<br />
∞<br />
∫ x 2 e −bx 2<br />
dx =<br />
0<br />
1 ⎛⎛ π ⎞⎞<br />
⎜⎜ ⎟⎟<br />
4b ⎝⎝ b ⎠⎠<br />
1<br />
2<br />
€<br />
6.<br />
∫<br />
sin 2 bx dx = x 2 − sin2bx<br />
4b<br />
€<br />
7.<br />
∫<br />
x sin<br />
bx dx = sinbx<br />
b 2<br />
− x cosbx<br />
b<br />
€<br />
8.<br />
∫ x sin 2 bx dx = x 2<br />
4 − x sin2bx<br />
4b<br />
− cos2bx<br />
8b 2<br />
€<br />
9.<br />
∫<br />
sin 3<br />
bx dx = − cosbx<br />
3b<br />
[ sin 2 bx + 2]<br />
€<br />
10.<br />
∫<br />
sin<br />
bx cosbx dx = sin2 bx<br />
2b<br />
€<br />
11.<br />
∫ cos 2 bx dx = x 2 + sin2bx<br />
4b<br />
€<br />
Other sources <strong>for</strong> integrals: CRC handbooks and The Integrator at http://www.integrals.com.
5<br />
III. A Guide to Complex Numbers<br />
General Definitions<br />
All complex numbers have at their root the imaginary number i,<br />
Complex numbers are written as a real part and an imaginary part,<br />
i = −1 . (1)<br />
€<br />
z = a + i b , (2)<br />
where z is a complex number and a and b are real numbers. The number a is referred to as the real part of the<br />
complex number, while the number b is referred to as the imaginary part since it is multiplied by i.<br />
€<br />
A function may also contain imaginary numbers. The simplest types of such functions can be divided into real and<br />
imaginary parts,<br />
f ( x) and<br />
In this equation, g x<br />
referred to as the real part of the function € h x<br />
h( x) = f ( x) + i g( x) . (3)<br />
( ) are real functions. As <strong>for</strong> the complex numbers defined in Equation (2), f ( x) is<br />
( ) and g( x) is referred to as the imaginary part of the function g( x) .<br />
€ €<br />
€<br />
Euler’s Relation<br />
€ €<br />
€<br />
Functions that contain imaginary numbers may not always be easily separated into real and imaginary parts.<br />
However, a typical function used in quantum mechanics has the imaginary number in the exponent,<br />
f ( x) = e i k x , (4)<br />
where k is a constant. Even this function may be separated into real and imaginary parts using Euler’s relation,<br />
€<br />
e i k x = cos kx + i sin kx . (5)<br />
Complex Conjugates<br />
€<br />
An important quantity when dealing with complex numbers and functions is the complex conjugate. The complex<br />
conjugate of a number or function that contains an imaginary part is obtained by replacing i by –i where it appears.<br />
A complex conjugate is denoted by an asterisk. For example, <strong>for</strong> a complex number z, the complex conjugate is z*.<br />
If z = a + i b, then the complex conjugate is<br />
z * = a − i b. (6)<br />
€<br />
The complex conjugate of a function such as the one in Equation (3) is defined similarly,<br />
€<br />
h * ( x) = f ( x) − i g( x) . (7)<br />
And, <strong>for</strong> the function given in Equation (4), the complex conjugate is<br />
€<br />
f * ( x) = e −i k x . (8)<br />
€
6<br />
Absolute Squares of Complex Variables<br />
An important property of the complex conjugate of a number or a function is that when the complex conjugate is<br />
multiplied by the original number or function, the result is always real and positive. For example, consider the<br />
product of a complex number z and its complex conjugate, z ⋅ z *, which is known as the absolute square,<br />
z ⋅ z * = ( a + i b) ( a − i b)<br />
= a€<br />
2 + i ab − iab − i 2 b 2<br />
= a 2 − i 2 b 2<br />
z ⋅ z * = a 2 + b 2 .<br />
(9)<br />
The above relation simplifies using the result that = −1. The complex conjugate multiplied by the original also<br />
yields a real and positive result € <strong>for</strong> functions. For example, consider the function given in Equation (4),<br />
i 2<br />
€<br />
f ( x) ⋅ f * ( x) = e i k x e −i k x<br />
= e 0<br />
f ( x) ⋅ f * ( x) = 1.<br />
(10)<br />
€<br />
More in<strong>for</strong>mation related to complex variables may be found in <strong>Math</strong>ematical Background 3 in your textbook.