SNAKE LEMMA IN INCOMPLETE RELATIVE HOMOLOGICAL ...

90 TAMAR JANELIDZE
hold, we conclude that p 1 ψ = e f ′
Q
π 2 . Recall, that the square f ′ ϕ = vπ 1 is a pullback (see
the proof of (i)), therefore, (Y × Z K w , π 1 , ψ) is the pullback of p and v 1 , yielding that ψ is
in E. Since p 1 ψ = e f ′
Q
π 2 , and ψ, p 1 , and π 2 are in E, we conclude that e f ′
Q
is also in E,
as desired.
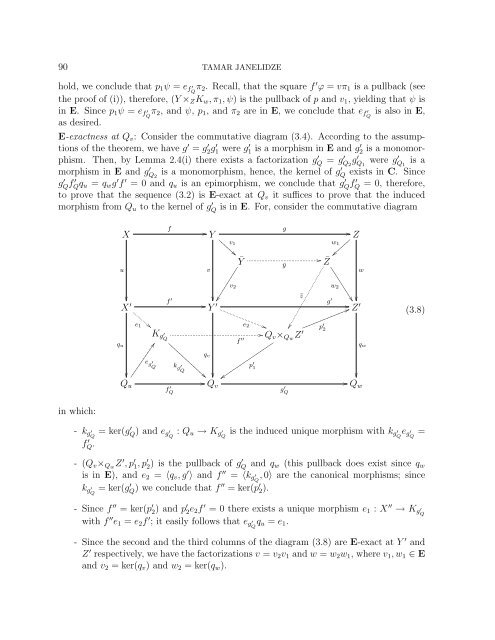
E-exactness at Q v : Consider the commutative diagram (3.4). According to the assumptions
of the theorem, we have g ′ = g 2g ′ 1 ′ were g 1 ′ is a morphism in E and g 2 ′ is a monomorphism.
Then, by Lemma 2.4(i) there exists a factorization g Q ′ = g′ Q 2
g Q ′ 1
were g Q ′ 1
is a
morphism in E and g Q ′ 2
is a monomorphism, hence, the kernel of g Q ′ exists in C. Since
g Q ′ f Q ′ q u = q w g ′ f ′ = 0 and q u is an epimorphism, we conclude that g Q ′ f Q ′ = 0, therefore,
to prove that the sequence (3.2) is E-exact at Q v it suffices to prove that the induced
morphism from Q u to the kernel of g Q ′ is in E. For, consider the commutative diagram
in which:
f
g
X
Y
Z
v 1 w 1
Ȳ
ȳ
¯Z
u
v
w
v 2
w 2
¯z
X ′
f ′ Y ′ g ′
Z ′ (3.8)
e 1
e 2
K g ′
Q
Q
q u
f ′′ v × Qw Z ′
p ′ 2
q w
q v
e g ′
k g ′ p ′ Q 1
Q
Q u
Q
f Q
′ v
Q w
- k g ′
Q
= ker(g ′ Q ) and e g ′ Q : Q u → K g ′
Q
is the induced unique morphism with k g ′
Q
e g ′
Q
=
f ′ Q .
- (Q v × Qw Z ′ , p ′ 1, p ′ 2) is the pullback of g ′ Q and q w (this pullback does exist since q w
is in E), and e 2 = 〈q v , g ′ 〉 and f ′′ = 〈k g ′
Q
, 0〉 are the canonical morphisms; since
k g ′
Q
= ker(g ′ Q ) we conclude that f ′′ = ker(p ′ 2).
g ′ Q
- Since f ′′ = ker(p ′ 2) and p ′ 2e 2 f ′ = 0 there exists a unique morphism e 1 : X ′′ → K g ′
Q
with f ′′ e 1 = e 2 f ′ ; it easily follows that e g ′
Q
q u = e 1 .
- Since the second and the third columns of the diagram (3.8) are E-exact at Y ′ and
Z ′ respectively, we have the factorizations v = v 2 v 1 and w = w 2 w 1 , where v 1 , w 1 ∈ E
and v 2 = ker(q v ) and w 2 = ker(q w ).