Perfect Matchings in Lattice Animals and Lattice Paths with Constraints
Perfect Matchings in Lattice Animals and Lattice Paths with Constraints
Perfect Matchings in Lattice Animals and Lattice Paths with Constraints
- No tags were found...
Create successful ePaper yourself
Turn your PDF publications into a flip-book with our unique Google optimized e-Paper software.
252 T. DO[LI]<br />
called <strong>in</strong>ternal. Borrow<strong>in</strong>g a term from the chemistry of<br />
aromatic hydrocarbons, we call the animal graphs <strong>with</strong>out<br />
<strong>in</strong>ternal vertices catacondensed. Otherwise, the graph<br />
is pericondensed. In the rest of this paper, when referr<strong>in</strong>g<br />
to lattice animals we will, <strong>in</strong> fact, be referr<strong>in</strong>g to the<br />
correspond<strong>in</strong>g animal graphs.<br />
In the follow<strong>in</strong>g sections our attention will be (not<br />
always fairly) divided between the hexagonal <strong>and</strong> the<br />
square lattice animals. In section <strong>Lattice</strong> <strong>Animals</strong> <strong>and</strong><br />
Ear Decompositions we use certa<strong>in</strong> decomposition techniques<br />
to establish the existence of perfect match<strong>in</strong>gs <strong>in</strong><br />
certa<strong>in</strong> classes of lattice animals <strong>and</strong> to derive simple<br />
lower bounds on their number. Then we proceed, <strong>in</strong> section<br />
Benzenoid Parallelograms, by demonstrat<strong>in</strong>g a correspondence<br />
between perfect match<strong>in</strong>gs <strong>in</strong> some special<br />
classes of hexagonal animals <strong>and</strong> lattice paths <strong>in</strong> rectangular<br />
lattices. The correspondence is then used to obta<strong>in</strong><br />
explicit formulas for the number of perfect match<strong>in</strong>gs <strong>in</strong><br />
some classes of hexagonal animals <strong>in</strong> terms of some well-<br />
-known comb<strong>in</strong>atorial sequences, <strong>and</strong> to establish certa<strong>in</strong><br />
identities <strong>in</strong>volv<strong>in</strong>g Fibonacci numbers. We conclude<br />
<strong>with</strong> a section on polyom<strong>in</strong>oes, us<strong>in</strong>g the correspondence<br />
<strong>with</strong> lattice paths to show how Catalan, Schröder <strong>and</strong><br />
Delannoy numbers appear as the enumerat<strong>in</strong>g sequences<br />
of perfect match<strong>in</strong>gs <strong>in</strong> some special polyom<strong>in</strong>oes.<br />
LATTICE ANIMALS AND EAR DECOMPOSITIONS<br />
A perfect match<strong>in</strong>g <strong>in</strong> a graph G is a collection M of<br />
edges of G such that every vertex of G is <strong>in</strong>cident <strong>with</strong><br />
exactly one edge from M. An edge e of G which appears<br />
<strong>in</strong> some perfect match<strong>in</strong>g of G is called allowed; otherwise,<br />
it is forbidden. A graph G is elementary if its allowed<br />
edges form a connected subgraph of G. For bipartite<br />
graphs, elementarity is equivalent to the fact that all<br />
its edges are allowed. 10<br />
There are many criteria for decid<strong>in</strong>g if a given lattice<br />
animal possesses a perfect match<strong>in</strong>g. We present<br />
here some results that follow from the ear decomposition<br />
techniques. We refer the reader to Ref. 10 for a full<br />
description of these techniques.<br />
Let G be a bipartite graph <strong>and</strong> G' subgraph of G. An<br />
ear of G relative to G' is any odd-length path <strong>in</strong> G hav<strong>in</strong>g<br />
both endpo<strong>in</strong>ts – but no <strong>in</strong>terior po<strong>in</strong>t – <strong>in</strong> G'. A bipartite<br />
ear decomposition of G start<strong>in</strong>g <strong>with</strong> G' is a representation<br />
of G <strong>in</strong> the form G = G'+P 1 +…+P k , where P 1<br />
is an ear of G'+P 1 relative to G', <strong>and</strong> P i is an ear of<br />
G'+P 1 +…+P i relative to G'+P 1 +…+P i–1 for 2 i k.<br />
An ear decomposition of a given graph is not unique.<br />
Proposition 2.1<br />
Every catacondensed lattice animal has a bipartite ear<br />
decomposition start<strong>in</strong>g <strong>with</strong> any edge.<br />
Proof<br />
Every catacondensed benzenoid graph <strong>with</strong> h hexagons<br />
can be constructed start<strong>in</strong>g from an arbitrary edge, add<strong>in</strong>g<br />
an ear of length five to obta<strong>in</strong> the first hexagon <strong>and</strong><br />
then add<strong>in</strong>g one hexagon at every further step. But add<strong>in</strong>g<br />
a hexagon means add<strong>in</strong>g only five new edges to the<br />
graph already constructed. It is obvious that these edges<br />
make an ear <strong>in</strong> the above sense. So, every catacondensed<br />
benzenoid has a bipartite ear decomposition start<strong>in</strong>g from<br />
any of its edges. Similarly, every catacondensed polyom<strong>in</strong>o<br />
can be constructed start<strong>in</strong>g from a s<strong>in</strong>gle edge <strong>and</strong><br />
add<strong>in</strong>g ears consist<strong>in</strong>g of three edges, one at a time.<br />
Hence, the claim is also valid for polyom<strong>in</strong>oes. <br />
We refer the reader to the p. 124 of Ref. 10 for the<br />
proof of the fact that a graph is elementary bipartite if<br />
<strong>and</strong> only if it has a bipartite ear decomposition. S<strong>in</strong>ce<br />
each ear <strong>in</strong> a bipartite ear decomposition contributes at<br />
least one new perfect match<strong>in</strong>g, we get a lower bound<br />
for the number of perfect match<strong>in</strong>gs <strong>in</strong> a catacondensed<br />
lattice animal.<br />
Corollary 2.2<br />
There are at least h + 1 perfect match<strong>in</strong>gs <strong>in</strong> a catacondensed<br />
lattice animal <strong>with</strong> h basic polygons. <br />
The lower bound from the Corollary 2.2 is a sharp<br />
one, <strong>in</strong> the sense that there are catacondensed benzenoids<br />
for which this bound is atta<strong>in</strong>ed. Namely, it is easy to<br />
see that a straight l<strong>in</strong>ear cha<strong>in</strong> of h hexagons conta<strong>in</strong>s<br />
exactly h+1 perfect match<strong>in</strong>gs. In the case of polyom<strong>in</strong>oes,<br />
the lower bound is atta<strong>in</strong>ed for the class of zig-zag<br />
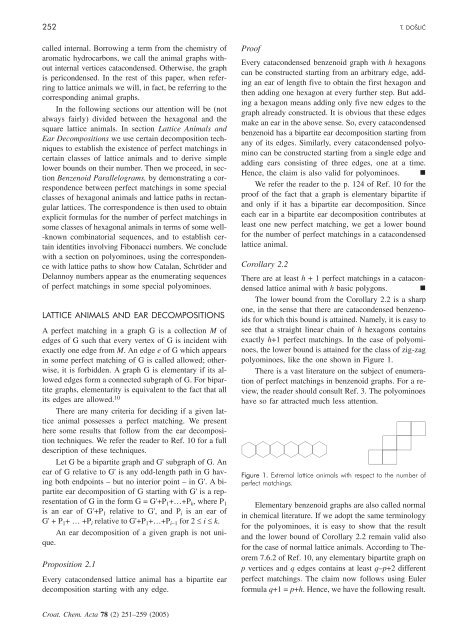
polyom<strong>in</strong>oes, like the one shown <strong>in</strong> Figure 1.<br />
There is a vast literature on the subject of enumeration<br />
of perfect match<strong>in</strong>gs <strong>in</strong> benzenoid graphs. For a review,<br />
the reader should consult Ref. 3. The polyom<strong>in</strong>oes<br />
have so far attracted much less attention.<br />
Figure 1. Extremal lattice animals <strong>with</strong> respect to the number of<br />
perfect match<strong>in</strong>gs.<br />
Elementary benzenoid graphs are also called normal<br />
<strong>in</strong> chemical literature. If we adopt the same term<strong>in</strong>ology<br />
for the polyom<strong>in</strong>oes, it is easy to show that the result<br />
<strong>and</strong> the lower bound of Corollary 2.2 rema<strong>in</strong> valid also<br />
for the case of normal lattice animals. Accord<strong>in</strong>g to Theorem<br />
7.6.2 of Ref. 10, any elementary bipartite graph on<br />
p vertices <strong>and</strong> q edges conta<strong>in</strong>s at least q–p+2 different<br />
perfect match<strong>in</strong>gs. The claim now follows us<strong>in</strong>g Euler<br />
formula q+1 = p+h. Hence, we have the follow<strong>in</strong>g result.<br />
Croat. Chem. Acta 78 (2) 251–259 (2005)