Perfect Matchings in Lattice Animals and Lattice Paths with Constraints
Perfect Matchings in Lattice Animals and Lattice Paths with Constraints
Perfect Matchings in Lattice Animals and Lattice Paths with Constraints
- No tags were found...
You also want an ePaper? Increase the reach of your titles
YUMPU automatically turns print PDFs into web optimized ePapers that Google loves.
258 T. DO[LI]<br />
a)<br />
(0,0)<br />
(6,0)<br />
(0,0) (6,0)<br />
b)<br />
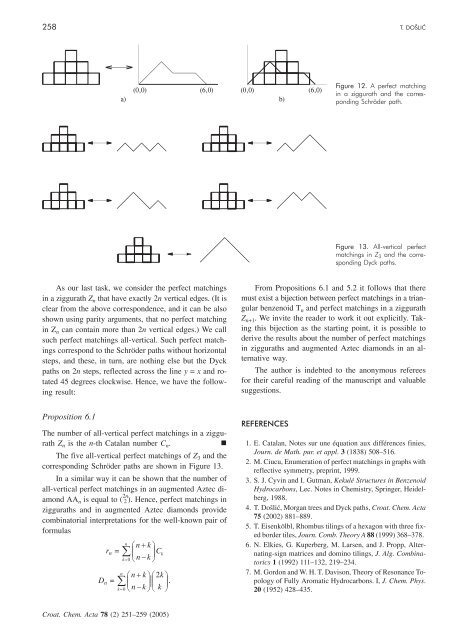
Figure 12. A perfect match<strong>in</strong>g<br />
<strong>in</strong> a ziggurath <strong>and</strong> the correspond<strong>in</strong>g<br />
Schröder path.<br />
Figure 13. All-vertical perfect<br />
match<strong>in</strong>gs <strong>in</strong> Z 3 <strong>and</strong> the correspond<strong>in</strong>g<br />
Dyck paths.<br />
As our last task, we consider the perfect match<strong>in</strong>gs<br />
<strong>in</strong> a ziggurath Z n that have exactly 2n vertical edges. (It is<br />
clear from the above correspondence, <strong>and</strong> it can be also<br />
shown us<strong>in</strong>g parity arguments, that no perfect match<strong>in</strong>g<br />
<strong>in</strong> Z n can conta<strong>in</strong> more than 2n vertical edges.) We call<br />
such perfect match<strong>in</strong>gs all-vertical. Such perfect match<strong>in</strong>gs<br />
correspond to the Schröder paths <strong>with</strong>out horizontal<br />
steps, <strong>and</strong> these, <strong>in</strong> turn, are noth<strong>in</strong>g else but the Dyck<br />
paths on 2n steps, reflected across the l<strong>in</strong>e y = x <strong>and</strong> rotated<br />
45 degrees clockwise. Hence, we have the follow<strong>in</strong>g<br />
result:<br />
From Propositions 6.1 <strong>and</strong> 5.2 it follows that there<br />
must exist a bijection between perfect match<strong>in</strong>gs <strong>in</strong> a triangular<br />
benzenoid T n <strong>and</strong> perfect match<strong>in</strong>gs <strong>in</strong> a ziggurath<br />
Z n+1 . We <strong>in</strong>vite the reader to work it out explicitly. Tak<strong>in</strong>g<br />
this bijection as the start<strong>in</strong>g po<strong>in</strong>t, it is possible to<br />
derive the results about the number of perfect match<strong>in</strong>gs<br />
<strong>in</strong> zigguraths <strong>and</strong> augmented Aztec diamonds <strong>in</strong> an alternative<br />
way.<br />
The author is <strong>in</strong>debted to the anonymous referees<br />
for their careful read<strong>in</strong>g of the manuscript <strong>and</strong> valuable<br />
suggestions.<br />
Proposition 6.1<br />
The number of all-vertical perfect match<strong>in</strong>gs <strong>in</strong> a ziggurath<br />
Z n is the n-th Catalan number C n .<br />
<br />
The five all-vertical perfect match<strong>in</strong>gs of Z 3 <strong>and</strong> the<br />
correspond<strong>in</strong>g Schröder paths are shown <strong>in</strong> Figure 13.<br />
In a similar way it can be shown that the number of<br />
all-vertical perfect match<strong>in</strong>gs <strong>in</strong> an augmented Aztec diamond<br />
AA n is equal to ( 2 2 n ). Hence, perfect match<strong>in</strong>gs <strong>in</strong><br />
zigguraths <strong>and</strong> <strong>in</strong> augmented Aztec diamonds provide<br />
comb<strong>in</strong>atorial <strong>in</strong>terpretations for the well-known pair of<br />
formulas<br />
n<br />
n<br />
k<br />
r n = <br />
<br />
n k C k<br />
k <br />
0<br />
<br />
D n =<br />
n<br />
<br />
k0<br />
n<br />
k<br />
2k<br />
<br />
<br />
n<br />
k<br />
<br />
k .<br />
<br />
REFERENCES<br />
1. E. Catalan, Notes sur une équation aux différences f<strong>in</strong>ies,<br />
Journ. de Math. pur. et appl. 3 (1838) 508–516.<br />
2. M. Ciucu, Enumeration of perfect match<strong>in</strong>gs <strong>in</strong> graphs <strong>with</strong><br />
reflective symmetry, prepr<strong>in</strong>t, 1999.<br />
3. S. J. Cyv<strong>in</strong> <strong>and</strong> I. Gutman, Kekulé Structures <strong>in</strong> Benzenoid<br />
Hydrocarbons, Lec. Notes <strong>in</strong> Chemistry, Spr<strong>in</strong>ger, Heidelberg,<br />
1988.<br />
4. T. Do{li}, Morgan trees <strong>and</strong> Dyck paths, Croat. Chem. Acta<br />
75 (2002) 881–889.<br />
5. T. Eisenkölbl, Rhombus til<strong>in</strong>gs of a hexagon <strong>with</strong> three fixed<br />
border tiles, Journ. Comb. Theory A 88 (1999) 368–378.<br />
6. N. Elkies, G. Kuperberg, M. Larsen, <strong>and</strong> J. Propp, Alternat<strong>in</strong>g-sign<br />
matrices <strong>and</strong> dom<strong>in</strong>o til<strong>in</strong>gs, J. Alg. Comb<strong>in</strong>atorics<br />
1 (1992) 111–132, 219–234.<br />
7. M. Gordon <strong>and</strong> W. H. T. Davison, Theory of Resonance Topology<br />
of Fully Aromatic Hydrocarbons. I, J. Chem. Phys.<br />
20 (1952) 428–435.<br />
Croat. Chem. Acta 78 (2) 251–259 (2005)