Vol. 7 No 5 - Pi Mu Epsilon

We begin by stating a fairly obvious fact.
Theoklim 1.
A quadrilateral which is both cyclic and equilic is
an isosceles trapezoid with A = B = 60°
The proof of Theorem 1 is left to the reader.
Ve&kni^ion. If one angle of an equilic quadrilateral is equal to
90° the quadrilateral is called right equilic.
Th~oklim 2. In a right equilic quadrilateral, a diagonal is equal
to an unequal side.
FIGURE 2A
8
CK = CB, so B and K coincide, making angle DAB = angle DAK = 90'.
In the next two theorems we investigate some interesting relations
between the equilic quadrilateral and equilateral triangles.
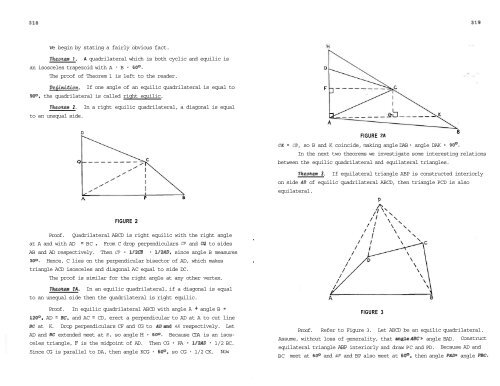
Tfteo~em 3. If equilateral triangle ABP is constructed interiorly
on side A5 of equilic quadrilateral ABCD, then triangle PCD is also
equilateral.
FIGURE 2
Proof. Quadrilateral ABCD is right equilic with the right angle
at A and with AD = BC . From C drop perpendiculars CP and CQ to sides
AB and AD respectively. Then CP = 1/2CB = 1/2AD, since angle B measures
f
30° Hence, C lies on the perpendicular bisector of AD, which makes Ã
triangle ACD isosceles and diagonal AC equal to side DC.
The proof is similar for the right angle at any other vertex.
Thokern 2A. In an equilic quadrilateral, if a diagonal is equal
to an unequal side then the quadrilateral is right equilic.
Proof. In equilic quadrilateral ABCD with angle A + angle B =
120° AD = BC, and AC = CD, erect a perpendicular to AD at A to cut line
BC at K. Drop perpendiculars CF and CG to ADand AK respectively. Let
AD and BC extended meet at H, so angle H = 600. Because CDA is an isosceles
triangle, F is the midpoint of AD. Then CG = FA = 1/2AD = 1/2 BC.
Since CG is parallel to DA, then angle KCG = 60° so CG = 1/2 CK. Now
FIGURE 3
Proof. Refer to Figure 3. Let ABCD be an equilic quadrilateral.
Assume, without loss of generality, that angleABC> angle BAD. Construct
equilateral triangle ABP interiorly and draw PC and PD. Because AD and
BC meet at 60 and AP and BP also meet at 60° then angle PAD= angle PBC.