Vol. 7 No 5 - Pi Mu Epsilon

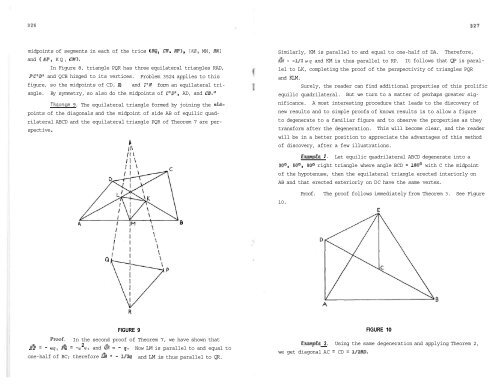
midpoints of segments in each of the trios (BQ, CN, RP), (KB, MN, RA)
and (AP, KQ, CM).
In Figure 8, triangle PQR has three equilateral triangles RAD,
PCID' and QCB hinged to its vertices. Problem 3524 applies to this
figure, so the midpoints of CD, AD',
angle. By symmetry, so also do the midpoints of C'D',
and CIB form an equilateral tri-
AD, and CB.I1
The-otem 9. The equilateral triangle formed by joining the rnid-
points of the diagonals and the midpoint of side AB of equilic quad-
rilateral ABCD and the equilateral triangle PQR of Theorem 7 are perspect
ive .
Similarly, KM is parallel to and equal to one-half of DA. Therefore,
iSf = -1/2 n>q and KM is thus parallel to RP. It follows that QP is parallel
to LK, completing the proof of the perspectivity of triangles PQR
and KLM.
Surely, the reader can find additional properties of this prolific
equilic quadrilateral. But we turn to a matter of perhaps greater sig-
nificance. A most interesting procedure that leads to the discovery of
new results and to simple proofs of known results is to allow a figure
to degenerate to a familiar figure and to observe the properties as they
transform after the degeneration. This will become clear, and the reader
will be in a better position to appreciate the advantages of this method
of discovery, after a few illustrations.
EXamph 1. Let equilic quadrilateral ABCD degenerate into a
30° 60° 900 right triangle where angle BCD = 180' with C the midpoint
of the hypotenuse, then the equilateral triangle erected interiorly on
AB and that erected exteriorly on DC have the same vertex.
10.
Proof.
The proof follows immediately from Theorem 3. See Figure
Proof.
FIGURE 9
In the second proof of Theorem 7, we have shown that
R^P = - q, P& = -o) q, and $& = - q.
one-half of BC; therefore & = - l/2q
Now LM is parallel to and equal to
and LM is thus parallel to QR.
FIGURE 10
EXamp& 2. Using the same degeneration and applying Theorem 2,
we get diagonal AC = CD = 1/2BD.