Chapter 3 - Experimental Error - WH Freeman

56
3 Experimental Error
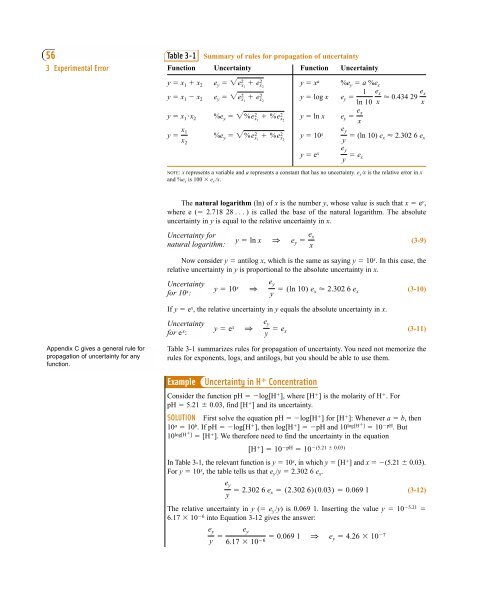
Table 3-1
Summary of rules for propagation of uncertainty
Function Uncertainty Function Uncertainty
y x 1 x 2 e y 2e 2 x y x a
1
e 2 x 2
%e y a %e x
1 e x
e x
y x 1 x 2 e y 2e 2 x 1
e 2 x 2
y log x e y 0.434 29
ln 10 x
x
e x
y x 1 x 2 %e y 2%e 2 x 1
%e 2 x 2
y ln x e y
x
x 1
e y
y %e y 2%e 2 x y 10 x
1
%e 2 x 2
(ln 10) e x 2.302 6 e
x y
x
2
e y
y e x e
y x
NOTE: x represents a variable and a represents a constant that has no uncertainty. e x /x is the relative error in x
and %e x is 100 e x /x.
The natural logarithm (ln) of x is the number y, whose value is such that x e y ,
where e ( 2.718 28 ...) is called the base of the natural logarithm. The absolute
uncertainty in y is equal to the relative uncertainty in x.
Uncertainty for
natural logarithm:
y ln x1 e y e x
x
(3-9)
Now consider y antilog x, which is the same as saying y 10 x . In this case, the
relative uncertainty in y is proportional to the absolute uncertainty in x.
Uncertainty
for 10 x :
y 10 x 1 e y
y (ln 10) e x 2.302 6 e x
(3-10)
If y e x , the relative uncertainty in y equals the absolute uncertainty in x.
Uncertainty
for e x :
y e x 1 e y
y e x
(3-11)
Appendix C gives a general rule for
propagation of uncertainty for any
function.
Table 3-1 summarizes rules for propagation of uncertainty. You need not memorize the
rules for exponents, logs, and antilogs, but you should be able to use them.
Example
Uncertainty in H Concentration
Consider the function pH log[H ], where [H ] is the molarity of H . For
pH 5.21 0.03, find [H ] and its uncertainty.
SOLUTION First solve the equation pH log[H ] for [H ]: Whenever a b, then
10 a 10 b . If pH log[H ], then log[H ] pH and 10 log[H ]
10 pH . But
10 log[H ]
[H ]. We therefore need to find the uncertainty in the equation
[H ] 10 pH 10(5.21 0.03)
In Table 3-1, the relevant function is y 10 x , in which y [H ] and x (5.21 0.03).
For y 10 x , the table tells us that e y /y 2.302 6 e x .
e y
y 2.302 6 e x (2.302 6)(0.03) 0.069 1
(3-12)
The relative uncertainty in y ( e y /y) is 0.069 1. Inserting the value y 10 5.21
6.17 10 6 into Equation 3-12 gives the answer:
e y
y
e y
6.17 10 0.069 11 e 6 y 4.26 10 7