Advanced SVC models for newton-raphson load flow and ... - ITCJ
Advanced SVC models for newton-raphson load flow and ... - ITCJ
Advanced SVC models for newton-raphson load flow and ... - ITCJ
Create successful ePaper yourself
Turn your PDF publications into a flip-book with our unique Google optimized e-Paper software.
134<br />
IEEE TRANSACTIONS ON POWBK SYSTEMS, VOL. 15, NO. I. PCDRUARY znnn<br />
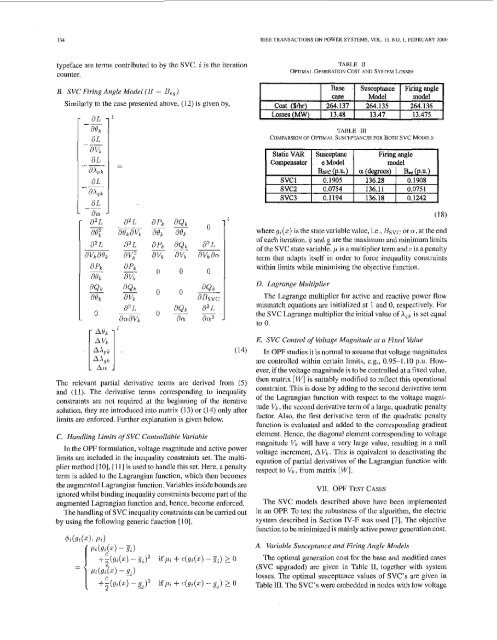
TAULE If<br />
OWIMAI. GENERATION COST AND SYSrF.M LOSSES<br />
- aL -I<br />
-~<br />
asi,<br />
a I,<br />
a C%<br />
BL<br />
--<br />
-- -<br />
ilX,i<br />
dL<br />
--<br />
a&<br />
aL -_<br />
- act -<br />
-<br />
Base<br />
CaSe<br />
cost (Sh) 264.137<br />
Losses (MW) 13.48<br />
Compensator<br />
svc 1<br />
svc2<br />
svc3<br />
Susceptance Firing angle<br />
Model model<br />
264.135 264.136<br />
13.47 13.475<br />
TABLE Ill<br />
COMPARSION OF OPTIMAI. SUSCEPTANCBS FOR BOT?[ <strong>SVC</strong> MOIX1.S<br />
I Staticvm I ~usceptanc I Firing angle I<br />
e Model<br />
model<br />
Bsvc (P.U.) a (degrees) Blb (P.U.)<br />
0.1905 136.28 0.1908<br />
0.0754 136.11 0.0751<br />
0.1194 136.18 0.1242<br />
(1 8)<br />
wheregi(x) isthestatevariablevalue, i.e., Hsvc orru,attheend<br />
of each iteration. 4 <strong>and</strong> g are the maximum <strong>and</strong> minimum limits<br />
of the <strong>SVC</strong> state variable. p is a multiplier term <strong>and</strong> c is a pcnalty<br />
term that adapts itself in order to <strong>for</strong>ce inequality constraints<br />
within limits while minimising the objective function.<br />
D. Lugrange Multiplier<br />
The Lagrange multiplier <strong>for</strong> active <strong>and</strong> reactive powcr <strong>flow</strong><br />
mismatch equations are initialized at 1 <strong>and</strong> 0, respectively. For<br />
the <strong>SVC</strong> Lagrangc multiplier the initial valiic of A,,, is set equal<br />
to 0.<br />
(14)<br />
The relevant partial derivative terms are derived from (5)<br />
<strong>and</strong> (I I). The derivative terms corrcsponding to ineqtiality<br />
constraints are not required at the beginning of the iterative<br />
solution, they are introduced into matrix (I 3) or (14) only after<br />
limits arc en<strong>for</strong>ced. Further explanation is given below.<br />
C. H<strong>and</strong>ling Limits of <strong>SVC</strong> Controllable Variable<br />
In the OPF <strong>for</strong>innlation, voltage magnitude <strong>and</strong> active power<br />
limits are included in the inequality constraints set. The inultiplier<br />
method 1101, [I I] is used to h<strong>and</strong>le this set. Here, a penalty<br />
term is added to the Lagrangian function, which then becomes<br />
the augmented Lagrangian function. Variables inside bounds are<br />
ignored whilst binding ineqtiality constraints become part of the<br />
augmented Lagrangian function <strong>and</strong>, hence, hecome en<strong>for</strong>ced.<br />
The h<strong>and</strong>ling of <strong>SVC</strong> inequality constraints can be carried out<br />
by using the following generic function 1101,<br />
di(Si(Xj, lJij<br />
3;)' ifpi + c(gi(z) - B ~) >_ o<br />
c<br />
+,(Si(xj -G)~ ifpi + c(s;(z) - -%9.) 2 U<br />
E. <strong>SVC</strong> Control of Voltage Magnitude ut a Fixed Value<br />
In OPF studies it is normal to asstime that voltage magnitudes<br />
arc controlled within certain limits, e.g., 0.95-1.10 p.u. However,<br />
if the voltage magnitude is to he controlled at a Fixed value,<br />
then matrix [W] is suitably modified to reflect this opcrational<br />
constraint. This is done by adding to the second derivative term<br />
of the Lagrangian function with respect to the voltage inagnitude<br />
Vi, the second derivative term of a large, quadratic penalty<br />
factor. Also, the first derivative term of the quadratic pcnally<br />
function is evaluated <strong>and</strong> added to the corresponding gradient<br />
element. Hence, the diagonal element corresponding to voltage<br />
magnitude Vi, will have a very large value, resulting in a null<br />
voltage increment, A l/k. This is equivalent to deactivating the<br />
equation of partial derivatives of the Lagrangian function with<br />
respect to K:, from matrix [I&'].<br />
VI1. OPF TRST CASES<br />
The <strong>SVC</strong> <strong>models</strong> described above have been implemented<br />
in an OPE To test the robustness of the algorithm, the electric<br />
system described in Section IV-F was used [7]. The objective<br />
function to be minimized is mainly active power generation cost.<br />
A. Variable Susceptance <strong>and</strong> Firing Angle Models<br />
The optimal generation cost <strong>for</strong> the base <strong>and</strong> modified cases<br />
(<strong>SVC</strong> upgraded) are given in Table 11, together with system<br />
losses. The optimal susceptance values of <strong>SVC</strong>'s are given in<br />
Table 111. The <strong>SVC</strong>'s were embedded in nodes with low voltage