Section 7: Solving Oblique Triangles

7. SOLVING OBLIQUE TRIANGLES: THE LAW OF SINES
o
An oblique triangle is one without an angle of measure 90 . When either two angles and
a side are known (AAS) in the triangle ABC or two sides and the angle opposite one
of them (SSA) is given, then the law of sines may be applied to solve the triangle.
Theorem 7.1: The Law of Sines
In the general triangle ABC
sinα
a
=
sin β
b
=
sin γ
c
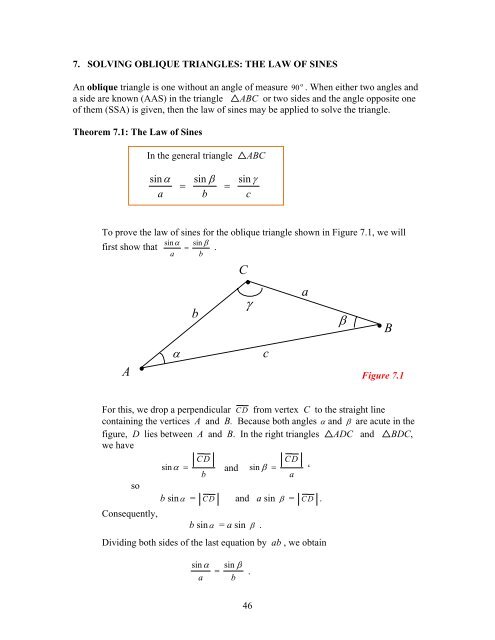
To prove the law of sines for the oblique triangle shown in Figure 7.1, we will
first show that
sinα sin β
= .
a b
b
C
.
γ
a
β
.
B
A
.
α
c
Figure 7.1
For this, we drop a perpendicular CD from vertex C to the straight line
containing the vertices A and B. Because both angles α and β are acute in the
figure, D lies between A and B. In the right triangles ADC and BDC,
we have
sin α =
C D
C D
and sin β =
b
a
‘
so
b sinα = C D and a sin β = C D .
Consequently,
b sinα = a sin β .
Dividing both sides of the last equation by ab , we obtain
sinα sin β
= .
a b
46

.
E
C
.
γ ′
a
h
l
.
A
α
b
D
β
.
B
Figure 7.2
Now to verify that
sin α sin γ
a c
= , we observe that angle γ is obtuse. Let BE
be
the perpendicular from vertex B to the line l containing side AC . Let
Then
h
o
h
sin γ ′ = = sin ( 180 −γ
) = sin γ and sin α =
a
c
so a sin γ = c sin α .
sin α sin γ
Thus, = .
a c
The proof of the law of sines in the case of an acute triangle ABC is similar.
B E = h.
Notice that the law of sines (Theorem 7.1) can be written in the alternative form:
a
sinα
=
b
sin β
=
c
sin γ
.
Solving a Triangle (AAS)
If two angles of the triangle ABC are given, then the third angle can be found by using
the relationship:
o
α + β + γ = 180 ;
47

hence, the three denominators sinα , sin β , and sin γ can be found using a calculator.
Now, if any one of the sides a, b, or c is also given, then the equations
a
sinα
=
b
sin β
=
c
sin γ
can be solved for the remaining two sides.
The following example indicates the procedure for solving a triangle when two angles
and one side are given (or can be determined from the information provided.) Unless
otherwise indicated, we shall round off all angles to the nearest hudredth of a degree, and
all side lengths to four significant digits.
Example 7.1 ---------------------------- ------------------------------------------------------------
In ABC , suppose that α = 41 o
o
, β = 77 , and a = 74. Solve for γ , b, and c.
B
c
β = 77 o
a = 74
Figure 7.3
A
o
α = 41
b
γ
C
o
Solution γ = −
o
o o
77 = 62
180 41 −
a
By the law of sines,
sin 41
hence, since a = 74,
b =
and
c =
a sin 77
sin 41
sin 41
o
a sin 62
o
o
o
=
=
sin 41
o
74 sin 77
sin 41
o
74sin 62
o
o
o
b c
= = ;
o
o
sin 77 sin 62
= 109.9
= 99.59 .
___________________________________________________________________________________________________________________________________________________________________________________________________________________________________________________________________________________________
Note that in this case there is always a unique solution by the Angle-Side-Angle criteria
for congruent triangles.
Solving a Triangle (SSA)
Because there are several possibilities, the situation in which you are given the lengths of
two sides of a triangle and the angle opposite one of them is called the ambiguous case.
For instance, suppose you are given side a, side b, and angle α in ABC . You might try
to construct ABC from this information by drawing a line segment A C of length b
and a ray l that starts at A and makes an angle α with A C (Figure 7.4). To find the
48

emaining vertex B , you could use a compass to draw an arc of a circle of radius a with
center C. If the arc intersects the ray l at point B, then ABC is the desired triangle.
C
b
γ
a
Figure 7.4
A
α
c
β
B
l
I As figure 7.5 illustrates, there are actually four possibilities if you try to construct
ABC by the above method in case α is acute:
( i ) The circle does not intersect the ray l at all and there is no triangle
ABC (Figure 7.5a).
( ii ) The circle intersects the ray l in exactly one point B and there is just one
right triangle ABC (Figure 7.5b)
( iii ) The circle intersects the ray l in two points B 1 and B 2 and there are
two triangles A B 1 C and A B 2 C (Figure 7.5c).
( iv ) The circle intersects the ray l in exactly one point B and there is just one
acute triangle ABC (Figure 7.5d).
Figure 7.5
C
C
A
α
b
a
l
A
α
b
a
.
B
l
( a ) a < b sin α ⇒ no triangle possible ( b ) a = b sin α ⇒ one right triangle
49

A
α
b
C
a
. .
B 1 B 2
l
A
α
b
C
a
.
B
l
( c ) b sin α < a < b ⇒ two triangles ( d ) a > b ⇒ one acute triangle
II In case α is obtuse, then there are only two possibilities as shown in Figure 7.6.
Figure 7.6
C
b
A
α
a
.
B
l
C
b
A
a
l
( a ) a > b ⇒ one triangle ( b ) a < b ⇒ no triangle possible
In the ambiguous case, you can always use a calculator to solve the triangle ABC. Just
use the law of sines,
sinα sin β
=
a b
⎛ sinα ⎞
to evaluate sin β : sin β = b ⎜ ⎟
⎝ a ⎠
, 0 < β <
o
180 .
50

⎛ sinα ⎞
Recall that the sine of an angle is never greater than 1; hence, if b ⎜ ⎟ > 1, then this
⎝ a ⎠
trigonometric equation has no solution, and no triangle satisfies the given conditions. If
⎛ sinα ⎞
⎛ sinα ⎞
o
b ⎜ ⎟ = 1, then the equation has only one solution, β = 90 . If b ⎜ ⎟ < 1, then
⎝ a ⎠ ⎝ a ⎠
the trigonometric equation has two solutions. Namely,
⎛ sinα ⎞
β 1 = arcsin ⎜b
o
⎟ and β 2 = 180 − β 1 .
⎝ a ⎠
Once you have determined β ( or β 1 and β 2 ), you know two angles and two sides of the
triangle (or triangles), and you can solve the triangle by using the methods previously
explained. Even if there are two solutions β 1 and β 2 of the trigonometric equation for β,
it is possible that only one of these solutions corresponds to an actual triangle satisfying
the given conditions (see Figure 7.5d and Figure 7.6a).
Example 7.2 ---------------------------- ------------------------------------------------------------
Solve the triangle in each case.
o
1. α = 30 , a = 8, b = 5.
Since α is acute and a > b there is only one acute triangle
constructible.
o
sin β sin 30
=
5 8
⎛ 5 ⎞⎛
1 ⎞ 5
⇒ sin β = ⎜ ⎟⎜
⎟ =
⎝ 8 ⎠⎝
2 ⎠ 16
A
⎛ 5 ⎞
o
⇒ β = arcsin ⎜ ⎟ = 18.21 .
⎝16
⎠
o o
Then = 180 o o
γ − 30 −18.21
= 131 .79 .
o
o
⎛
o
sin131.79 sin 30
⎞
Finally, ⎜
sin131.79
= ⇒ c = 8
⎟ = 11.93.
o
c 8
⎝ sin 30 ⎠
C
γ
5 8
o
30
c
β
B
___________________________________________________________________________________________________________________________________________________________________________________________________________________________________________________________________________________________
2. α =
o
30 , a = 5, b = 8.
8
Here α is acute and b sin a = < a < b 2
⇒ there are two triangles constructible.
o
sin 30 sin β
=
5 8
⎛ 8 ⎞ ⎛ 1 ⎞
⇒ sin β = ⎜ ⎟ ⎜ ⎟
⎝ 5 ⎠ ⎝ 2 ⎠
4
= .
5
A
o
30
8 5
5
β 2
B’
β 1
γ 2
C
γ 1
β 1
B
51

Thus, β 1 = arcsin
⎛ 4 ⎞
⎜ ⎟
⎝ 5 ⎠
sin30
5
o
= 53 .13 . Then γ 1 =
o
o
sin96.87
=
c
To find the measure of β 2 observe that
o
180 o o
− 30 − 53.13 = 96 .87 and
⎛ sin96.87
⇒ c = 5 ⎜
o
⎝ sin30
o
o
⎞
⎟
= 9.928
⎠
sin β 2 = sin ( π − β1
) = sinπ ⋅ cos β 1 – cos π ⋅ sin β 1 = sin β 1 .
o
o
Thus, β 2 = 180 − β 1 = 180 o o
− 53.13 = 126 .87
o
o
and γ 2 = 180 o o
− 30 −126.87
= 23 .13 .
o
o
sin30 sin 23.13 ⎛
o
sin 23.13 ⎞
Then = ⇒ c = 5 ⎜ ⎟ = 3. 928 .
5
o
c
sin30
⎝ ⎠
___________________________________________________________________________________________________________________________________________________________________________________________________________________________________________________________________________________________
Section 7 Problems--------------- ------- -----------------------------------------------------------
In problems 1 to 10 use the law of sines to solve each triangle ABC. Round off angles
to the nearest hundredth of a degree and side lengths to four significant digits.
1. a = 32, β =
o
38 , γ =
o
21 .
2. a = 50, b = 30, α = 45 .
3. a = 40, b = 70, α = 30 .
4. b = 4.5, c = 9, γ =
o
60 .
5. a = 31, b = 33, α = 60 .
6. α = β = o 14 , c = 30.
o
7. α = 30 , a = 8, b = 5.
o
8. α = 30 , a = 5, b = 8.
o
9. β = 60 , a = 11, b = 12.
o
10. β = 60 , a = 12, b = 11.
o
o
o
52