Vol. 11 No 1 - Pi Mu Epsilon

26 ANATOLYSHTEKHMAN
Proof. From [7] Theorem 3.6 we know that the inequality AG(x, y) ~ L:1 (x, y)
2
2
holds for all x, y > 0. From our Definition 2, L~ (x, y) = L ( x~, y~) 3 , so we obtain
2
AG(x, y) ~ L ( x~, y~) 3 and AG(x, y)~ ~ L ( x~, y!). Now, let us substitute p = x ~
and q = y~, then AG (pt,qt) ~ = AGf(p,q) ~ L(p,q). By Theorem 8 AGt(x,y) <
L(x, y) holds for all t E (0, ~). 0
Therefore we can exclude any values oft between 0 and ~'since ACt and Lt are
strictly increasing functions oft E (0, 1 ).
For the rest of a problem i.e., where t E [~, 1) we have the following conjecture.
CONJECTURE 7. For every number t E [~, 1) there exists a constant m > 0 such
that if y > mx > 0 then AGt(x, y) < L(x, y).
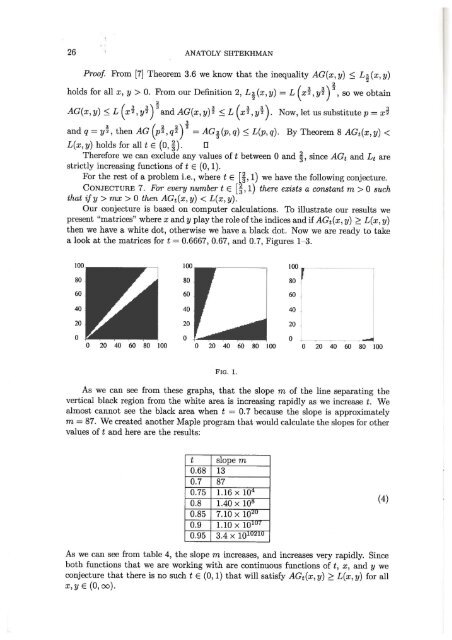
Our conjecture is based on computer calculations. To illustrate our results we
present "matrices" where x andy play the role of the indices and if AGt(x, y) 2: L(x, y)
then we have a white dot, otherwise we have a black dot. Now we are ready to take
a look at the matrices fort= 0.6667, 0.67, and 0.7, Figures 1- 3.
100 100
100 r
80 80 80 ~
ARITHMETIC-GEOMETRIC MEANS 27
Acknowledgement. I want to dedicate this paper and say special thanks to Dr.
Jakub Jasinski for all of his time, effort, help, and assistance during this research.
REFERENCES
[1] BECKENBACH, BELLMAN, E.F.R. "Inequalities", Springer-Verlag, Berlin-Heidelberg-New York,
1961
[2] BERRGREN, L., BORWEIN, J., AND BORWEIN, P., "II: a Source Book," Springer-Verlag Inc. New
York 1997.
[3] Cox, D.A., Gauss and the Arithmetic-Geometric Mean, Notices AMS, 32 (1985) 147-151.
[4] OLMSTED, J.M.H., "Advanced Calculus", Prentice-Hall, Inc. Englewood Cliffs, N.J., 1961
[5] SALAMIN, E., Computation of rr using Arithmetic-Geometric Mean, Mathematics of Computation,
30 (1976) 565-570.
[6] STEWART, JAMES, "Calculus", Brooks/Cole Publishing Company, 3-rd edition, 1995.
[7] VAMANAMURTHY, M.K., VUORINEN , M. , Inequalities for Means, Journal of Mathematical Analysis
and Applications, 183 (1994) 155-166.
Anatoly Shtekhman, 539 Adams Ave, Scranton PA 18510.
Anatoly Shtekhman is a native ofVinnitsa, Ukraine. In 1995 he moved to the U.S.
where, in 1996, he became a math major at the University of Scranton. This paper
is the result of a junior research project under the direction of Dr. Jakub Jasinski.
60 60 60 ~
40 40 40
20 20 20
0 0 0
0 20 40 60 80 100 0 20 40 60 80 100 0 20 40 60 80 100
FIG. 1.
As we can see from these graphs, that the slope m of the line separating the
vertical black region from the white area is increasing rapidly as we increase t. We
almost cannot see the black area when t = 0. 7 because the slope is approximately
m = 87. We created another Maple program that would calculate the slopes for other
values oft and here are the results:
t slope m
0.68 13
0.7 87
0.75 1.16 X 10 4
0.8 1.40 X lQM
0.85 7.10 X 10 20
0.9 1.10 X 10 107
0.95 3.4 X 1010210
As we can see from table 4, the slope m increases, and increases very rapidly. Since
both functions that we are working with are continuous functions oft, x, andy we
conjecture that there is no such t E (0, 1) that will satisfy AGt(x, y) 2: L(x, y) for all
x, y E (0, oo).
(4)
A Note on Infinite Harmony.
2:::-:-=00
'l
i=1
n 1
The divergence of the Harmonic Series may be considered a "classic" among the
basic results of calculus. There are many different proofs of this fact and it might be
a nice exercise for students to find their own proofs before looking up the standard
proof in the their Calculus Book. Here is a proof by ANDREW CUSUMANO of Great
Neck, New York:
We start with the observation that
1 1 2x 2
x - c + x + c = x 2 - c2 > ;;
from which we easily deduce that the sum of any string of 2k + 1 terms of the harmoni
series centered about 1/(2k + 1) is
The following 3(2k + 1) = 2(3k + 1) + 1 terms will be centered about the term
1 1
~----~~----~ = -------
(3k+2)+(3k+l) 3(2k+l)
and so will also sum up to a number greater than 1, and so on. It follows immediately
that the harmonic series diverges.
The asymptotics of these estimates could be investigated.