A MODIFIED BERNOULLI NUMBER D. Zagier The classical ... - Up To

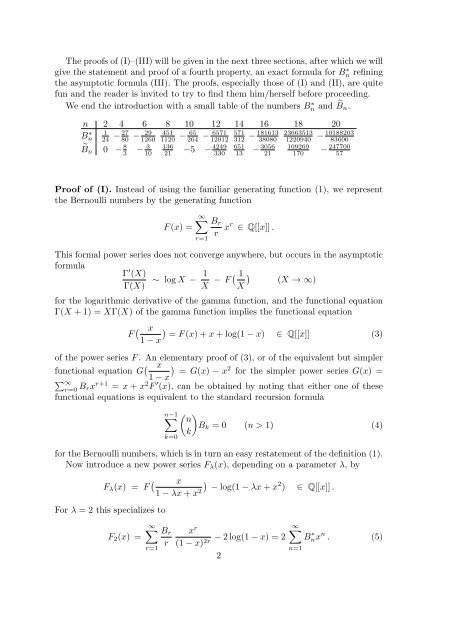
The proofs of (I)–(III) will be given in the next three sections, after which we willgive the statement and proof of a fourth property, an exact formula for B ∗ n refiningthe asymptotic formula (III). The proofs, especially those of (I) and (II), are quitefun and the reader is invited to try to find them him/herself before proceeding.We end the introduction with a small table of the numbers B ∗ n and ˜B n .n 2 4 6 8 10 12 14 16 18 20B ∗ n124 −27 80 − 291260˜B n 0 − 8 3− 3104511120 − 65264 − 65711201213621−5 − 4249330571312 −181613 3808065113− 305621236635131220940 −10188203 83600109269170− 24770057Proof of (I). Instead of using the familiar generating function (1), we representthe Bernoulli numbers by the generating functionF(x) =∞∑r=1B rr xr ∈ Q[[x]].This formal power series does not converge anywhere, but occurs in the asymptoticformulaΓ ′ (X)∼ logX − 1 Γ(X) X − F( 1 )(X → ∞)Xfor the logarithmic derivative of the gamma function, and the functional equationΓ(X +1) = XΓ(X) of the gamma function implies the functional equationF ( x )= F(x)+x+log(1−x) ∈ Q[[x]] (3)1−xof the power series F. An elementary proof of (3), or of the equivalent but simplerfunctional equation G ( x )= G(x) − x 2 for the simpler power series G(x) =∑ ∞1−xr=0 B rx r+1 = x + x 2 F ′ (x), can be obtained by noting that either one of thesefunctional equations is equivalent to the standard recursion formulan−1∑( nB k = 0 (n > 1) (4)k)k=0for the Bernoulli numbers, which is in turn an easy restatement of the definition (1).Now introduce a new power series F λ (x), depending on a parameter λ, byF λ (x) = F ( x )−log(1−λx+x 21−λx+x 2 ) ∈ Q[[x]].For λ = 2 this specializes toF 2 (x) =∞∑r=1B rrx r∞(1−x) 2r −2log(1−x) = 2 ∑2n=1B ∗ nx n . (5)