On the defining polynomials of maximal real cyclotomic extensions

More documents

Recommendations

Info

$- \ v - Real Academia de Ciencias Exactas, FÃƒÂsicas y Naturales$

M. Aranés and A. ArenasX −1 ) is obviously a monic polynomial in X with rational coefficients, vanishing when X = ζ and ofdegree p ν−1 (p − 1), we see thatWe writeX pν−1 (p−1)/2 Ψ(X + X −1 ) = Φ(X) (2)Ψ p ν (X) = Ψ(X) = a m X m + a m−1 X m−1 + · · · + a 1 X + a 0 (3)where, of course a m = 1, and m = 1 2 ϕ(pν ) = p ν−1 (p − 1)/2.This paper is devoted to the evaluation of the coefficients a m−1 , . . . , a 1 , a 0 .2 PreliminariesIn order to achieve our aim we first proceed to expand the relation (2) using the notation of (3):⎛⎛⎞∑m (Φ(X) = X m a i X + 1 ) i m∑i∑(= a i X m−i ⎝ i2m∑∑(XX j)2(i−j) ⎠ = ⎜i⎝ a iji=0i=0j=0t=0i−2j=t−m0≤j≤i≤m⎞)⎟⎠ Xt .This shows that a 0 , . . . , a m is a solution of the system of linear equations∑( ) ia i = b t , (4)ji−2j=t−m0≤j≤i≤mwhere by (1), for 0 ≤ t ≤ ϕ(p ν ) = 2m,⎧⎨1, if t = p ν−1 l, 0 ≤ l < pb t =⎩0, otherwise,For the sake of clarity we rewrite the preceding system of equations (4) more explicitly as follows:⎧k/2∑( )m − k + 2ja m−k+2j , for k = 2, 4, . . . , r.⎪⎨jj=0b 2m−k =(5)(k−1)/2∑( )m − k + 2j⎪⎩a m−k+2j , for k = 1, 3, . . . , s.jj=0where r = m, s = m − 1, if p ≡ 1 (mod 4) or p = 2; and r = m − 1, s = m in the case p ≡ 3 (mod 4).In order to get the explicit solution of (5) we are led to compute the following determinants of order k,for strictly positive integers r, k:( r+2(k−1)) ( r+2(k−2))100 0 · · · · · · 0( r+2(k−1)) ( r+2(k−2)) ( r+2(k−3))2100 · · · · · · 0. .... .. .A r (k) :=....... ..... 0( r+2(k−1)) ( r+2(k−2)) ( r+2(k−3)) ( r+2(k−4))( k−1k−2k−3k−4· · · · · · r ( 0)∣ r+2(k−1)) ( r+2(k−2)) ( r+2(k−3)) ( r+2(k−4))(kk−1k−2k−4· · · · · · r 1)∣184
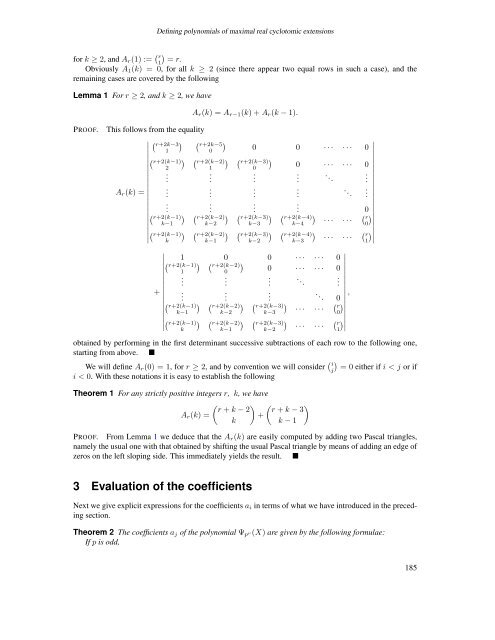
Defining polynomials of maximal real cyclotomic extensionsfor k ≥ 2, and A r (1) := ( r1)= r.Obviously A 1 (k) = 0, for all k ≥ 2 (since there appear two equal rows in such a case), and theremaining cases are covered by the followingLemma 1 For r ≥ 2, and k ≥ 2, we havePROOF.This follows from the equality( r+2k−31( r+2(k−1)2.A r (k) =..( r+2(k−1)k−1(∣ r+2(k−1)+∣kA r (k) = A r−1 (k) + A r (k − 1).) ( r+2k−50)) ( r+2(k−2)1.) ( r+2(k−2)k−2( r+2(k−1)( r+2(k−1)k−1( r+2(k−1)k) ( r+2(k−2)k−1..) ( r+2(k−3)0) ( r+2(k−3)k−3) ( r+2(k−3)k−20 0 · · · · · · 0)0 · · · · · · 0... .. ...... ... 0) ( r+2(k−4))( k−4· · · · · · r 0)) ( r+2(k−4))(k−3· · · · · · r 1)∣1 0 0 · · · · · · 0) ( r+2(k−2))100 · · · · · · 0. ... .. .. ..... 0,) ( r+2(k−2)) ( r+2(k−3))( k−2k−3· · · · · · r 0)) ( r+2(k−2)) ( r+2(k−3))(k−1k−2· · · · · · r ∣1)obtained by performing in the first determinant successive subtractions of each row to the following one,starting from above. We will define A r (0) = 1, for r ≥ 2, and by convention we will consider ( ij)= 0 either if i < j or ifi < 0. With these notations it is easy to establish the followingTheorem 1 For any strictly positive integers r, k, we have( ) ( )r + k − 2 r + k − 3A r (k) =+kk − 1PROOF. From Lemma 1 we deduce that the A r (k) are easily computed by adding two Pascal triangles,namely the usual one with that obtained by shifting the usual Pascal triangle by means of adding an edge ofzeros on the left sloping side. This immediately yields the result. 3 Evaluation of the coefficientsNext we give explicit expressions for the coefficients a i in terms of what we have introduced in the precedingsection.Theorem 2 The coefficients a j of the polynomial Ψ p ν (X) are given by the following formulae:If p is odd,185
Page 5 and 6: Defining polynomials of maximal rea
Page 7 and 8: Defining polynomials of maximal rea
Page 9: Defining polynomials of maximal rea

On the defining polynomials of maximal real cyclotomic extensions

You also want an ePaper? Increase the reach of your titles

Delete template?

Save as template?