lecture notes 13

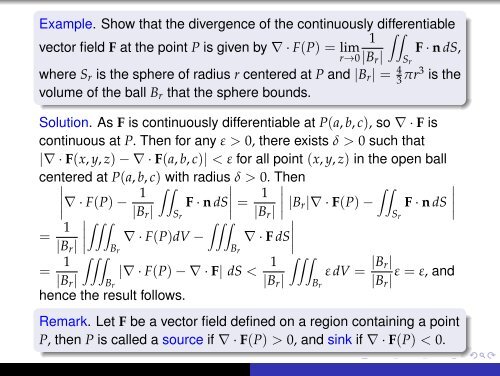
.Example. Show that the divergence of the continuously differentiable∫∫1vector field F at the point P is given by ∇ · F(P) = lim F · n dS,r→0 |B r | S rwhere S r is the sphere of radius r centered at P and |B r | =3 4 πr3 is the. volume of the ball B r that the sphere bounds.Solution. As F is continuously differentiable at P(a, b, c), so ∇ · F iscontinuous at P. Then for any ε > 0, there exists δ > 0 such that|∇ · F(x, y, z) − ∇ · F(a, b, c)| < ε for all point (x, y, z) in the open ballcentered at P(a, b, c) with radius δ > 0. Then∣ ∇ · F(P) − 1 ∫∫F · n dS|B r |∣ = 1∫∫S r |B r | ∣ |B r|∇ · F(P) − F · n dS∣S r= 1∫∫∫∫∫∫|B r | ∣ ∇ · F(P)dV − ∇ · F dS∣B r B r= 1 ∫∫∫|∇ · F(P) − ∇ · F| dS < 1 ∫∫∫ε dV = |B r|ε = ε, and|B r | B r |B r | B r |B r |hence the result follows..Remark. Let F be a vector field defined on a region containing a point. P, then P is called a source if ∇ · F(P) > 0, and sink if ∇ · F(P) < 0.. . . . . .