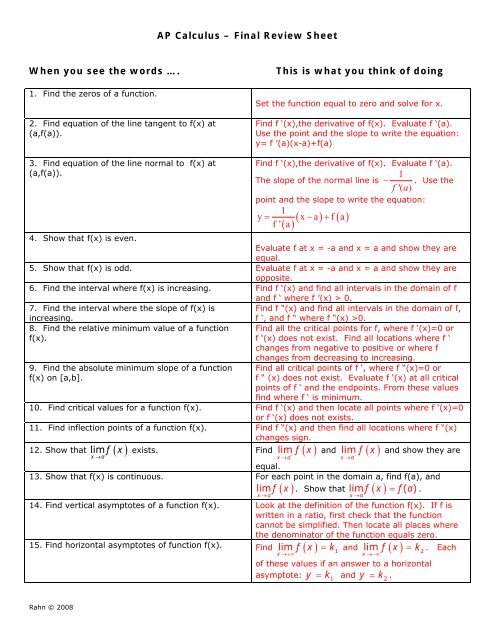
AP Calculus – Final Review Sheet When you see the words …. This ...

37. Find the area bounded by f(x), the x-axis,x=1 and x = 10 using 3 trapezoids, where Δx=3. Find f(1), f(4), f(7), and f(10). Use these for thebases in finding the area of three trapezoids withheights of 3:1 1 1(3)( f(1) + f(4)) + (3)( f(4) + f(7)) + (3)( f(7) + f (10))2 2 238. Approximate the area bounded by f(x), thex-axis, x=0 and x = 7 using left Reimannsums from information about f(x) given intabular data.x 0 1 5 7f(x) 1 13 16 539. Approximate the area bounded by f(x), thex-axis, x=0 and x = 7 using right Reimannsums from information about f(x) given intabular data.x 0 1 6 7f(x) -1 -13 -16 -541. Approximate the area bounded by f(x), thex-axis, x = 0, and x = 14 using two subintervalsand midpoint rectangles from information aboutf(x) given in tabular data.x 0 3 6 10 14f(x) 1 7 12 11 340. Approximate the area bounded by f(x), thex-axis, x = 0, and x = using threetrapezoids from information about f(x)given in tabular data.x 1 5 6 10f(x) 2 7 12 15Find the base, difference between x values, and height(at left hand end) of the three rectangles.1 1 + 4 13 + 2 16( ) ( ) ( ) ( ) ( ) ( )Find the base, difference between x values, and height(at right hand end) of the three rectangles.1 − 13 + 5 − 16 + 1 −5( ) ( ) ( ) ( ) ( ) ( )Find the intervals for the two rectangles: (0,6) and(6,14). The midpoints are 3 and 10. Find the heightof the rectangles: 7 and 11 respectively. Find the6 7 + 8 11area: ( ) ( ) ( ) ( )Find the height of the three trapezoids: 4, 1, and 4.Find the bases: 2 and 7, 7 and 12, and 12 and 15.Find the areas:1 1 1( 4)( 2 + 7) + ( 1)( 7 + 12) + ( 4)( 12 + 15)2 2 242. Given the graph of f ‘ (x) >0 between x=0and x = a and f(0) =8, find f(a). = +∫043. Solve the differential equation44. Describe the meaning of f ()dt tx∫adydx1+xy= .45. Given a base is bounded by x = a, x = b, f(x)and g(x), where f(x) < g(x) for all a

48. Find the minimum acceleration given v(t), thevelocity function.49. Approximate the value of f(1.1) by using thetangent line to f at x=1.50. Given the value of F(a) and the fact that theanti-derivative of f is F, find F(b). = +∫Find a(t) or the derivative of v(t) and a’(t). Find thecritical points for a(t) from a’(t). Find where a’(t) ischanging from negative to positive (a(t) changingfrom decreasing to increasing). These are locationsfor the local minimum accelerations.Write the tangent line at x=1.y = f '(1)( x − 1) + f (1) . Use x = 1.1 in this tangentline to find the approximate value of f(1.1).bF( b) F( a) f( x)dx51. Find the derivative of f(g(x)).( f ( g( x)) ) ' = f '( g( x)) ⋅ g '( x )a52. Given f ( x)dxb∫, find f ( x)ab∫ [ + k]dx53. Given a graph of f ‘(x), find where f(x) isincreasing.54. Given v(t), the velocity function, and s(0),the initial position, find the greatest distancefrom the origin of a particle on [0,b].55. Given a water tank with g gallons initially, isbeing filled at the rate of F(t) gallons/min andt ,emptied at the rate of E(t) gallons/min on [ ]1 ,t 2find the amount of water in the tank at mminutes where t1 < m < t2.56. Given a water tank with g gallons initially, isbeing filled at the rate of F(t) gallons/min andt ,emptied at the rate of E(t) gallons/min on [ ]1 ,t 2find the rate the water amount is changing at m.57. Given a water tank with g gallons initially, isbeing filled at the rate of F(t) gallons/min andt ,emptied at the rate of E(t) gallons/min on [ ]1 ,t 2find the time when the water is at a minimum.58. Given a chart of x and f(x) on selectedvalues between a and b, estimate f ‘(c) where c isbetween a and b.ab b b∫⎣⎡ ( ) ⎦⎤∫ ( ) ∫∫f x + k dx = f x dx + kdx =a a abaf( x) dx + k( b − a)From the graph of f ‘(x) find where the graph is belowthe x-axis. This means f ‘(x) is negative. Describethese intervals.Find when v(t) is zero. This means the function is atrest at these values. Write s(t).tst () s(0) v( xdx. ) Evaluate s(t) at each place= +∫0v(t) is zero. Pick out the greatest distance from theorigin.m∫ ( () − ())t1Ft Et dtF(t)-E(t)Differentiate the integral in question 55 with respectto t. This will give you a rate equation or the equationin question 56. Find the zeros for F(t)-E(t). Evaluatethe integral from question 55 at these zero’s and theendpoints. Pick out the minimum value.Use two sets of points (a, f(a)) and (b, f(b)) near c tof( b) − f( a)evaluate f '( c)≈b − aRahn © 2008

dy59. Given , draw a slope fielddx60. Given that f(x) < g(x). find the area betweencurves f(x) and g(x) between x = a and x = b on[a,b].Identify points on the graph. Name the coordinates ofthese points. Evaluate dy at these points. Draw adxshort line that represents the given slope at thatpoint. The slope field should model the slope of afamily of functions whose derivative is dydx .b∫a(( f x) − g( x))dx61. Given that f(x) > g(x). Find the volume of thesolid created if the region between curves f(x)and g(x) between x = a and x = b on [a,b]. isrevolved about the x-axis.f( a + h) − f( a)62. Find a limit in the form lim.h→0h63. Given information about f(x) for x in [a,b],show that there exists a c in the interval [a,b],f(b)-f(a)where f '(c) = . b-a64. Given f “ (x) and all critical values of x in(a,b) where f ’(x)=0, determine the location of allrelative extrema for f.65. Given f ‘(x) in graphical form on a domain(a,b), determine the location of all relativeextrema for f.66. Given that functions f and g are twicedifferentiable, find h ‘(x) if h(x) = f(x)g(x) +k.πb2 2∫ (( ( )) − ( ( )))af x g x dxDetermine the value of a and the function f.Differentiate f and evaluate at a.Check to see that f(x) is continuous on [a,b] anddifferentiable on (a,b). Then the Mean Value Theoremguarantees that there exists a c such thatf(b)-f(a)f '(c) = b-aCheck the concavity of f at each critical value wheref ‘(x) = 0. If f”(x)>0 you have found the location of aminimum. If f”(x)