Quantum Gravity

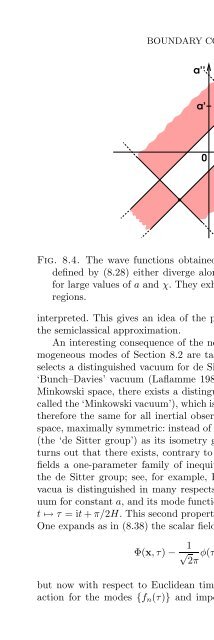
BOUNDARY CONDITIONS 267a’’0000000000000000000000000000000011111111111111111111111111111111000000000000000000000000000000001111111111111111111111111111111100000000000000000000000000000000111111111111111111111111111111110000000000000000000000000000000011111111111111111111111111111111000000000000000000000000000000001111111111111111111111111111111100000000000000000000000000000000111111111111111111111111111111110000000000000000000000000000000011111111111111111111111111111111a’ 000000000000000000000000000000001111111111111111111111111111111100000000000000000000000000000000111111111111111111111111111111110000000000000000000000000000000011111111111111111111111111111111000000000000000000000000000000001111111111111111111111111111111100000000000000000000000000000000111111111111111111111111111111110000000000000000000000000000000011111111111111111111111111111111000000000000000000000000000000001111111111111111111111111111111100000000000000000000000000000000111111111111111111111111111111110000000000000000000000000000000011111111111111111111111111111111000000000000000000000000000000001111111111111111111111111111111100000000000000000000000000000000000000000000000000000000000000000000000000000000000000000001111111111111111111111111111111111111111111111111111111111111111111111111111111111111111111000000000000000000000000000000001111111111111111111111111111111000000000000000000000000000000011111111111111111111111111111110000000000000000000000000000000111111111111111111111111111111100000000000000000000000000000001111111111111111111111111111111000000000000000000000000000000011111111111111111111111111111110000000000000000000000000000000111111111111111111111111111111100000000000000000000000000000001111111111111111111111111111111000000000000000000000000000000011111111111111111111111111111110000000000000000000000000000000111111111111111111111111111111100000000000000000000000000000001111111111111111111111111111111000000000000000000000000000000011111111111111111111111111111110000000000000000000000000000000111111111111111111111111111111100000000000000000000000000000001111111111111111111111111111111000000000000000000000000000000011111111111111111111111111111110000000000000000000000000000000111111111111111111111111111111100000000000000000000000000000001111111111111111111111111111111χ’ χ’’Fig. 8.4. The wave functions obtained from the path integral for the modeldefined by (8.28) either diverge along the light cones in minisuperspace orfor large values of a and χ. They exhibit oscillatory behaviour in the shadedregions.interpreted. This gives an idea of the problems that result if one goes beyondthe semiclassical approximation.An interesting consequence of the no-boundary proposal occurs if the inhomogeneousmodes of Section 8.2 are taken into account. In fact, this proposalselects a distinguished vacuum for de Sitter space—the so-called ‘Euclidean’ or‘Bunch–Davies’ vacuum (Laflamme 1987). What is the Euclidean vacuum? InMinkowski space, there exists a distinguished class of equivalent vacua (simplycalled the ‘Minkowski vacuum’), which is invariant under the Poincarégroupandtherefore the same for all inertial observers. De Sitter space is, like Minkowskispace, maximally symmetric: instead of the Poincaré group it possesses SO(4, 1)(the ‘de Sitter group’) as its isometry group, which also has 10 parameters. Itturns out that there exists, contrary to Minkowski space, for massive quantumfields a one-parameter family of inequivalent vacua which are invariant underthe de Sitter group; see, for example, Birrell and Davies (1982). One of thesevacua is distinguished in many respects: it corresponds to the Minkowski vacuumfor constant a, and its mode functions are regular on the Euclidean sectiont ↦→ τ =it + π/2H. This second property gives it the name ‘Euclidean vacuum’.One expands as in (8.38) the scalar field into its harmonics,Φ(x,τ) − √ 1 φ(τ) = ∑ f n (τ)Q n ,2π{n}but now with respect to Euclidean time τ. One then calculates the Euclideanaction for the modes {f n (τ)} and imposes the following regularity conditions